Chabot Mathematics § 6. 7 Rational Eqn Apps Bruce Mayer, PE Licensed Electrical & Mechanical Engineer BMayer@Chabot. College. edu Chabot College Mathematics 1 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Review § 6. 6 MTH 55 § Any QUESTIONS About • § 6. 6 → Rational Equations § Any QUESTIONS About Home. Work • § 6. 6 → HW-22 Chabot College Mathematics 2 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
§ 6. 7 Rational Equation Applications § Problems Involving Work § Problems Involving Motion § Problems Involving Proportions § Problems involving Average Cost Chabot College Mathematics 3 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Solve a Formula for a Variable § Formulas occur frequently as mathematical models. Many formulas contain rational expressions, and to solve such formulas for a specified letter, we proceed as when solving rational equations. Chabot College Mathematics 4 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Solve Rational Eqn for a Variable 1. Determine the DESIRED letter (many times formulas contain multiple variables) 2. Multiply on both sides to clear fractions or decimals, if that is needed. 3. Multiply if necessary to remove parentheses. 4. Get all terms with the letter to be solved for on one side of the equation and all other terms on the other side, using the addition principle. 5. Factor out the unknown. 6. Solve for the letter in question, using the multiplication principle. Chabot College Mathematics 5 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
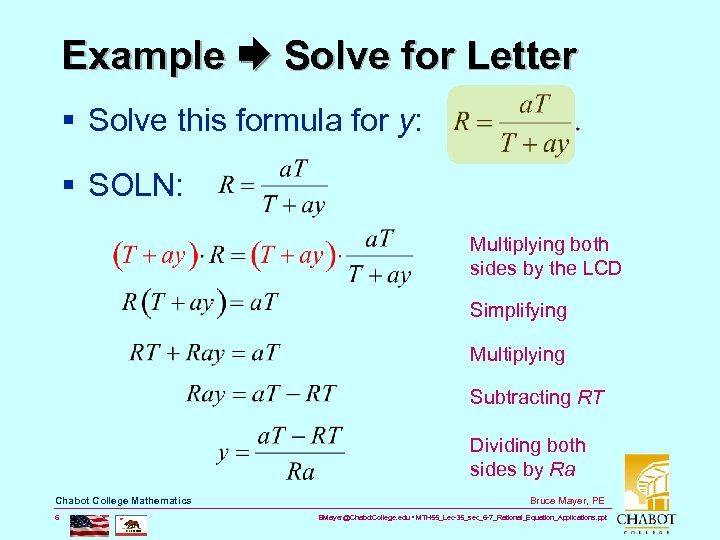
Example Solve for Letter § Solve this formula for y: § SOLN: Multiplying both sides by the LCD Simplifying Multiplying Subtracting RT Dividing both sides by Ra Chabot College Mathematics 6 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
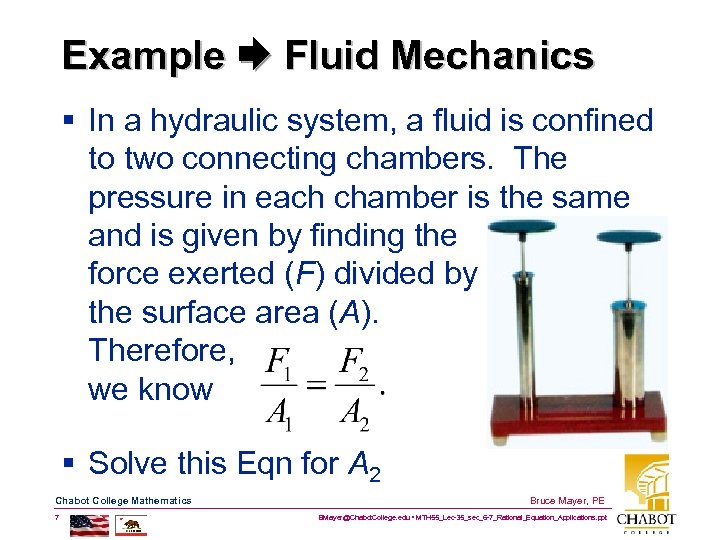
Example Fluid Mechanics § In a hydraulic system, a fluid is confined to two connecting chambers. The pressure in each chamber is the same and is given by finding the force exerted (F) divided by the surface area (A). Therefore, we know § Solve this Eqn for A 2 Chabot College Mathematics 7 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
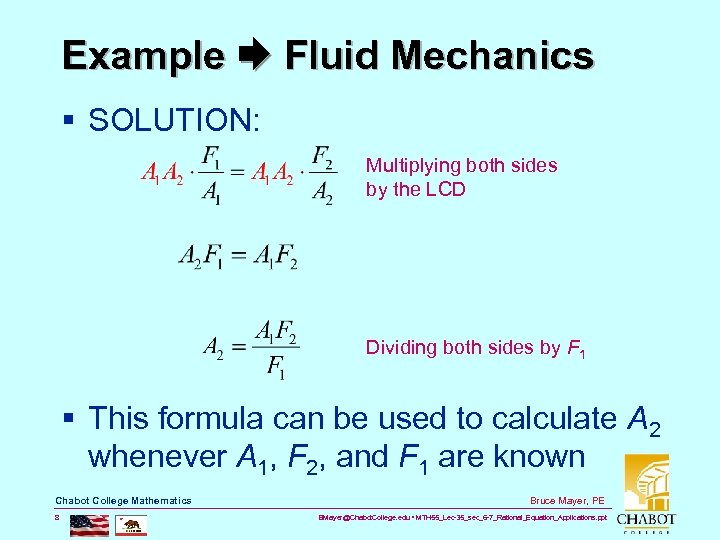
Example Fluid Mechanics § SOLUTION: Multiplying both sides by the LCD Dividing both sides by F 1 § This formula can be used to calculate A 2 whenever A 1, F 2, and F 1 are known Chabot College Mathematics 8 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Problems Involving Work § Rondae and Marrisa work during the summer painting houses. • Rondae can paint an average size house in 12 days • Marrisa requires 8 days to do the same painting job. § How long would it take them, working together, to paint an average size house? Chabot College Mathematics 9 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
House Painting cont. 1. Familiarize. We familiarize ourselves with the problem by exploring two common, but incorrect, approaches. a) One common, incorrect, approach is to add the two times. → 12 + 8 = 20 b) Another incorrect approach is to assume that Rondae and Marrisa each do half the painting. – Rondae does ½ in 12 days = 6 days – Marrisa does ½ in 8 days = days 4 6 days + 4 days = 10 days. Bruce Mayer, PE Chabot College – Mathematics 10 BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
House Painting cont. § A correct approach is to consider how much of the painting job is finished in ONE day; i. e. , consider the work RATE § It takes Rondae 12 days to finish painting a house, so his rate is 1/12 of the job per day. § It takes Marrisa 8 days to do the painting alone, so her rate is 1/8 of the job per day. § Working together, they can complete 1/8 + 1/12, or 5/24 of the job in one day. Chabot College Mathematics 11 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
![House Painting cont. § Note That given a TIME-Rate [Amount] = [Rate] • [Time.](https://present5.com/presentation/45203cb1b1420f574fee14ffe16dfe86/image-12.jpg "House Painting cont. § Note That given a TIME-Rate [Amount] = [Rate] • [Time.")
House Painting cont. § Note That given a TIME-Rate [Amount] = [Rate] • [Time. Quantity] § Form a table to help organize the info: Painter Rate of Work Time Amount Completed Rondae Marrisa 1/12 1/8 t t t/12 t/8 Chabot College Mathematics 12 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
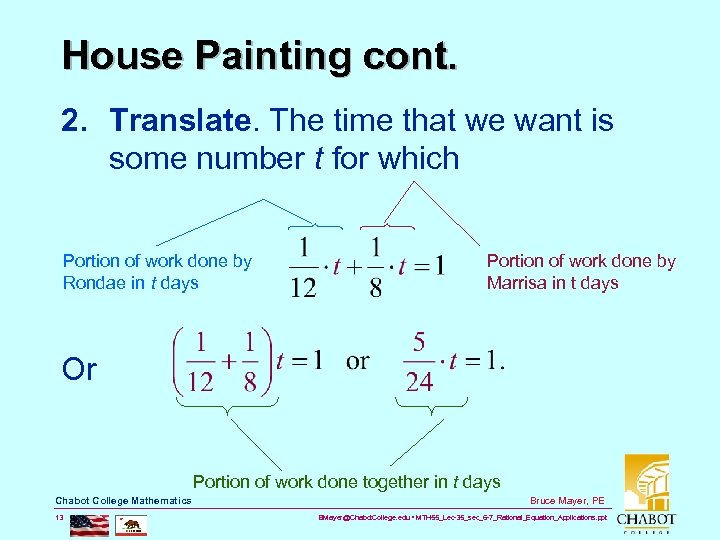
House Painting cont. 2. Translate. The time that we want is some number t for which Portion of work done by Rondae in t days Portion of work done by Marrisa in t days Or Portion of work done together in t days Chabot College Mathematics 13 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
House Painting cont. 3. Carry Out. We can choose any one of the above equations to solve: Chabot College Mathematics 14 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
House Painting cont. 4. Check. Test t = 24/5 days 5. State. Together, it will take Rondae & Marrisa 4 & 4/5 days to complete painting a house. Chabot College Mathematics 15 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
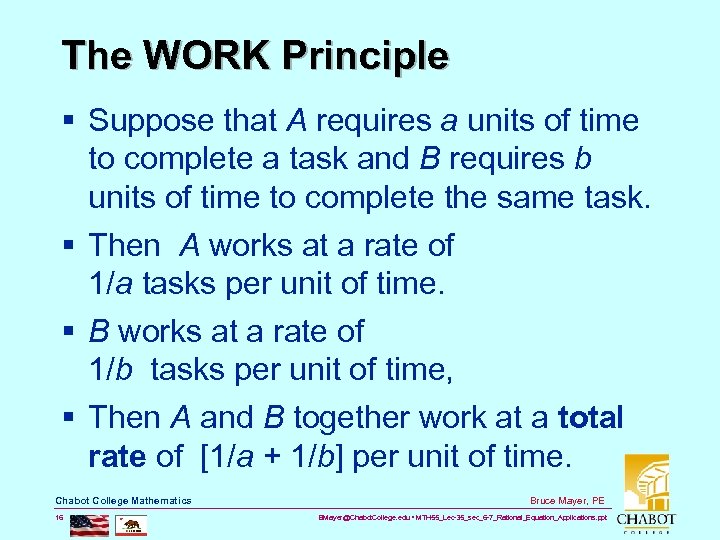
The WORK Principle § Suppose that A requires a units of time to complete a task and B requires b units of time to complete the same task. § Then A works at a rate of 1/a tasks per unit of time. § B works at a rate of 1/b tasks per unit of time, § Then A and B together work at a total rate of [1/a + 1/b] per unit of time. Chabot College Mathematics 16 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
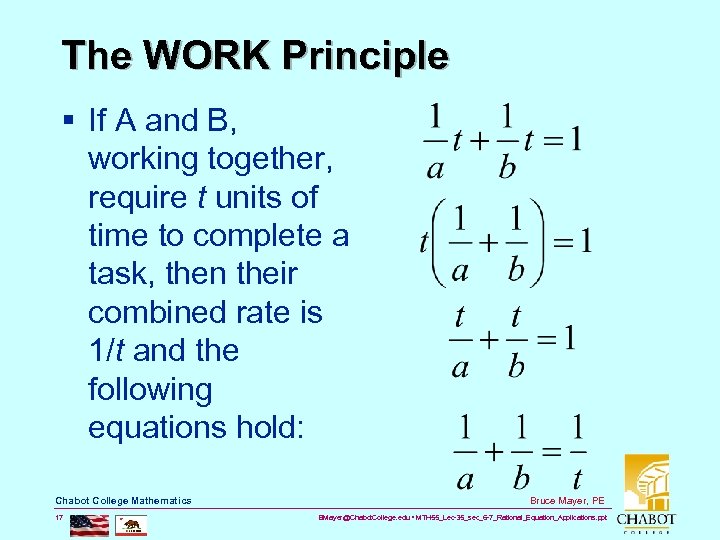
The WORK Principle § If A and B, working together, require t units of time to complete a task, then their combined rate is 1/t and the following equations hold: Chabot College Mathematics 17 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
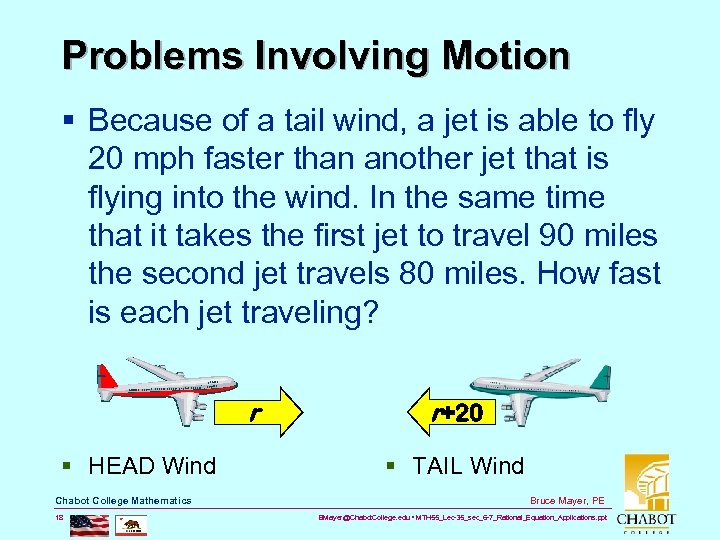
Problems Involving Motion § Because of a tail wind, a jet is able to fly 20 mph faster than another jet that is flying into the wind. In the same time that it takes the first jet to travel 90 miles the second jet travels 80 miles. How fast is each jet traveling? r § HEAD Wind Chabot College Mathematics 18 r+20 § TAIL Wind Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
HEADwind vs. TAILwind 1. Familiarize. We try a guess. If the fast jet is traveling 300 mph because of a tail wind the slow jet plane would be traveling 300− 20 or 280 mph. • • § At 300 mph the fast jet would have a 90 mile travel-time of 90/300, or 3/10 hr. At 280 mph, the other jet would have a travel-time of 80/280 = 2/7 hr. Now both planes spend the same amount of time traveling, So the guess is INcorrect. Chabot College Mathematics 19 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
![HEADwind vs. TAILwind 2. Translate. Fill in the blanks using [Time. Quantity]=[Distance]/[Rate] Air Craft](https://present5.com/presentation/45203cb1b1420f574fee14ffe16dfe86/image-20.jpg "HEADwind vs. TAILwind 2. Translate. Fill in the blanks using [Time. Quantity]=[Distance]/[Rate] Air Craft")
HEADwind vs. TAILwind 2. Translate. Fill in the blanks using [Time. Quantity]=[Distance]/[Rate] Air Craft Jet 1 Jet 2 Distance Speed or Rate Time (miles) (miles per hour) (hours) 80 90 r Chabot College Mathematics 20 r r + 20 r+20 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
![HEADwind vs. TAILwind § Set up a RATE Table [Distance]/[Rate] = [Time. Quantity] Air](https://present5.com/presentation/45203cb1b1420f574fee14ffe16dfe86/image-21.jpg "HEADwind vs. TAILwind § Set up a RATE Table [Distance]/[Rate] = [Time. Quantity] Air")
HEADwind vs. TAILwind § Set up a RATE Table [Distance]/[Rate] = [Time. Quantity] Air Distance Speed Craft (miles) (miles per hour) Jet 1 Jet 2 80 90 r r + 20 Time (hours) 80/r 90/(r + 20) The Times MUST be the SAME Chabot College Mathematics 21 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
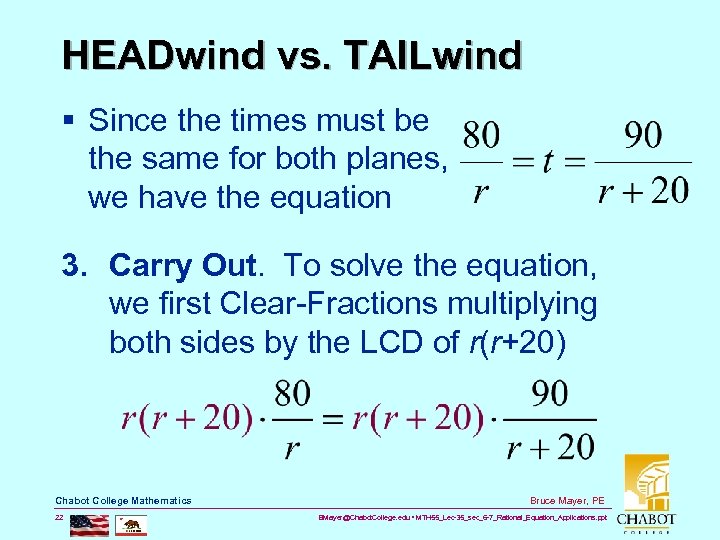
HEADwind vs. TAILwind § Since the times must be the same for both planes, we have the equation 3. Carry Out. To solve the equation, we first Clear-Fractions multiplying both sides by the LCD of r(r+20) Chabot College Mathematics 22 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
HEADwind vs. TAILwind § Complete the “Carry Out” Simplified by Clearing Fractions Using the distributive law Subtracting 80 r from both sides Dividing both sides by 10 § Now we have a possible solution. The speed of the slow jet is 160 mph and the speed of the fast jet is 180 mph Chabot College Mathematics 23 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
HEADwind vs. TAILwind 4. Check. Read the problem to confirm that we were able to find the speeds. At 160 mph the jet would cover 80 miles in ½ hour and at 180 mph the other jet would cover 90 miles in ½ hour. Since the times are the same, the speeds Chk 5. State. One jet is traveling at 160 mph and the second jet is traveling at 180 mph Chabot College Mathematics 24 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
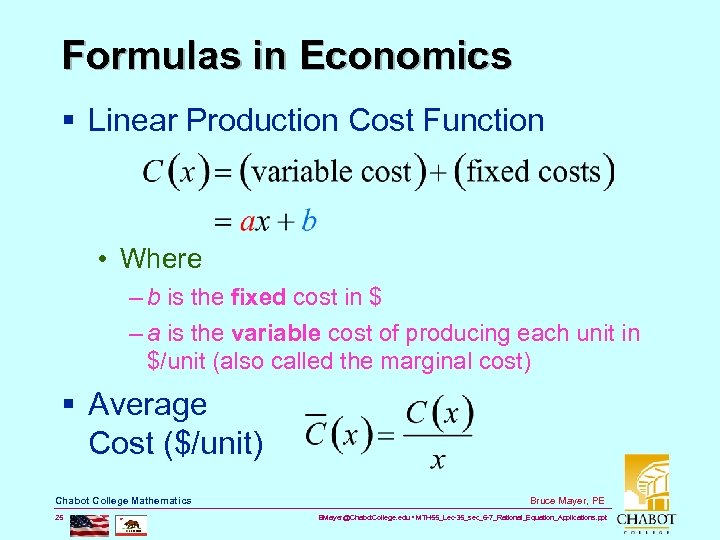
Formulas in Economics § Linear Production Cost Function • Where – b is the fixed cost in $ – a is the variable cost of producing each unit in $/unit (also called the marginal cost) § Average Cost ($/unit) Chabot College Mathematics 25 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Formulas in Economics § Price-Demand Function: Suppose x units can be sold (demanded) at a price of p dollars per units. • Where – m & n are SLOPE Constants in $/unit & unit/$ – d & k are INTERCEPT Constants in $ & units Chabot College Mathematics 26 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
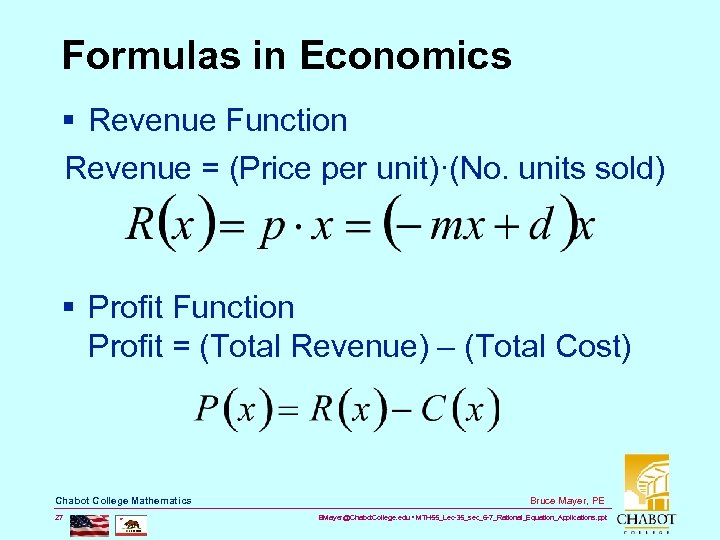
Formulas in Economics § Revenue Function Revenue = (Price per unit)·(No. units sold) § Profit Function Profit = (Total Revenue) – (Total Cost) Chabot College Mathematics 27 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Example Average Cost § Metro Entertainment Co. spent $100, 000 in production costs for its off-Broadway play Pride & Prejudice. Once running, each performance costs $1000 a) Write the Cost Function for conducting z performances b) Write the Average Cost Function for the z performances c) How many performances, n, result in an average cost of $1400 per show Chabot College Mathematics 28 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
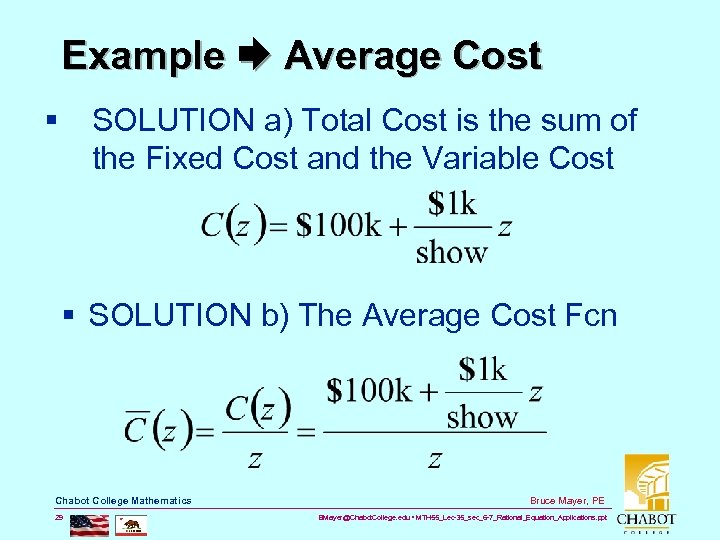
Example Average Cost § SOLUTION a) Total Cost is the sum of the Fixed Cost and the Variable Cost § SOLUTION b) The Average Cost Fcn Chabot College Mathematics 29 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
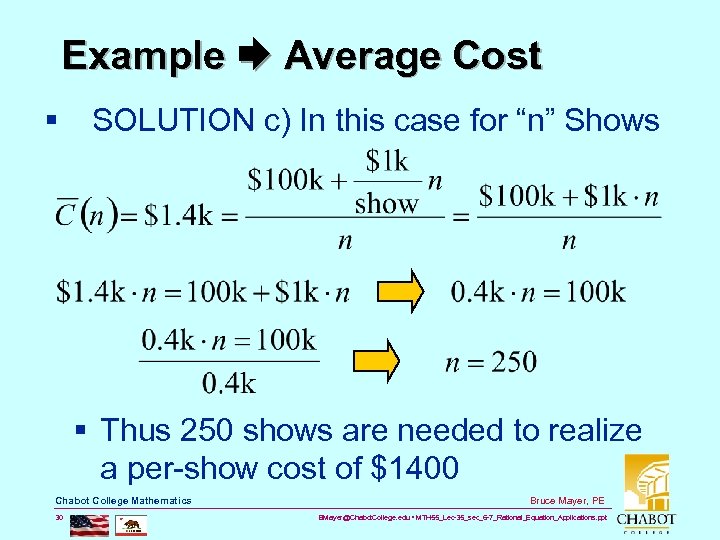
Example Average Cost § SOLUTION c) In this case for “n” Shows § Thus 250 shows are needed to realize a per-show cost of $1400 Chabot College Mathematics 30 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
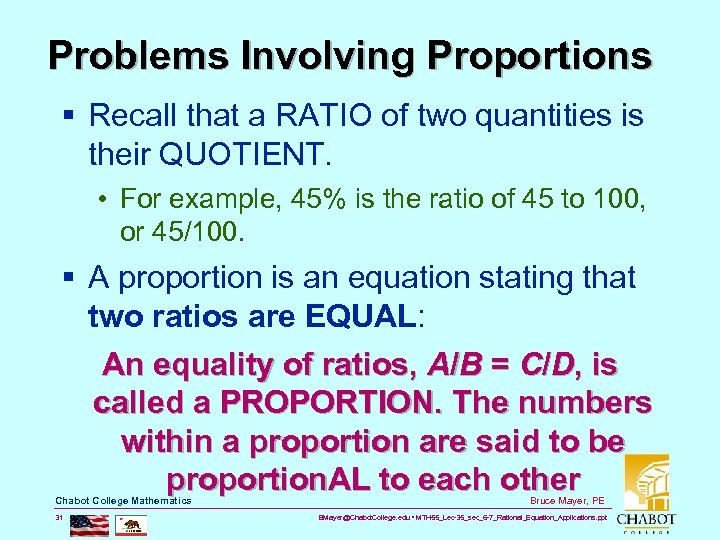
Problems Involving Proportions § Recall that a RATIO of two quantities is their QUOTIENT. • For example, 45% is the ratio of 45 to 100, or 45/100. § A proportion is an equation stating that two ratios are EQUAL: An equality of ratios, A/B = C/D, is called a PROPORTION. The numbers within a proportion are said to be proportion. AL to each other Chabot College Mathematics 31 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
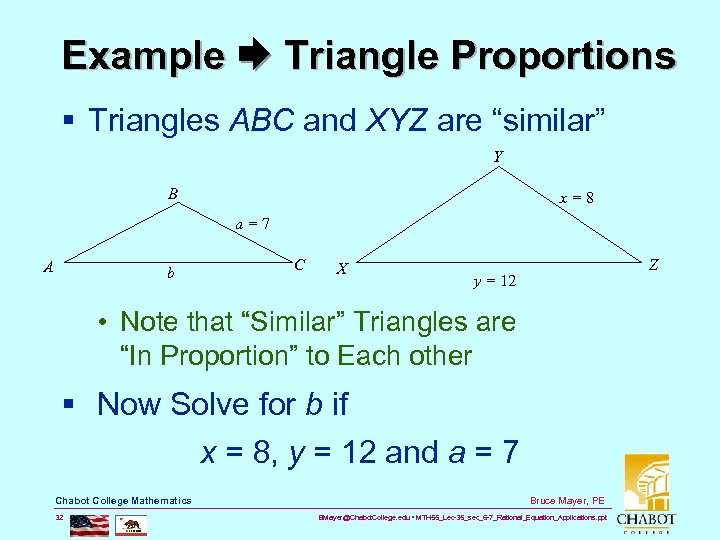
Example Triangle Proportions § Triangles ABC and XYZ are “similar” Y B x=8 a=7 A b C X Z y = 12 • Note that “Similar” Triangles are “In Proportion” to Each other § Now Solve for b if x = 8, y = 12 and a = 7 Chabot College Mathematics 32 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
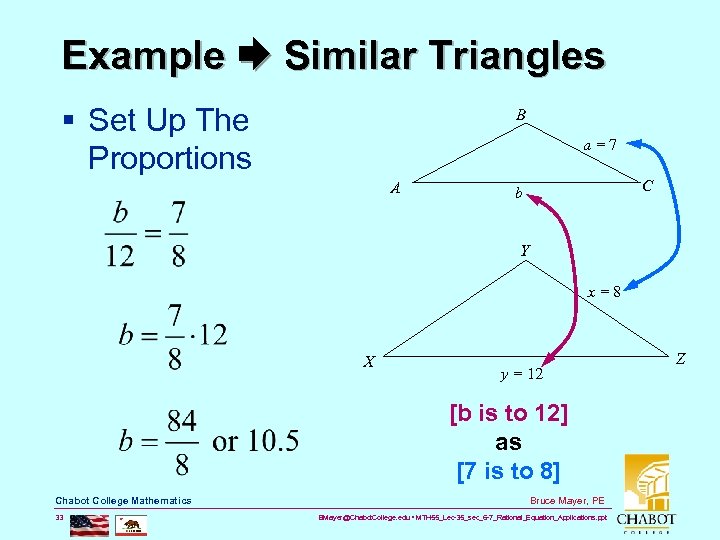
Example Similar Triangles § Set Up The Proportions B a=7 A C b Y x=8 X y = 12 [b is to 12] as [7 is to 8] Chabot College Mathematics 33 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt Z
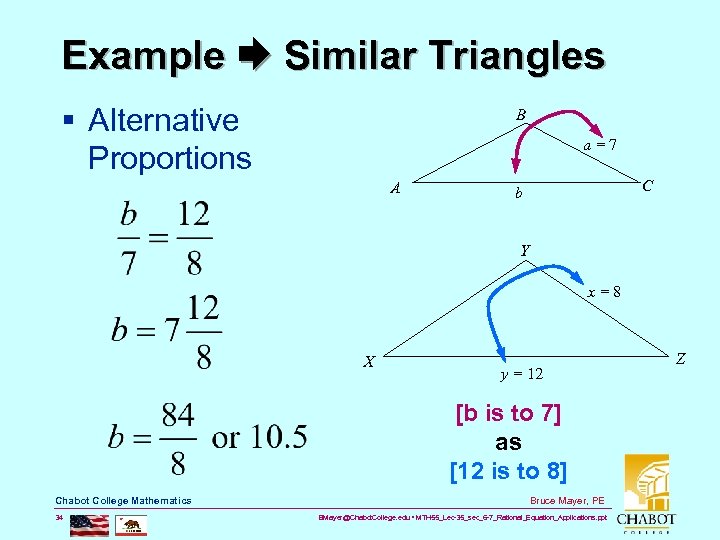
Example Similar Triangles § Alternative Proportions B a=7 A C b Y x=8 X y = 12 [b is to 7] as [12 is to 8] Chabot College Mathematics 34 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt Z
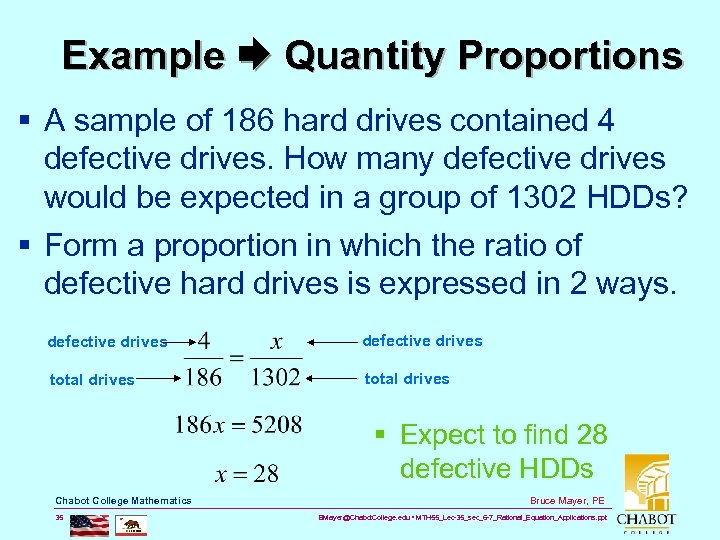
Example Quantity Proportions § A sample of 186 hard drives contained 4 defective drives. How many defective drives would be expected in a group of 1302 HDDs? § Form a proportion in which the ratio of defective hard drives is expressed in 2 ways. defective drives total drives § Expect to find 28 defective HDDs Chabot College Mathematics 35 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Whale Proportionality § To determine the number of humpback whales in a pod, a marine biologist, using tail markings, identifies 35 members of the pod. § Several weeks later, 50 whales from the SAME pod are randomly sighted. Of the 50 sighted, 18 are from the 35 originally identified. Estimate the number of whales in the pod. Chabot College Mathematics 36 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Tagged Whale Proportions 1. Familarize. We need to reread the problem to look for numbers that could be used to approximate a percentage of the pod sighted. § Since 18 of the 35 whales that were later sighted were among those originally identified, the ratio 18/50 estimates the percentage of the pod originally identified. Chabot College Mathematics 37 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
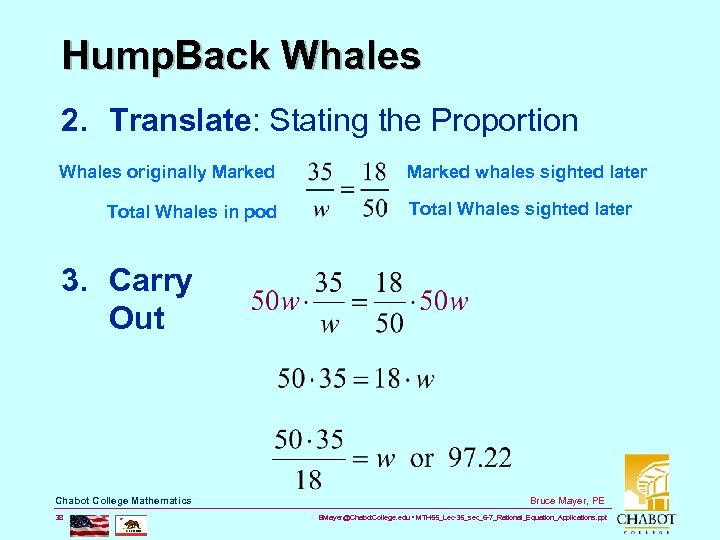
Hump. Back Whales 2. Translate: Stating the Proportion Whales originally Marked Total Whales in pod Marked whales sighted later Total Whales sighted later 3. Carry Out Chabot College Mathematics 38 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
More On Whales 4. Check. The check is left to the student. 5. State. There about 97 whales in the Pod Chabot College Mathematics 39 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
![One More Whale § Another way to summarize [35 is to w] the RANDOM-Tagging](https://present5.com/presentation/45203cb1b1420f574fee14ffe16dfe86/image-40.jpg "One More Whale § Another way to summarize [35 is to w] the RANDOM-Tagging")
One More Whale § Another way to summarize [35 is to w] the RANDOM-Tagging and as RANDOM-Sighting Relation: [18 is to 50] § Thus the Proportionality: § Solve for w Chabot College Mathematics 40 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Example Vespa Scooters § Juan’s new scooter goes 4 mph faster than Josh does on his scooter. In the same time that it takes Juan to travel 54 miles, Josh travels 48 miles. § Find the speed of each scooter. Chabot College Mathematics 41 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Example Vespa Scooters § Familiarize. Let’s guess that Juan is going 20 mph. Josh would then be traveling 20 – 4, or 16 mph. § At 16 mph, he would travel 48 miles in 3 hr. Going 20 mph, Juan would cover 54 mi in 54/20 = 2. 7 hr. Since 3 2. 7, our guess was wrong, but we can see that if r = the rate, in miles per hour, of Juan’s scooter, then the rate of Josh’s scooter = r – 4. Chabot College Mathematics 42 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
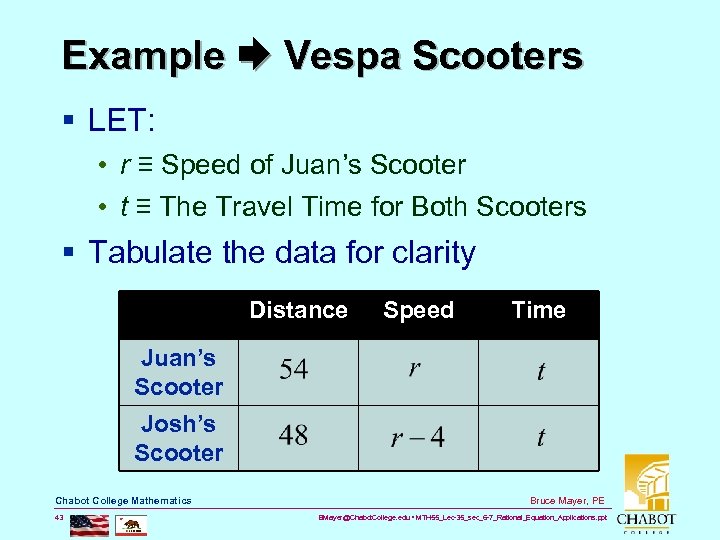
Example Vespa Scooters § LET: • r ≡ Speed of Juan’s Scooter • t ≡ The Travel Time for Both Scooters § Tabulate the data for clarity Distance Speed Time Juan’s Scooter Josh’s Scooter Chabot College Mathematics 43 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
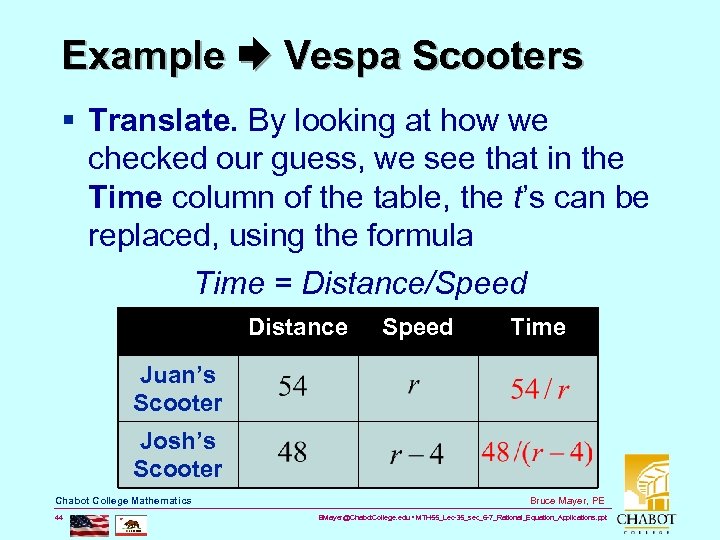
Example Vespa Scooters § Translate. By looking at how we checked our guess, we see that in the Time column of the table, the t’s can be replaced, using the formula Time = Distance/Speed Distance Speed Time Juan’s Scooter Josh’s Scooter Chabot College Mathematics 44 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
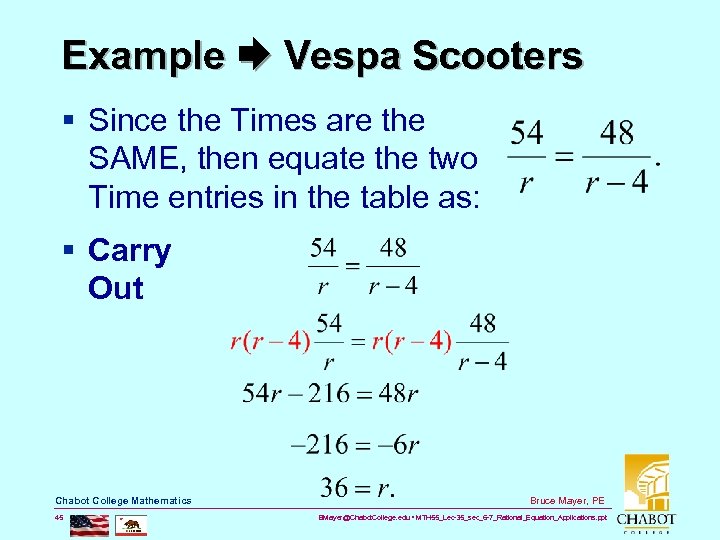
Example Vespa Scooters § Since the Times are the SAME, then equate the two Time entries in the table as: § Carry Out Chabot College Mathematics 45 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Example Vespa Scooters § Check: If our answer checks, Juan’s scooter is going 36 mph and Josh’s scooter is going 36 − 4 = 32 mph. Traveling 54 miles at 36 mph, Juan is riding for 54/36 or 1. 5 hours. Traveling 48 miles at 32 mph, Josh is riding for 48/32 or 1. 5 hours. The answer checks since the two times are the same. § State: Juan’s speed is 36 mph, and Josh’s speed is 32 mph Chabot College Mathematics 46 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
White. Board Work § Problems From § 6. 7 Exercise Set • 16 (ppt), 34, 44 § Mass Flow Rate for a Diverging Nozzle Chabot College Mathematics 47 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
P 6. 7 -16 § Given Avg Cost Function Graph: § Find Production Quatity for Avg Cost of $425/Chair § SOLUTION: Cast Right & Down 20 k § ANS → 20 k Chairs/mon Chabot College Mathematics 48 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
All Done for Today Human Proportions: Head. Length Base. Line Chabot College Mathematics 49 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
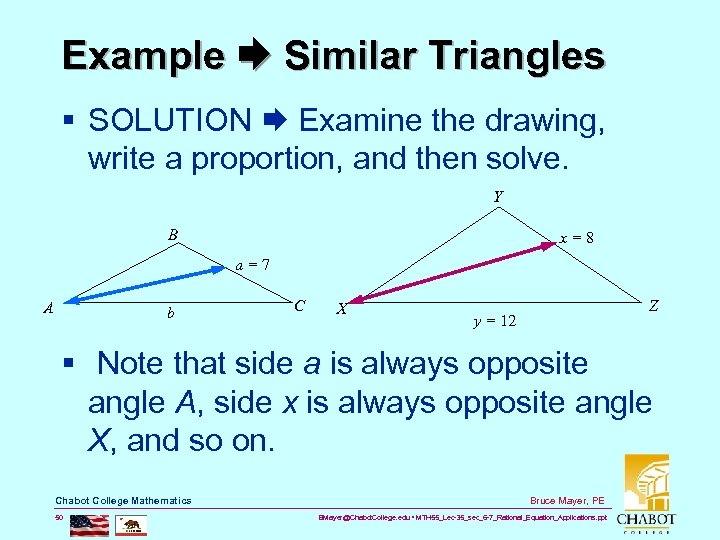
Example Similar Triangles § SOLUTION Examine the drawing, write a proportion, and then solve. Y B x=8 a=7 A b C X Z y = 12 § Note that side a is always opposite angle A, side x is always opposite angle X, and so on. Chabot College Mathematics 50 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Chabot Mathematics Appendix Bruce Mayer, PE Licensed Electrical & Mechanical Engineer BMayer@Chabot. College. edu – Chabot College Mathematics 51 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
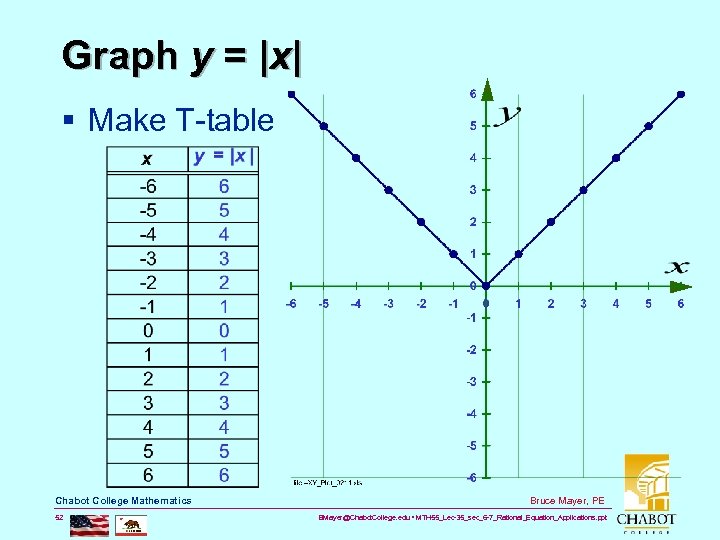
Graph y = |x| § Make T-table Chabot College Mathematics 52 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Chabot College Mathematics 53 Bruce Mayer, PE BMayer@Chabot. College. edu • MTH 55_Lec-35_sec_6 -7_Rational_Equation_Applications. ppt
Скачать презентацию Chabot Mathematics 6 7 Rational Eqn Apps
45203cb1b1420f574fee14ffe16dfe86.ppt