Ch 3 Derivatives 3 3 Rules

Ch. 3 – Derivatives 3. 3 – Rules for Differentiation

• Let’s learn some shortcuts for finding derivatives! • Power Rule: If , then – Ex: If g(x)=x 5, then g’(x)=5 x 4. – Ex: If h(x)=x-2, then h’(x)=-2 x-3. • Constant Multiple Rule: – Ex: If f(x)=3 x 2, then • Sum and Difference Rule: – Ex: If f(x)= x 2+x , then

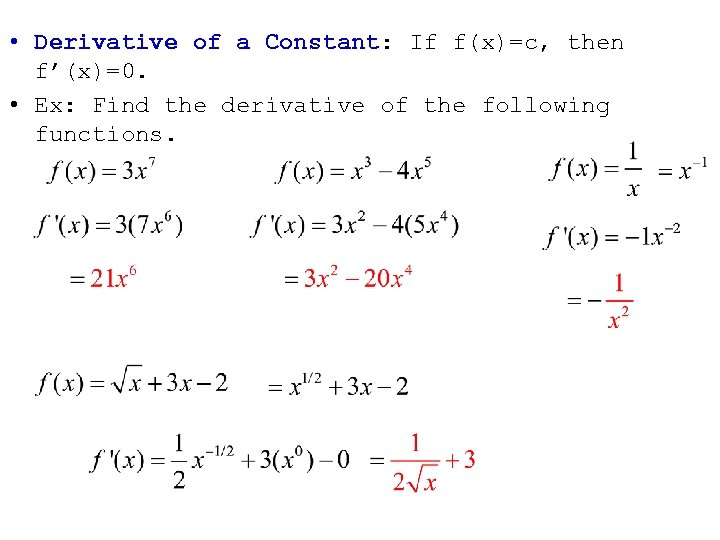• Derivative of a Constant: If f(x)=c, then f’(x)=0. • Ex: Find the derivative of the following functions.

• To find a second derivative, find the derivative of the derivative! • Ex: Find the second derivatives of the following functions.

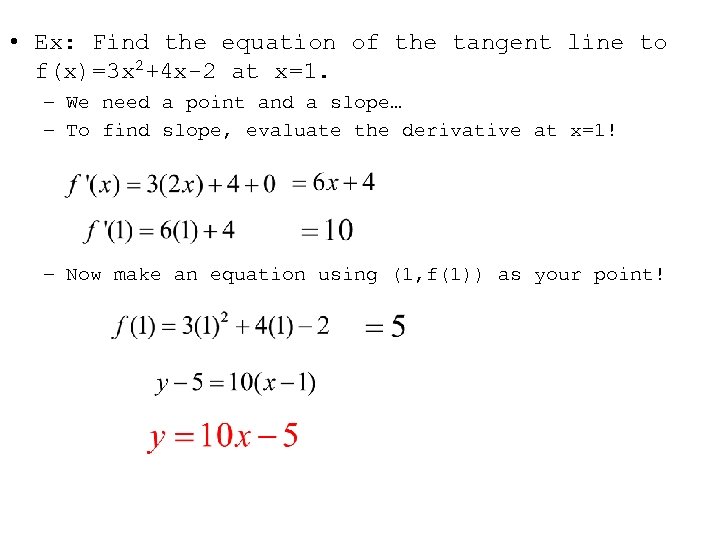• Ex: Find the equation of the tangent line to f(x)=3 x 2+4 x-2 at x=1. – We need a point and a slope… – To find slope, evaluate the derivative at x=1! – Now make an equation using (1, f(1)) as your point!

• Ex: Find the x-values at which the tangent line to the curve of is horizontal. – Horizontal tangent line means slope=0 – Slope=0 means derivative=0, so find the zeros of the derivative! – So

Product Rule: • When two functions are multiplied together, we must use product rule to find the total derivative. • Ex: Find the derivative of f(x)=(3 x 2 -x)(4–x 3). – Separate the problem into two products, u=3 x 2 -x and v=4 -x 3. . . – Would you get the same answer if you multiplied out f(x) at the start, then differentiated? YES!

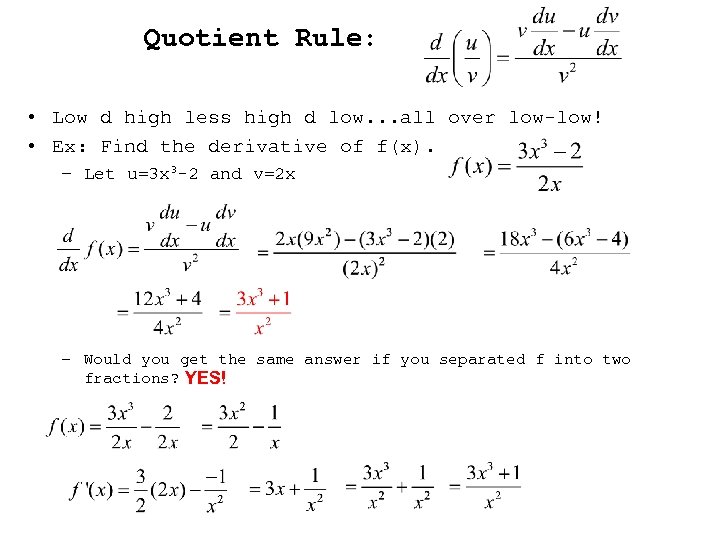Quotient Rule: • Low d high less high d low. . . all over low-low! • Ex: Find the derivative of f(x). – Let u=3 x 3 -2 and v=2 x – Would you get the same answer if you separated f into two fractions? YES!

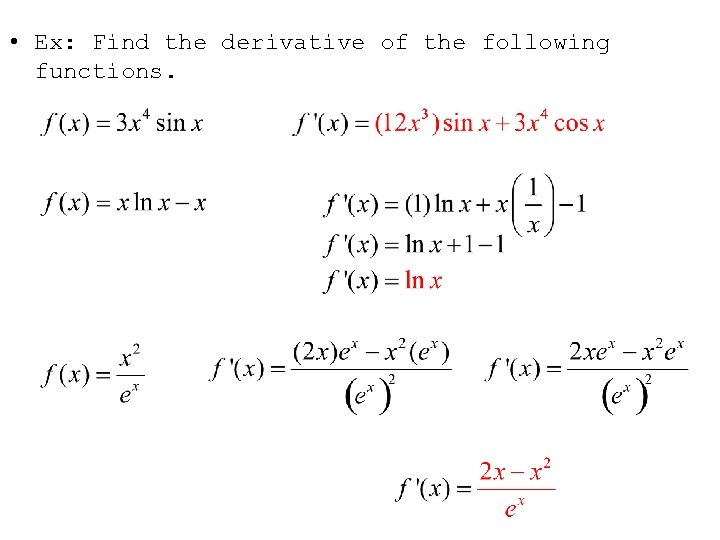• Ex: Find the derivative of the following functions.

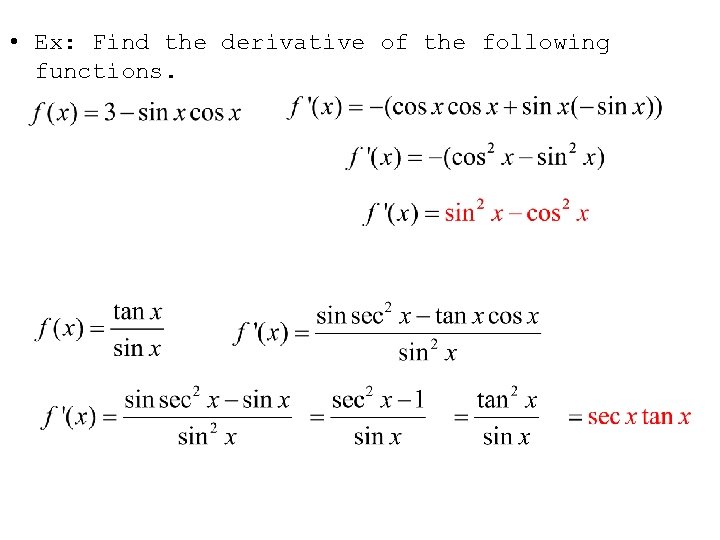• Ex: Find the derivative of the following functions.

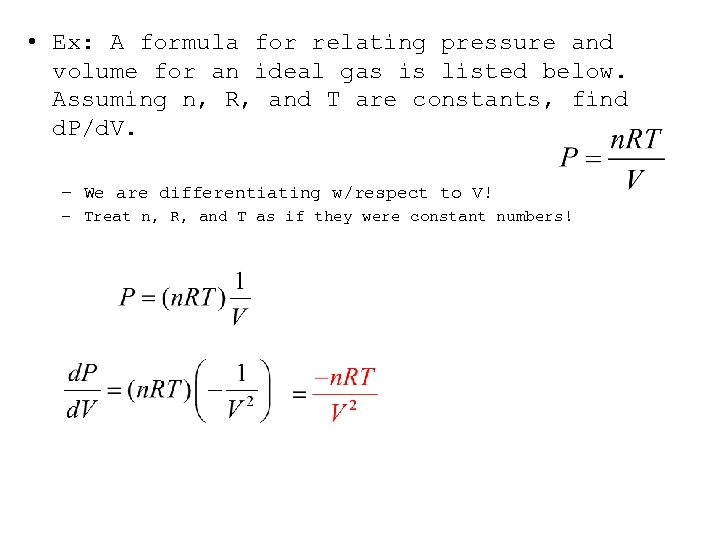• Ex: A formula for relating pressure and volume for an ideal gas is listed below. Assuming n, R, and T are constants, find d. P/d. V. – We are differentiating w/respect to V! – Treat n, R, and T as if they were constant numbers!