Bohr Model of the Atom Bohr s Atomic Model

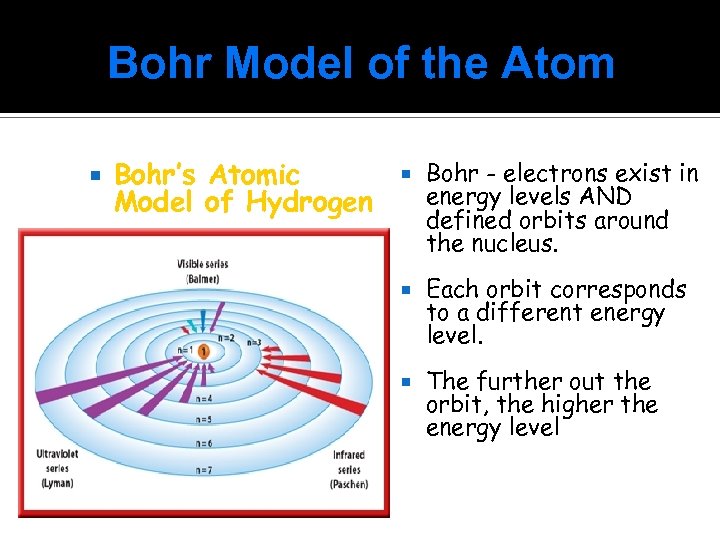
Bohr Model of the Atom Bohr’s Atomic Model of Hydrogen Bohr - electrons exist in energy levels AND defined orbits around the nucleus. Each orbit corresponds to a different energy level. The further out the orbit, the higher the energy level

Other Scientists Contributions De Broglie Heisenburg Modeled electrons as waves Heisenberg Uncertainty Principle: states one cannot know the position and energy of an electron Electrons exist in orbital’s of probability Orbital - the area in space around the nucleus where there is a 90% probability of finding an electron

Other Scientists Contributions Schrödinger Wave Equation - mathematical solution of an electron’s energy in an atom quantum mechanical model of the atom – current model of the atom treating electrons as waves.

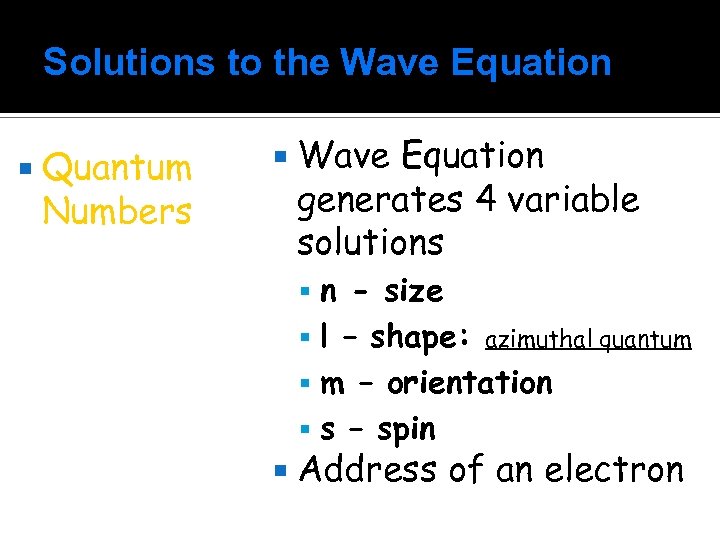
Solutions to the Wave Equation Quantum Numbers Wave Equation generates 4 variable solutions n - size l – shape: azimuthal quantum m – orientation s – spin Address of an electron

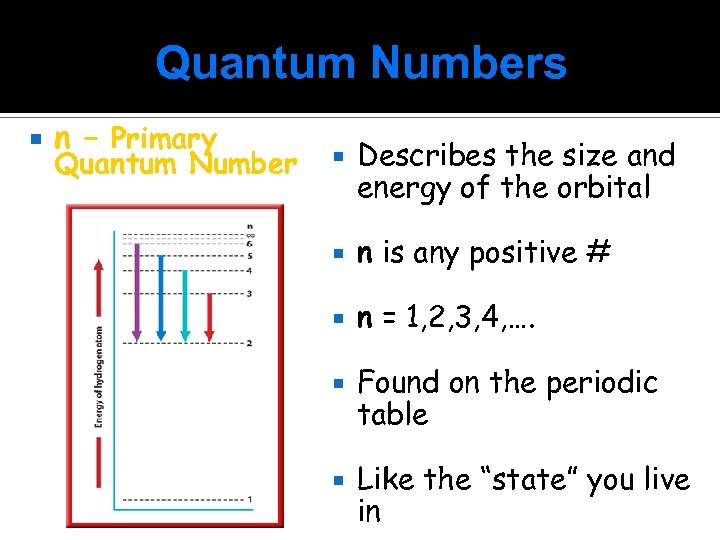
Quantum Numbers n – Primary Quantum Number Describes the size and energy of the orbital n is any positive # n = 1, 2, 3, 4, …. Found on the periodic table Like the “state” you live in

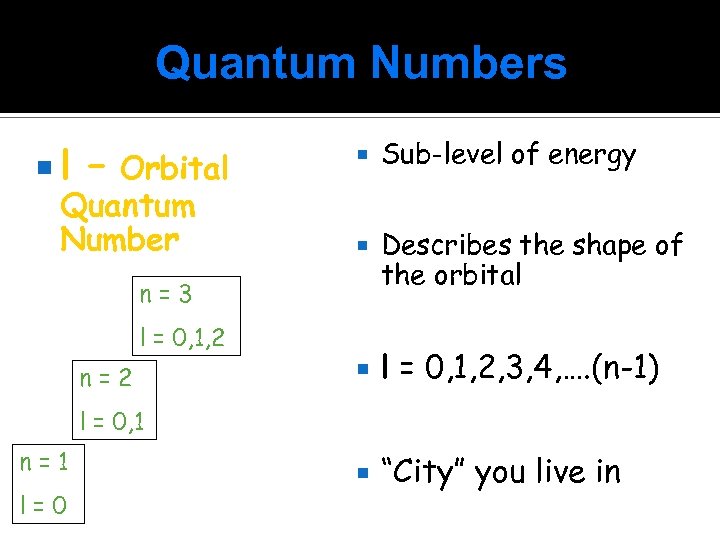
Quantum Numbers l – Orbital Quantum Number Sub-level of energy Describes the shape of the orbital l = 0, 1, 2, 3, 4, …. (n-1) “City” you live in n=3 l = 0, 1, 2 n=2 l = 0, 1 n=1 l=0

Quantum Numbers l – Orbital Quantum Number # level = # sublevels 1 st level – 1 sublevel 2 nd level – 2 sublevels 4 th level = 4 sublevels

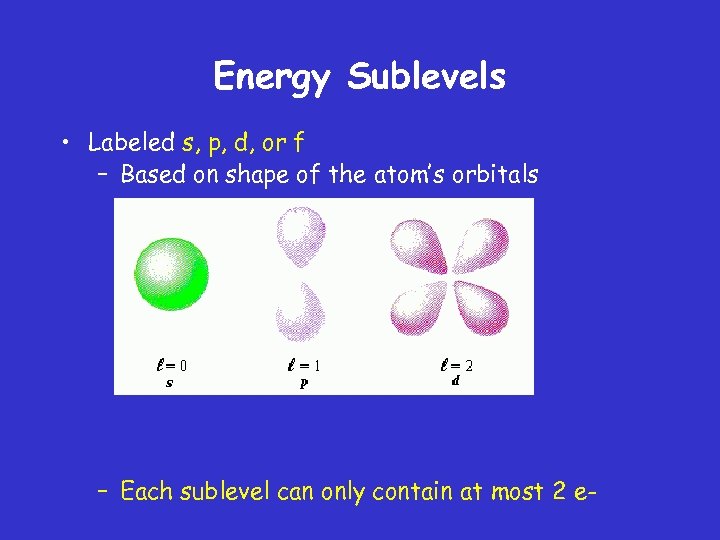
Energy Sublevels • Labeled s, p, d, or f – Based on shape of the atom’s orbitals – Each sublevel can only contain at most 2 e-

Quantum Numbers m – Magnetic Quantum Number Describes the orientation of the orbital in space Also denotes how many orbital's are in each sublevel For each sublevel there are 2 l +1 orbital's m = 0, ± 1, ± 2, ± 3, ±l “Street” you live on

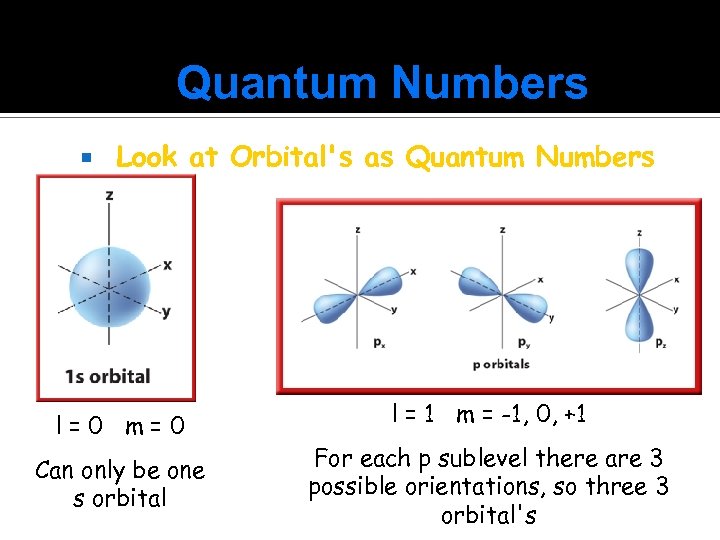
Quantum Numbers Look at Orbital's as Quantum Numbers l=0 m=0 Can only be one s orbital l = 1 m = -1, 0, +1 For each p sublevel there are 3 possible orientations, so three 3 orbital's

Assigning the Numbers v The three quantum numbers (n, l, and m) are integers. v The principal quantum number (n) cannot be zero. v n must be 1, 2, 3, etc. v The angular quantum number (l ) can be any integer between 0 and n - 1. v For n = 3, l can be either 0, 1, or 2. v The magnetic quantum number (ml) can be any integer between -l and +l. v For l = 2, m can be either -2, -1, 0, +1, +2.

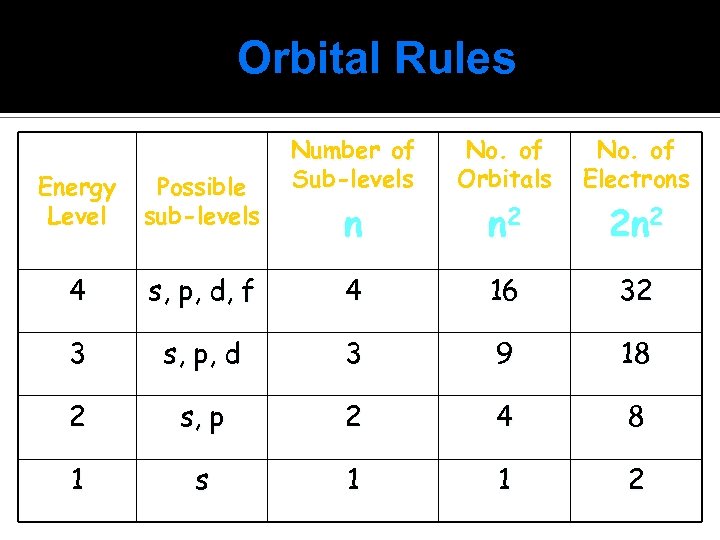
Orbital Rules Number of Sub-levels No. of Orbitals No. of Electrons n n 2 2 n 2 Energy Level Possible sub-levels 4 s, p, d, f 4 16 32 3 s, p, d 3 9 18 2 s, p 2 4 8 1 s 1 1 2

Hog Hilton Time Read the scenario Complete the questions Completed packet due tomorrow HW: Finish Packet

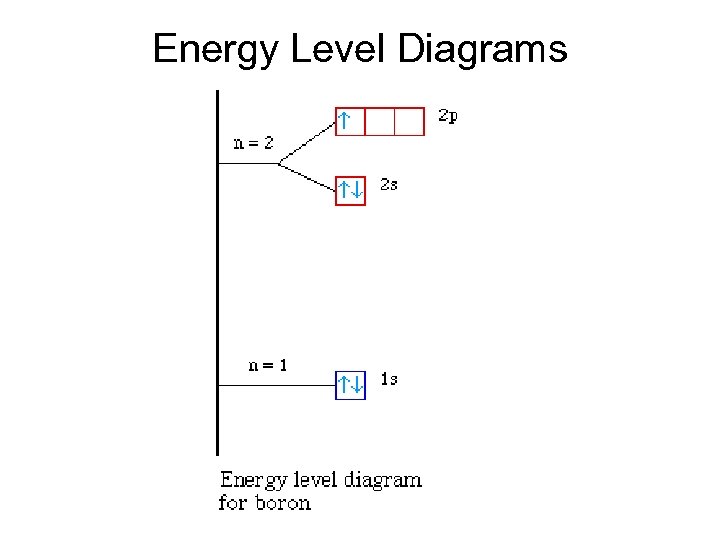
Energy Level Diagrams

Aufbau Principle • Electrons occupy the lowest energy level orbital available.

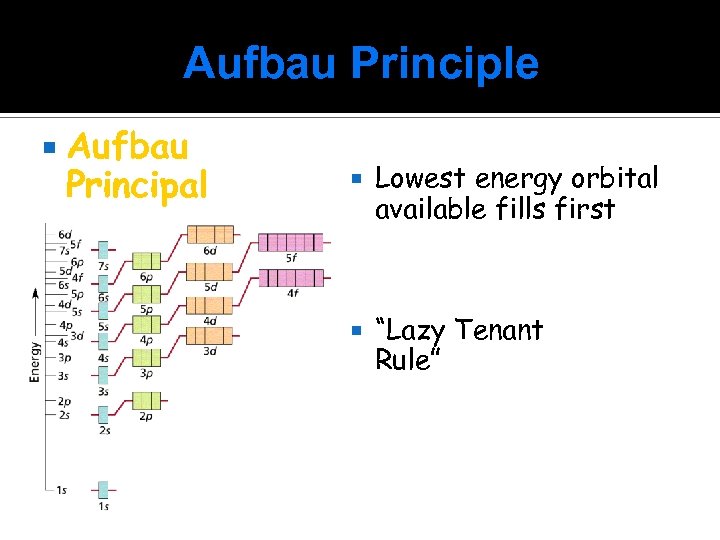
Aufbau Principle Aufbau Principal Lowest energy orbital available fills first “Lazy Tenant Rule”

Pauli Exclusion Principle No two electrons in an atom can have the same four quantum numbers. Wolfgang Pauli Every house has a different address

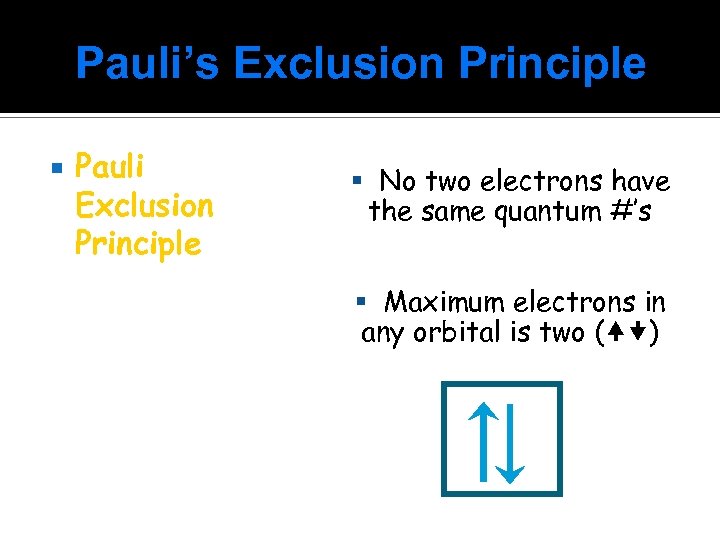
Pauli’s Exclusion Principle Pauli Exclusion Principle No two electrons have the same quantum #’s Maximum electrons in any orbital is two ( )

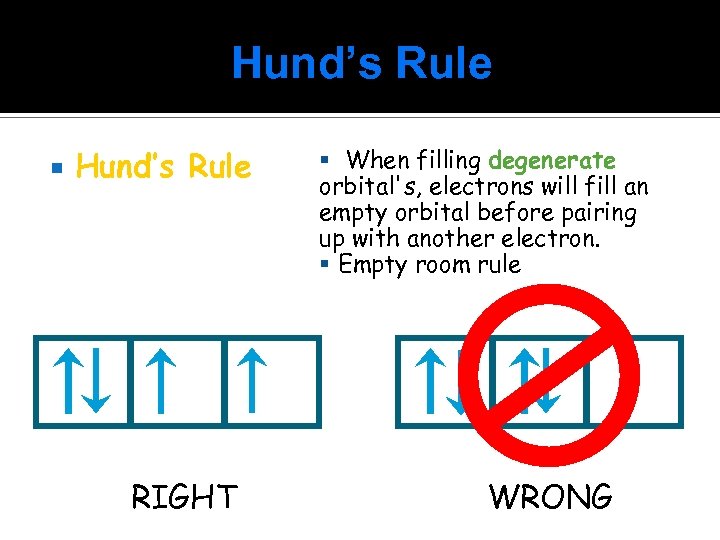
Hund’s Rule RIGHT When filling degenerate orbital's, electrons will fill an empty orbital before pairing up with another electron. Empty room rule WRONG

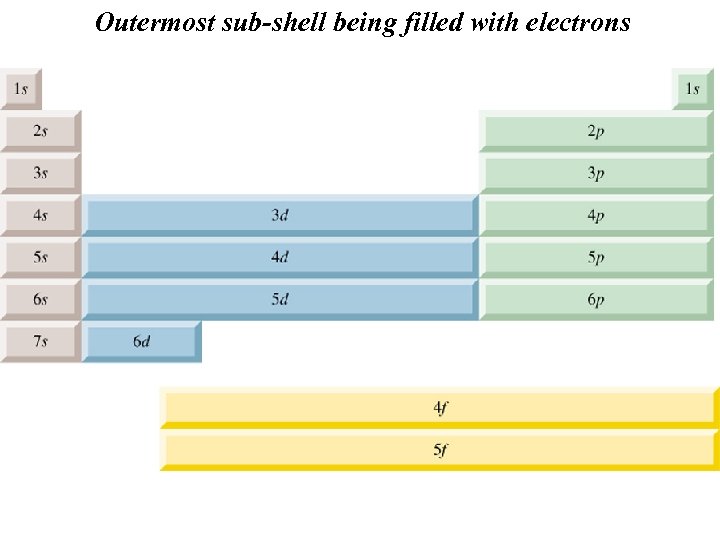
Outermost sub-shell being filled with electrons

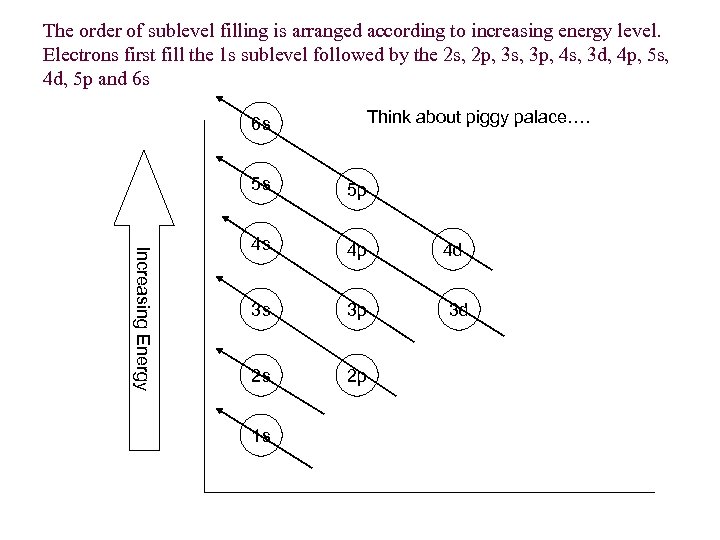
The order of sublevel filling is arranged according to increasing energy level. Electrons first fill the 1 s sublevel followed by the 2 s, 2 p, 3 s, 3 p, 4 s, 3 d, 4 p, 5 s, 4 d, 5 p and 6 s Think about piggy palace…. 6 s Increasing Energy 5 s 5 p 4 s 4 p 3 s 3 p 2 s 2 p 1 s 4 d 3 d

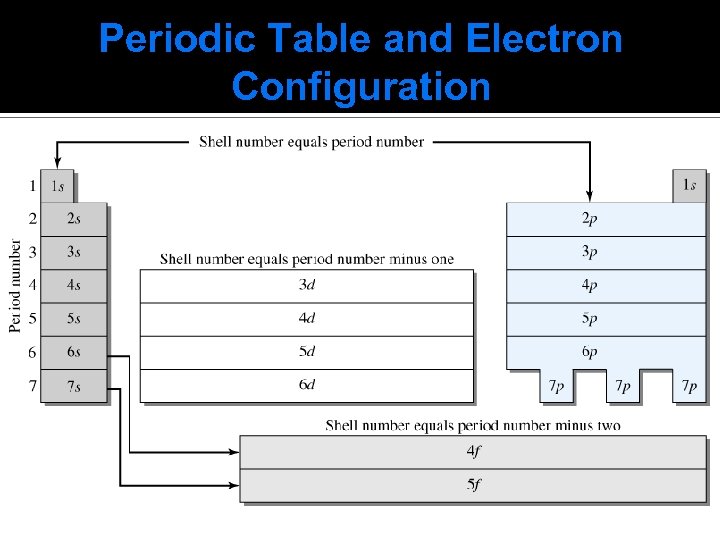
Periodic Table and Electron Configuration

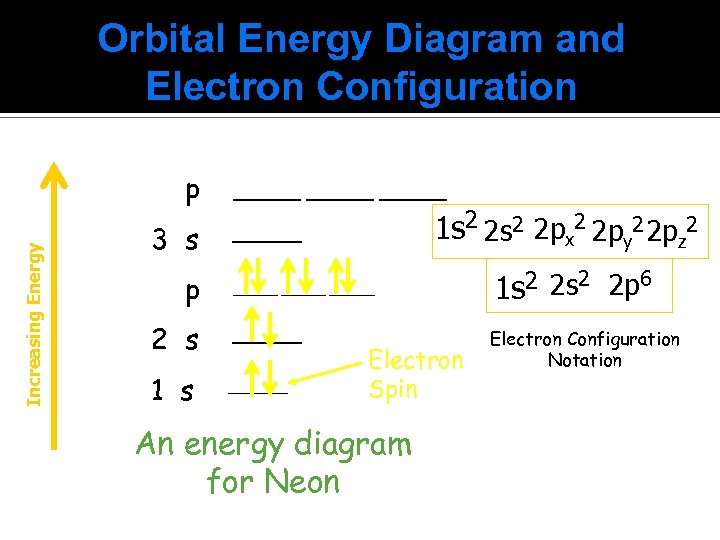
Orbital Energy Diagram and Electron Configuration Increasing Energy p 3 s p ______ 1 s 2 2 px 2 2 py 2 2 pz 2 ______ 2 s ______ 1 s ______ Electron Spin An energy diagram for Neon 1 s 2 2 p 6 Electron Configuration Notation

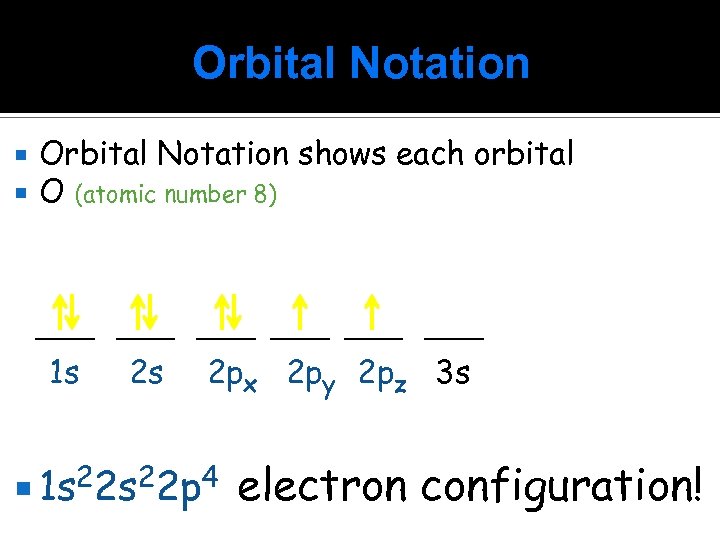
Orbital Notation shows each orbital O (atomic number 8) ____ ____ 1 s 2 s 2 px 2 py 2 pz 3 s 1 s 22 p 4 electron configuration!

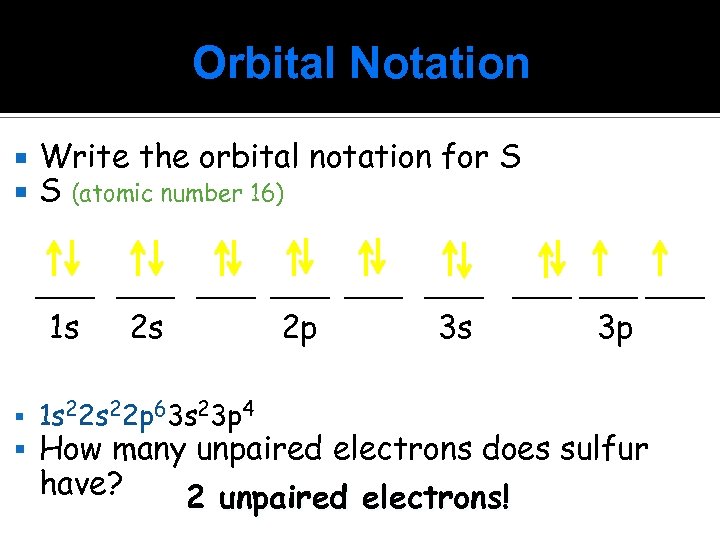
Orbital Notation Write the orbital notation for S S (atomic number 16) ____ ____ 1 s 2 s 1 s 22 p 63 s 23 p 4 2 p 3 s ____ 3 p How many unpaired electrons does sulfur have? 2 unpaired electrons!

Electron Configuration Shorthand way of writing electron configuration of atoms Ne: 10 2 1 s Number of electrons 2 2 s 6 2 p Energy sublevel Elemental Symbol and atomic number Principal energy level

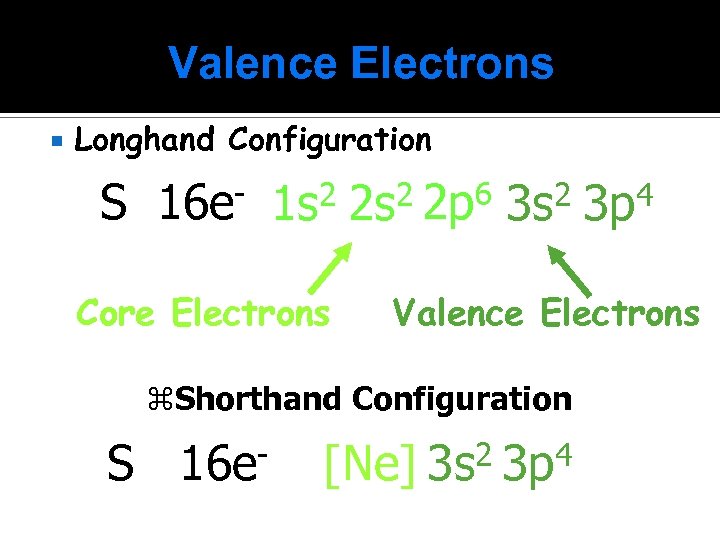
Valence Electrons Longhand Configuration S 16 e- 1 s 2 2 p 6 3 s 2 3 p 4 Core Electrons Valence Electrons z. Shorthand Configuration S 16 e [Ne] 2 3 s 4 3 p

Noble Gas Configuration Example - Germanium X X X X [Ar]4 s 2 3 d 10 4 p 2

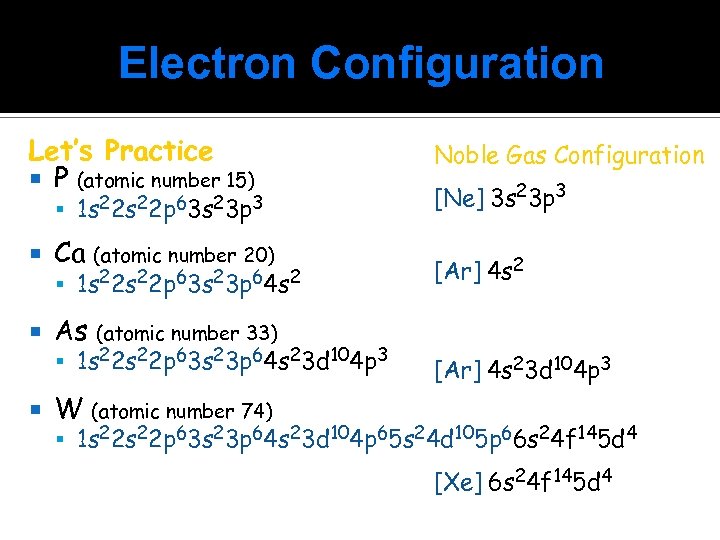
Electron Configuration Let’s Practice P (atomic number 15) 1 s 22 p 63 s 23 p 3 Ca (atomic number 20) As Noble Gas Configuration [Ne] 3 s 23 p 3 (atomic number 33) 1 s 22 s 22 p 63 s 23 p 64 s 23 d 104 p 3 [Ar] 4 s 23 d 104 p 3 W (atomic number 74) 1 s 22 p 63 s 23 p 64 s 23 d 104 p 65 s 24 d 105 p 66 s 24 f 145 d 4 [Xe] 6 s 24 f 145 d 4

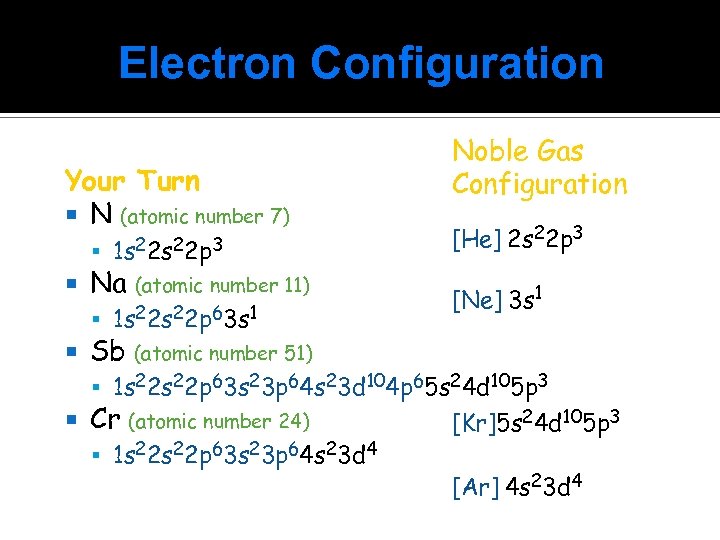
Electron Configuration Your Turn N (atomic number 7) 1 s 22 p 3 Na (atomic number 11) 1 s 22 p 63 s 1 Noble Gas Configuration [He] 2 s 22 p 3 [Ne] 3 s 1 Sb (atomic number 51) 1 s 22 p 63 s 23 p 64 s 23 d 104 p 65 s 24 d 105 p 3 Cr (atomic number 24) 1 s 22 p 63 s 23 p 64 s 23 d 4 [Kr]5 s 24 d 105 p 3 [Ar] 4 s 23 d 4

End of information for the test on Thursday 1/14

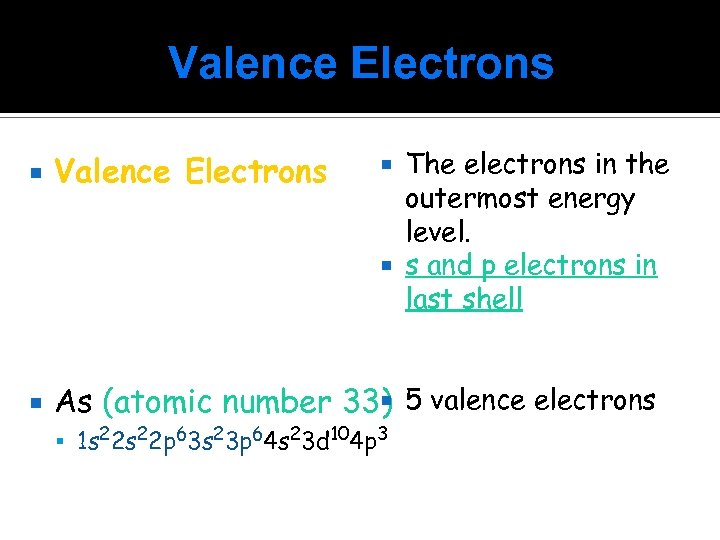
Valence Electrons The electrons in the outermost energy level. s and p electrons in last shell Valence Electrons As (atomic number 33) 5 valence electrons 1 s 22 p 63 s 23 p 64 s 23 d 104 p 3

Stability Full energy level Full sublevel Half full sublevel

Exceptions Copper Expect: [Ar] 4 s 2 3 d 9 Actual: [Ar] 4 s 1 3 d 10 Silver Expect: [Kr] 5 s 2 4 d 9 Actual: [Kr] 5 s 1 4 d 10 Chromium Molybdenum Expect: [Ar] 4 s 2 3 d 4 Actual: [Ar] 4 s 1 3 d 5 Expect: [Kr] 5 s 2 4 d 4 Actual: [Kr] 5 s 1 4 d 5 Exceptions are explained, but not predicted! Atoms are more stable with half full sublevel

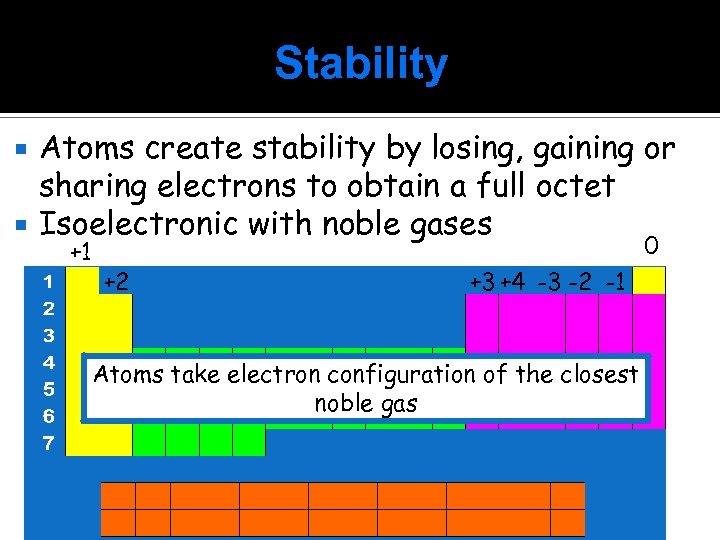
Stability Atoms create stability by losing, gaining or sharing electrons to obtain a full octet Isoelectronic with noble gases +1 0 +2 +3 +4 -3 -2 -1 Atoms take electron configuration of the closest noble gas

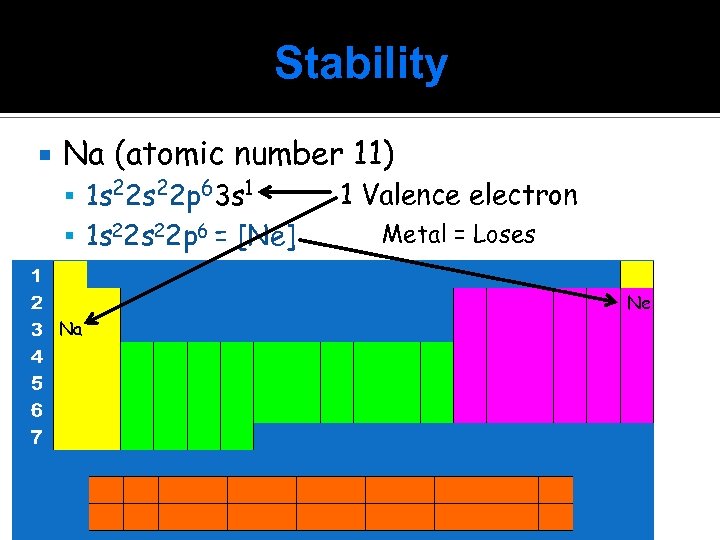
Stability Na (atomic number 11) 1 s 22 p 63 s 1 1 s 22 p 6 = [Ne] 1 Valence electron Metal = Loses Ne Na

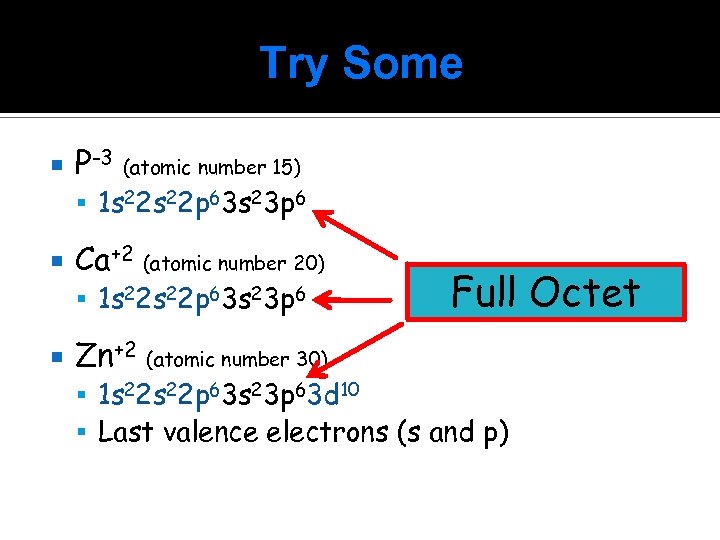
Try Some P-3 (atomic number 15) 1 s 22 p 63 s 23 p 6 Ca+2 (atomic number 20) 1 s 22 p 63 s 23 p 6 Zn+2 (atomic number 30) Full Octet 1 s 22 p 63 s 23 p 63 d 10 Last valence electrons (s and p)

Element Lithium Configuration notation Orbital notation 1 s 22 s 1 [He]2 s 1 ____ 1 s Beryllium ____ 2 p ____ 2 s ____ 2 p ____ [He]2 s 2 p 2 ____ 2 s ____ 2 p ____ 1 s 22 s 2 p 3 [He]2 s 2 p 3 ____ 2 s ____ 2 p ____ 1 s 22 s 2 p 4 [He]2 s 2 p 4 ____ 2 s ____ 2 p ____ 1 s 22 s 2 p 5 [He]2 s 2 p 5 ____ 1 s Neon ____ 2 s 1 s 22 s 2 p 2 ____ 1 s Fluorine ____ [He]2 s 2 p 1 ____ 1 s Oxygen ____ 2 p 1 s 22 s 2 p 1 ____ 1 s Nitrogen ____ [He]2 s 2 ____ 1 s Carbon ____ 2 s 1 s 22 s 2 ____ 1 s Boron Noble gas notation ____ 2 s ____ 2 p ____ 1 s 22 s 2 p 6 [He]2 s 2 p 6 ____ 1 s ____ 2 s ____ 2 p ____

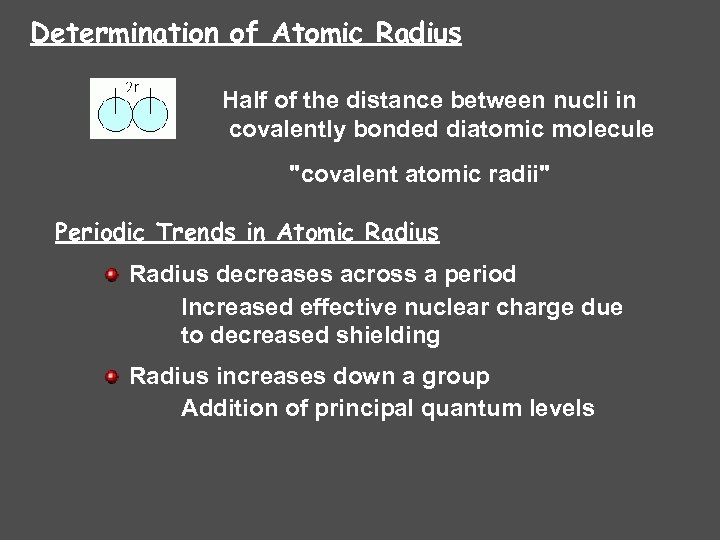
Determination of Atomic Radius Half of the distance between nucli in covalently bonded diatomic molecule "covalent atomic radii" Periodic Trends in Atomic Radius decreases across a period Increased effective nuclear charge due to decreased shielding Radius increases down a group Addition of principal quantum levels

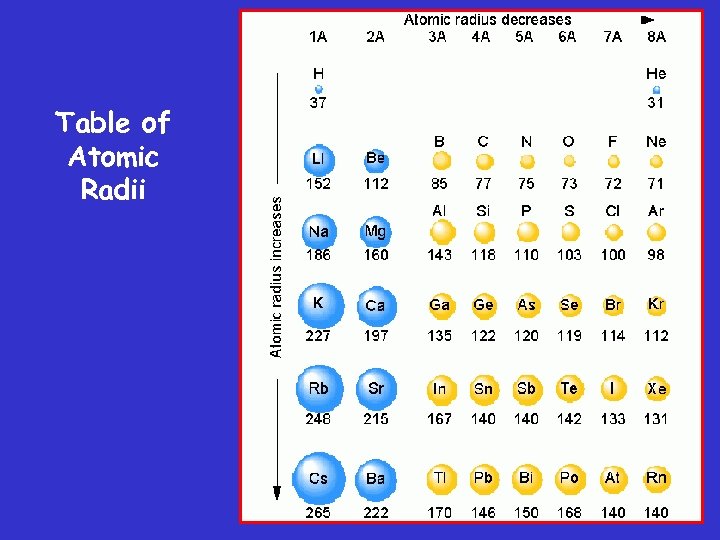
Table of Atomic Radii

Ionization Energy: the energy required to remove an electron from an atom q Increases for successive electrons taken from the same atom q Tends to increase across a period Electrons in the same quantum level do not shield as effectively as electrons in inner levels Irregularities at half filled and filled sublevels due to extra repulsion of electrons paired in orbitals, making them easier to remove q Tends to decrease down a group Outer electrons are farther from the nucleus

Electron Affinity - the energy change associated with the addition of an electron q Affinity tends to increase across a period q Affinity tends to decrease as you go down in a period Electrons farther from the nucleus experience less nuclear attraction Some irregularities due to repulsive forces in the relatively small p orbitals

Electronegativity A measure of the ability of an atom in a chemical compound to attract electrons q Electronegativities tend to increase across a period q Electronegativities tend to decrease down a group or remain the same

Ionic Radii Cations Anions Positively charged ions Smaller than the corresponding atom Negatively charged ions Larger than the corresponding atom

Table of Ion Sizes

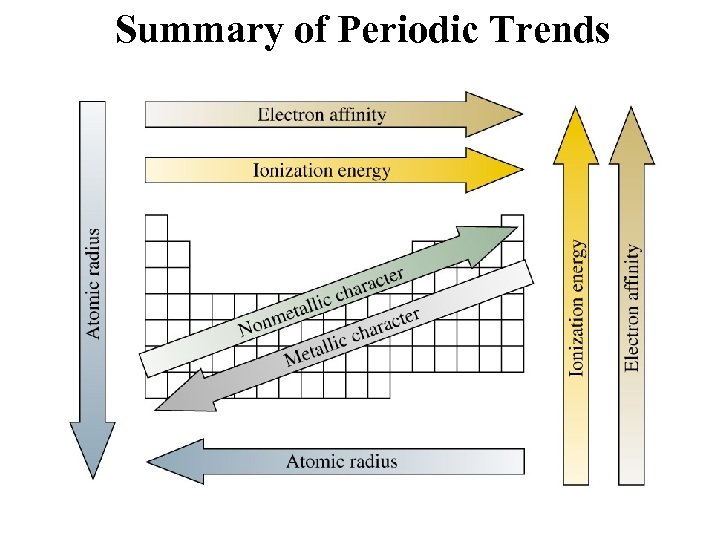
Summary of Periodic Trends