Black-Scholes-Merton_OPM.pptx
- Количество слайдов: 13

Black-Scholes-Merton OPM Fischer Black and Myron Scholes “developed” their option-pricing model in 1973. Robert Merton was the first to proclaim the model’s importance, and first to refer to the model as the Black-Scholes OPM. Scholes and Merton won the Nobel prize in 1997 for their “pioneering development” of the option pricing model. (Black had died – the Nobel prize is not given posthumously)

Assumptions of Model The underlying price follows a lognormal probability distribution. Thus, log return is normally distributed. Log returns are continuously compounded returns. The risk-free rate is known and is constant. Volatility is known and constant. No taxes or transaction costs. European-style options with no cash flows.
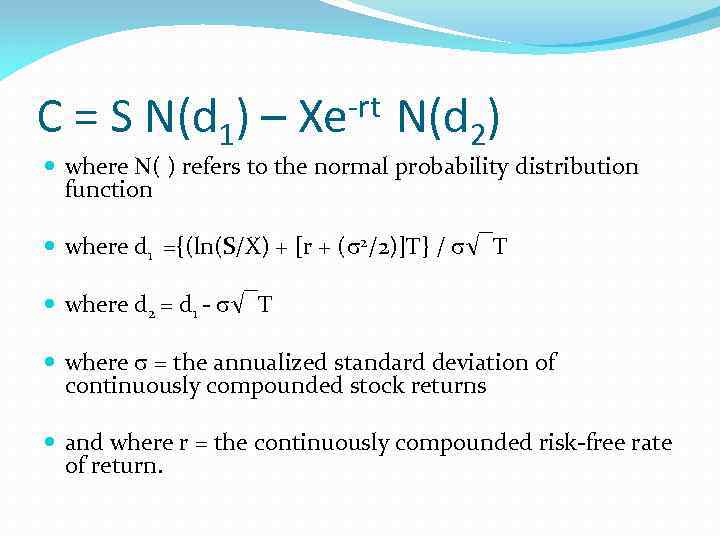
C = S N(d 1) – -rt Xe N(d 2) where N( ) refers to the normal probability distribution function where d 1 ={(ln(S/X) + [r + ( 2/2)]T} / √‾T where d 2 = d 1 - √‾T where = the annualized standard deviation of continuously compounded stock returns and where r = the continuously compounded risk-free rate of return.

Calculation Concerns Most normal probability distributions extend only to two decimal places; you’ll likely need to round your result before obtaining a value from the table. Note that the BSM model is quite sensitive to rounding errors! If r is not stated in continuous terms, take the natural log of (1 + r) to obtain the continuously compounded interest rate.

The Greeks: Delta The first derivative of option value with respect to a change in the value of the underlying. Delta ranges from 0 to 1 for a call option and from -1 to 0 for a put option. N(d 1) is an approximation for the call delta. N(d 1) – 1 is an approximation for the put delta. Without changes in underlying value, delta moves toward 1 for in-the-money calls and toward 0 for outof-the-money calls as time decays.

Delta – continued Many investors hedge their investment portfolios using delta values to determine how call option contracts to buy or sell. While this can reduce risk, theoretically the hedge must be rebalanced each time the underlying changes in value. This is referred to as “dynamic hedging” and can of course be costly in regard to time and transaction costs.

The Greeks: Gamma The second derivative of option price with respect to a change in underlying value. Gamma is larger when there is uncertainty as to whether the option will expire in- or out-of-themoney. Two situations that fit this pattern are, when the option is at-the-money or when the option is close to expiration. Under the above conditions, delta is a poor approximation of option price sensitivity.

The Greeks: Rho is the risk-free rate that corresponds to the option’s life. It is considered continuous in nature, and constant during the option life. To convert an Annual Percentage Rate (APR) to a continuous interest rate, take the natural logarithm of (1 + rate). An example would be that ln(1. 05) =. 0488 or 4. 88% continuously compounded. The value of an option is nearly insensitive to changes in rho.

The Greeks: Theta is the time to expiration of an option. Count the days until expiration and divide by 365; time is represented by a fraction of a year. For example, if there were 160 days until expiration of what was originally a six-month option, theta = 0. 4384. In general, assume that the longer the time to expiration, the more valuable the option. However, time decays with an option, even if the underlying price does not change.

Theta, continued There is a quirk with some European-style put options, and the quirk is that they can under certain conditions exhibit the characteristic that, as time to expiration expands, the option price is reduced rather than increased. By the way, for European-style options (which we do not encounter as frequently as American-style options, all of the option value can be viewed as time value, rather than decomposing into intrinsic and time values.

The Greeks: Vega Wait a minute! ‘Vega’ is not a Greek letter! Vega is the standard deviation of the continuously compounded return on the stock. Options are extremely sensitive to vega estimates, and vega is the most difficult input to estimate for an option. See next slide for discussion of historical versus implied volatility. In general, option values increase with increases in volatility.

Historical v. Implied Vega In general, theory suggests that you calculate the past volatility of the stock returns and use that as an input in the BSM model. However, it is easy to believe that historical volatility may not be what the market expects to occur for the future, so it is probably more relevant to “back out” the implied volatility figure from using the current option price in the BSM model. Traders then determine whether they think the implied volatility is “appropriate. ” Only the passage of time will confirm their beliefs.
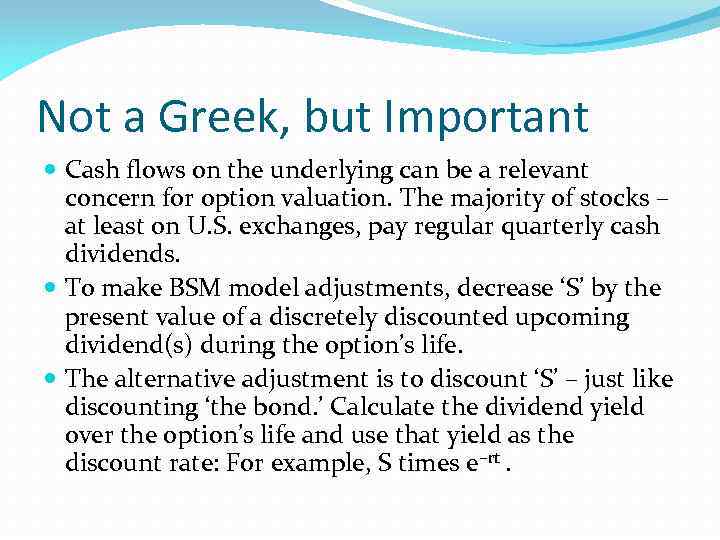
Not a Greek, but Important Cash flows on the underlying can be a relevant concern for option valuation. The majority of stocks – at least on U. S. exchanges, pay regular quarterly cash dividends. To make BSM model adjustments, decrease ‘S’ by the present value of a discretely discounted upcoming dividend(s) during the option’s life. The alternative adjustment is to discount ‘S’ – just like discounting ‘the bond. ’ Calculate the dividend yield over the option’s life and use that yield as the discount rate: For example, S times e–rt.
Black-Scholes-Merton_OPM.pptx