BJT-Transistors-Class+note.ppt
- Количество слайдов: 100
BJT Bipolar Junction Transistor • Widely used in amplifier circuits • Formed by junction of 3 materials • npn or pnp structure 0

pnp transistor 1
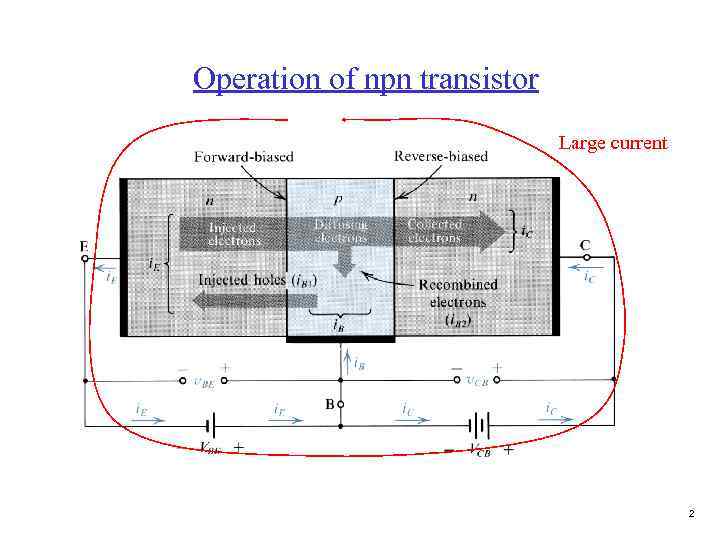
Operation of npn transistor Large current 2
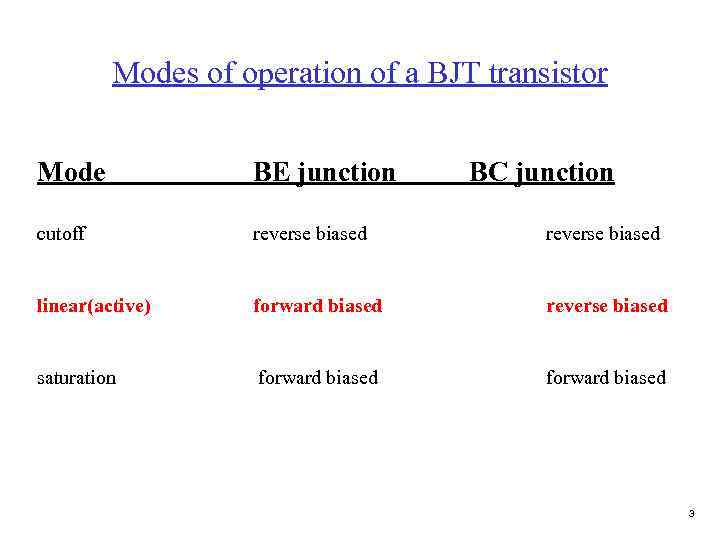
Modes of operation of a BJT transistor Mode BE junction BC junction cutoff reverse biased linear(active) forward biased reverse biased saturation forward biased 3
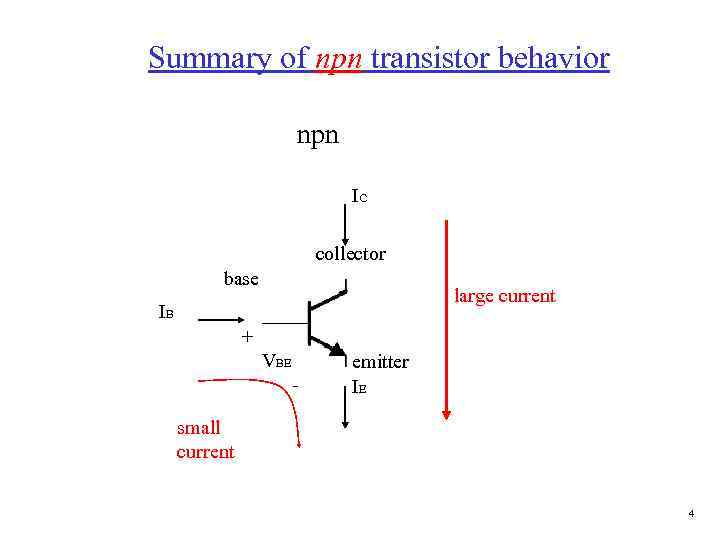
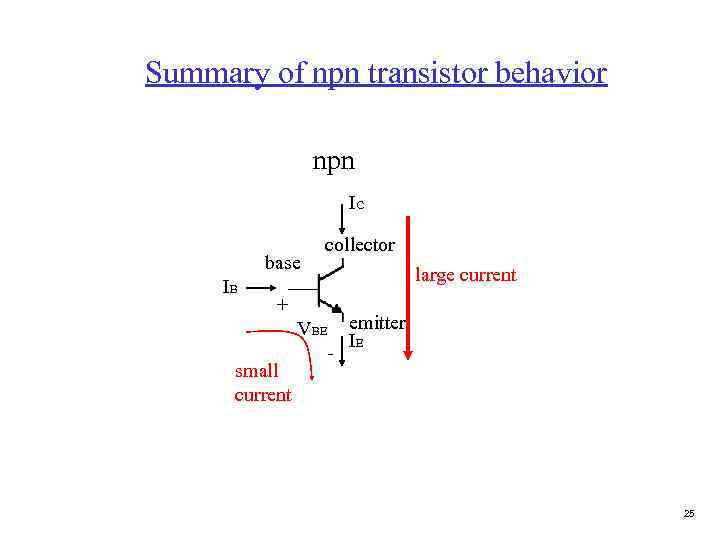
Summary of npn transistor behavior npn IC collector base large current IB + VBE - emitter IE small current 4

Summary of pnp transistor behavior pnp IC collector base large current IB + VBE - emitter IE small current 5
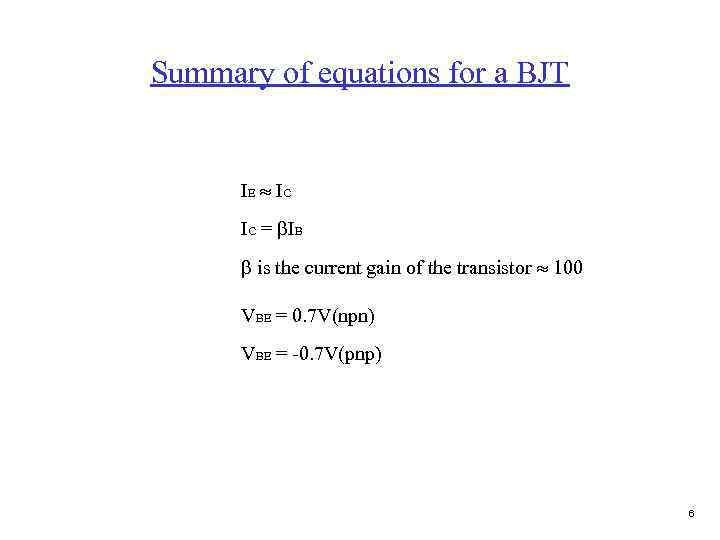
Summary of equations for a BJT IE IC IC = b. IB b is the current gain of the transistor 100 VBE = 0. 7 V(npn) VBE = -0. 7 V(pnp) 6

Graphical representation of transistor characteristics IC IB Output circuit Input circuit IE 7
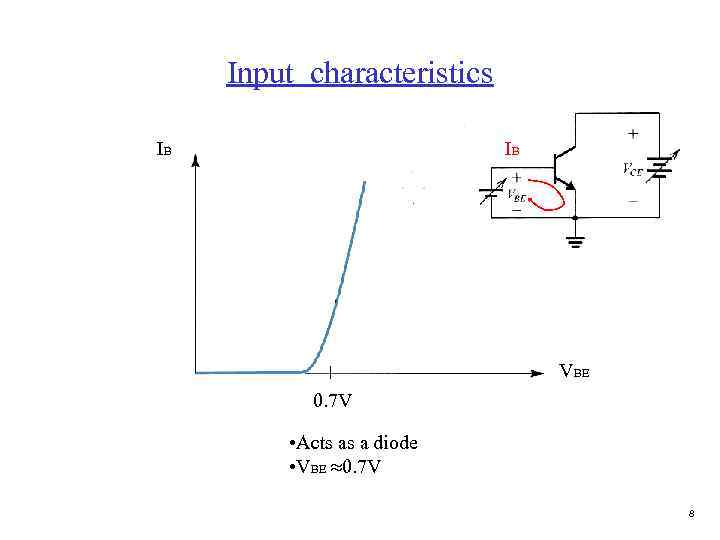
Input characteristics IB IB VBE 0. 7 V • Acts as a diode • VBE 0. 7 V 8

Output characteristics IC IC IB = 40 m. A IB = 30 m. A IB = 20 m. A IB = 10 m. A VCE Early voltage Cutoff region • At a fixed IB, IC is not dependent on VCE • Slope of output characteristics in linear region is near 0 (scale exaggerated) 9
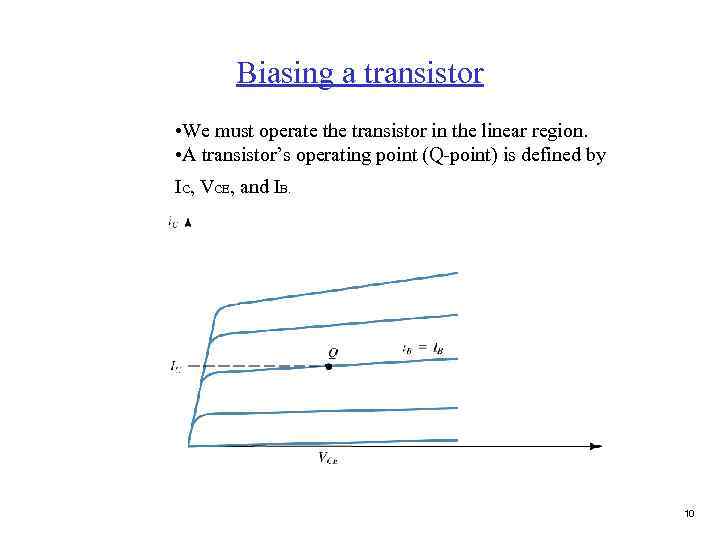
Biasing a transistor • We must operate the transistor in the linear region. • A transistor’s operating point (Q-point) is defined by IC, VCE, and IB. 10
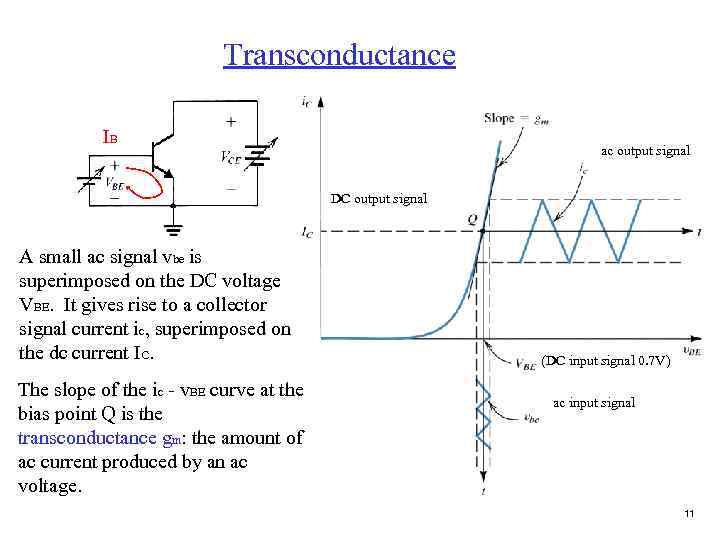
Transconductance IB ac output signal DC output signal A small ac signal vbe is superimposed on the DC voltage VBE. It gives rise to a collector signal current ic, superimposed on the dc current IC. The slope of the ic - v. BE curve at the bias point Q is the transconductance gm: the amount of ac current produced by an ac voltage. (DC input signal 0. 7 V) ac input signal 11
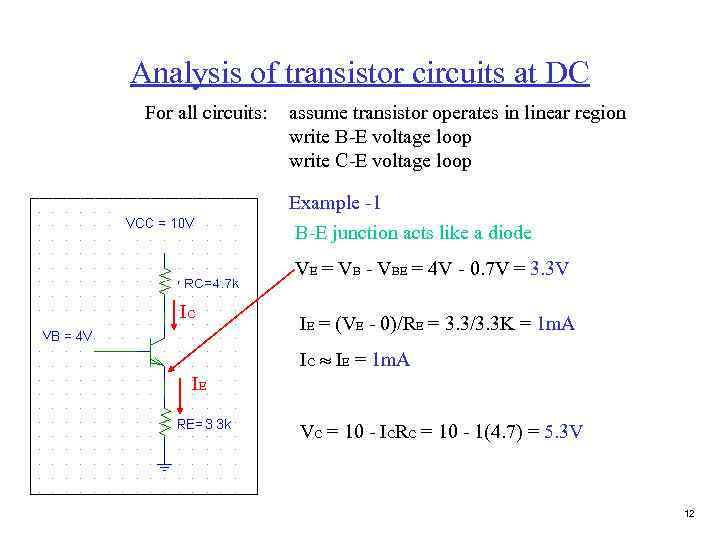
Analysis of transistor circuits at DC For all circuits: assume transistor operates in linear region write B-E voltage loop write C-E voltage loop Example -1 B-E junction acts like a diode VE = VB - VBE = 4 V - 0. 7 V = 3. 3 V IC IE = (VE - 0)/RE = 3. 3/3. 3 K = 1 m. A IC IE = 1 m. A IE VC = 10 - ICRC = 10 - 1(4. 7) = 5. 3 V 12
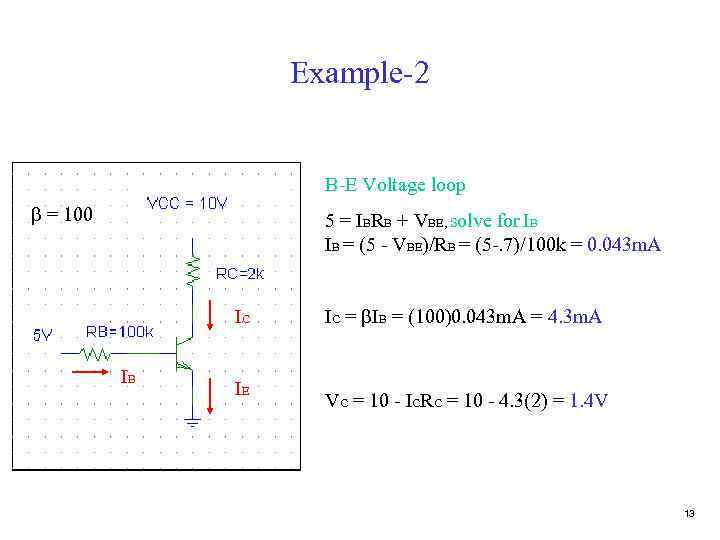
Example-2 B-E Voltage loop b = 100 5 = IBRB + VBE, solve for IB IB = (5 - VBE)/RB = (5 -. 7)/100 k = 0. 043 m. A IC IB IE IC = b. IB = (100)0. 043 m. A = 4. 3 m. A VC = 10 - ICRC = 10 - 4. 3(2) = 1. 4 V 13
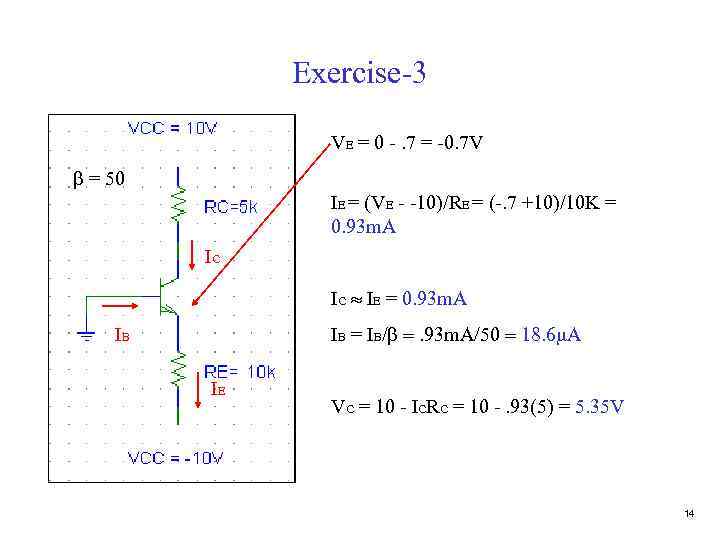
Exercise-3 VE = 0 -. 7 = -0. 7 V b = 50 IE = (VE - -10)/RE = (-. 7 +10)/10 K = 0. 93 m. A IC IC IE = 0. 93 m. A IB = IB/b =. 93 m. A/50 = 18. 6 m. A IB IE VC = 10 - ICRC = 10 -. 93(5) = 5. 35 V 14

Prob. • Use a voltage divider, RB 1 and RB 2 to bias VB to avoid two power supplies. • Make the current in the voltage divider about 10 times IB to simplify the analysis. Use VB = 3 V and I = 0. 2 m. A. (a) RB 1 and RB 2 form a voltage divider. Assume I >> IB I = VCC/(RB 1 + RB 2) I . 2 m. A = 9 /(RB 1 + RB 2) IB AND VB = VCC[RB 2/(RB 1 + RB 2)] 3 = 9 [RB 2/(RB 1 + RB 2)], Solve for RB 1 and RB 2. RB 1 = 30 KW, and RB 2 = 15 KW. 15
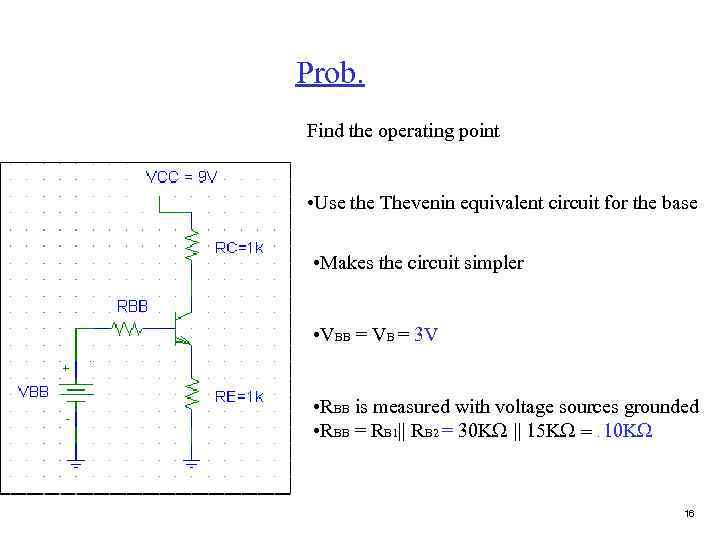
Prob. Find the operating point • Use the Thevenin equivalent circuit for the base • Makes the circuit simpler • VBB = VB = 3 V • RBB is measured with voltage sources grounded • RBB = RB 1|| RB 2 = 30 KW || 15 KW =. 10 KW 16

Prob. Write B-E loop and C-E loop B-E loop VBB = IBRBB + VBE +IERE IE =2. 09 m. A C-E loop VCC = ICRC + VCE +IERE VCE =4. 8 V B-E loop This is how all DC circuits are analyzed and designed! 17
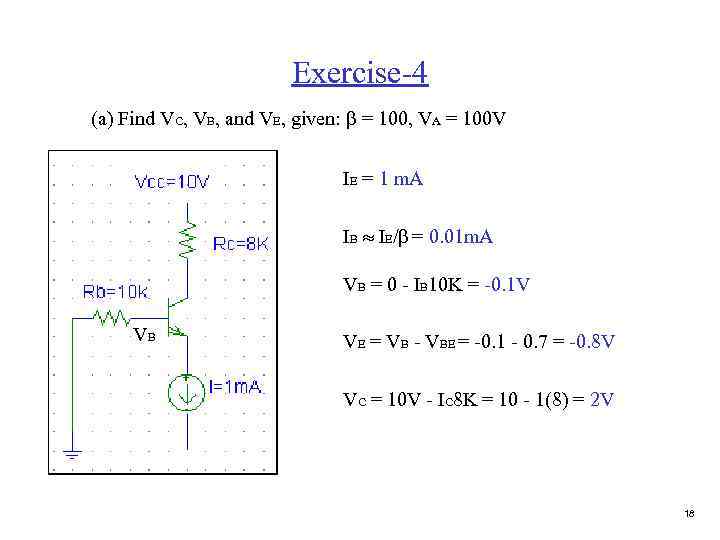
Exercise-4 (a) Find VC, VB, and VE, given: b = 100, VA = 100 V IE = 1 m. A IB IE/b = 0. 01 m. A VB = 0 - IB 10 K = -0. 1 V VB VE = VB - VBE = -0. 1 - 0. 7 = -0. 8 V VC = 10 V - IC 8 K = 10 - 1(8) = 2 V 18
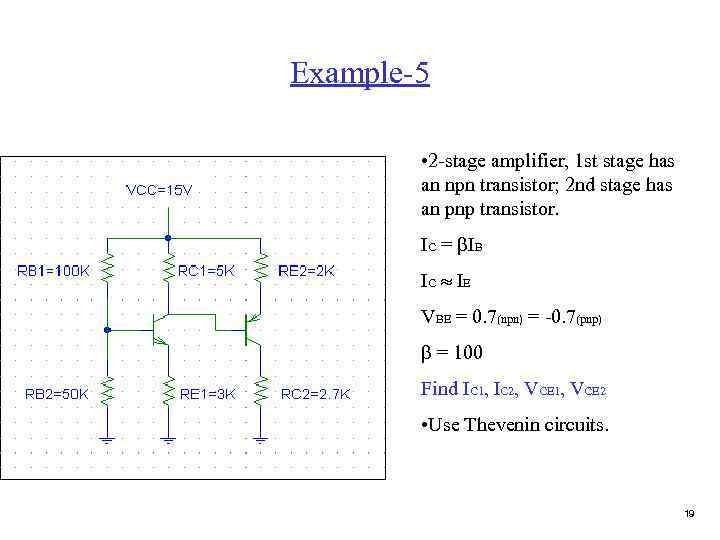
Example-5 • 2 -stage amplifier, 1 st stage has an npn transistor; 2 nd stage has an pnp transistor. IC = b. IB IC IE VBE = 0. 7(npn) = -0. 7(pnp) b = 100 Find IC 1, IC 2, VCE 1, VCE 2 • Use Thevenin circuits. 19

Example -5 • RBB 1 = RB 1||RB 2 = 33 K • VBB 1 = VCC[RB 2/(RB 1+RB 2)] VBB 1 = 15[50 K/150 K] = 5 V Stage 1 IB 1 IE 1 • B-E loop VBB 1 = IB 1 RBB 1 + VBE +IE 1 RE 1 Use IB 1 IE 1/ b 5 = IE 133 K / 100 +. 7 + IE 13 K IE 1 = 1. 3 m. A 20
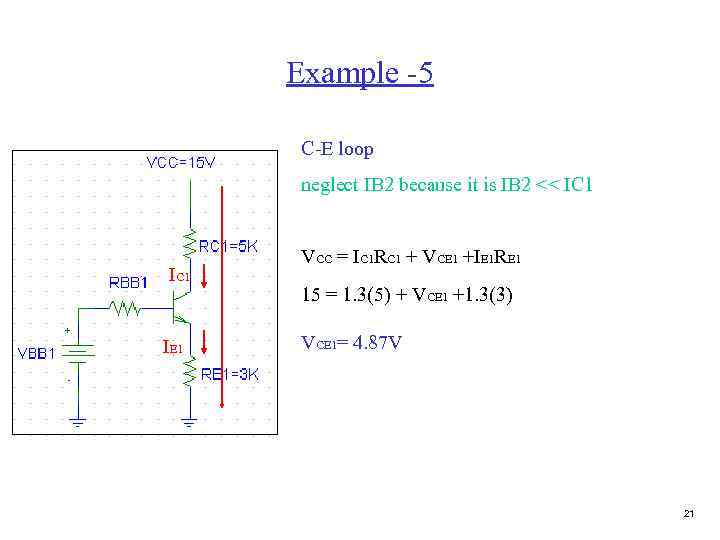
Example -5 C-E loop neglect IB 2 because it is IB 2 << IC 1 IE 1 VCC = IC 1 RC 1 + VCE 1 +IE 1 RE 1 15 = 1. 3(5) + VCE 1 +1. 3(3) VCE 1= 4. 87 V 21

Example-5 Stage 2 • B-E loop IE 2 VCC = IE 2 RE 2 + VEB +IB 2 RBB 2 + VBB 2 15 = IE 2(2 K) +. 7 +IB 2 (5 K) + 4. 87 + 1. 3(3) Use IB 2 IE 2/ b, solve for IE 2 IB 2 IE 2 = 2. 8 m. A 22
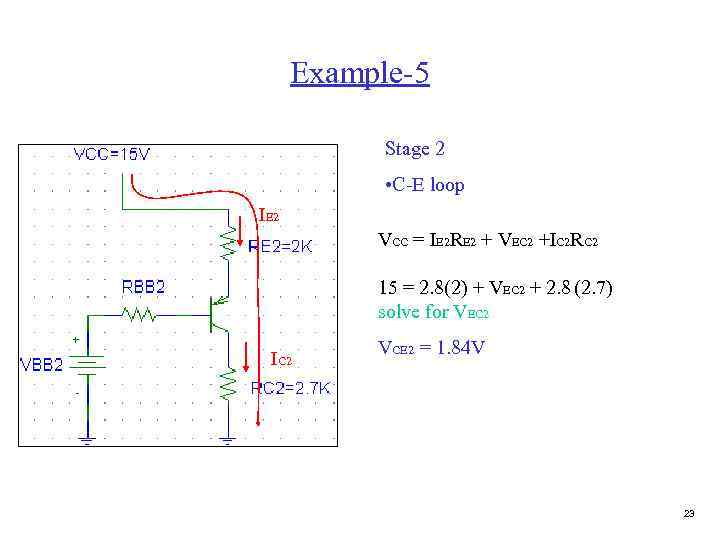
Example-5 Stage 2 • C-E loop IE 2 VCC = IE 2 RE 2 + VEC 2 +IC 2 RC 2 15 = 2. 8(2) + VEC 2 + 2. 8 (2. 7) solve for VEC 2 IC 2 VCE 2 = 1. 84 V 23

Summary of DC problem • Bias transistors so that they operate in the linear region B-E junction forward biased, C-E junction reversed biased • Use VBE = 0. 7 (npn), IC IE, IC = b. IB • Represent base portion of circuit by the Thevenin circuit • Write B-E, and C-E voltage loops. • For analysis, solve for IC, and VCE. • For design, solve for resistor values (IC and VCE specified). 24
Summary of npn transistor behavior npn IC base IB collector large current + VBE small current - emitter IE 25

Transistor as an amplifier • Transistor circuits are analyzed and designed in terms of DC and ac versions of the same circuit. • An ac signal is usually superimposed on the DC circuit. • The location of the operating point (values of IC and VCE) of the transistor affects the ac operation of the circuit. • There at least two ac parameters determined from DC quantities. 26

Transconductance IB ac output signal DC output signal A small ac signal vbe is superimposed on the DC voltage VBE. It gives rise to a collector signal current ic, superimposed on the dc current IC. The slope of the ic - v. BE curve at the bias point Q is the transconductance gm: the amount of ac current produced by an ac voltage. (DC input signal 0. 7 V) ac input signal 27
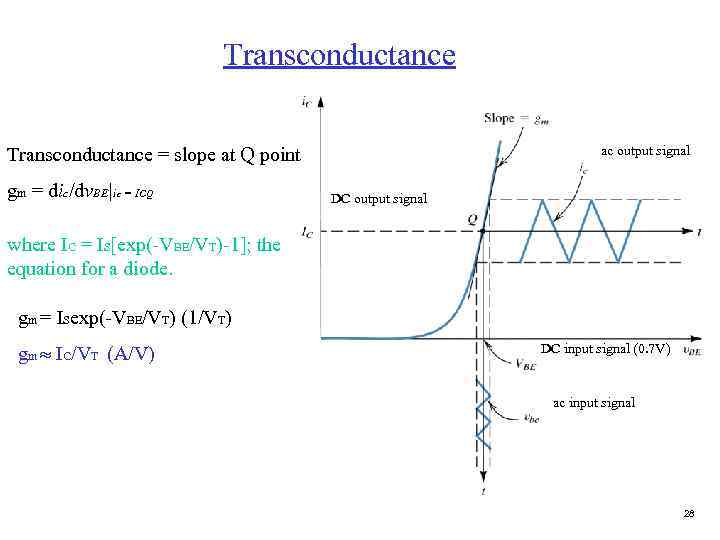
Transconductance ac output signal Transconductance = slope at Q point gm = dic/dv. BE|ic = ICQ DC output signal where IC = IS[exp(-VBE/VT)-1]; the equation for a diode. gm = ISexp(-VBE/VT) (1/VT) gm IC/VT (A/V) DC input signal (0. 7 V) ac input signal 28
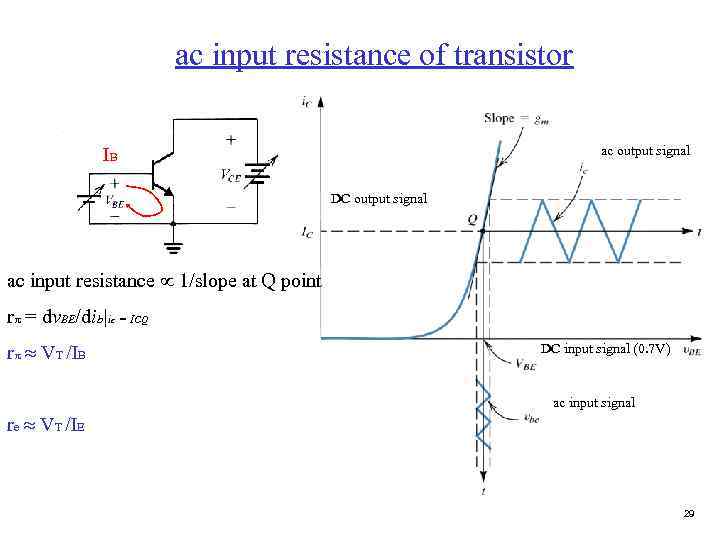
ac input resistance of transistor ac output signal IB DC output signal ac input resistance 1/slope at Q point rp = dv. BE/dib|ic = ICQ rp VT /IB DC input signal (0. 7 V) ac input signal re VT /IE 29

Small-signal equivalent circuit models • ac model • Hybrid-p model • They are equivalent • Works in linear region only 30

Steps to analyze a transistor circuit 1 DC problem Set ac sources to zero, solve for DC quantities, IC and VCE. 2 Determine ac quantities from DC parameters Find gm, rp, and re. 3 ac problem Set DC sources to zero, replace transistor by hybrid-p model, find ac quantites, Rin, Rout, Av, and Ai. 31
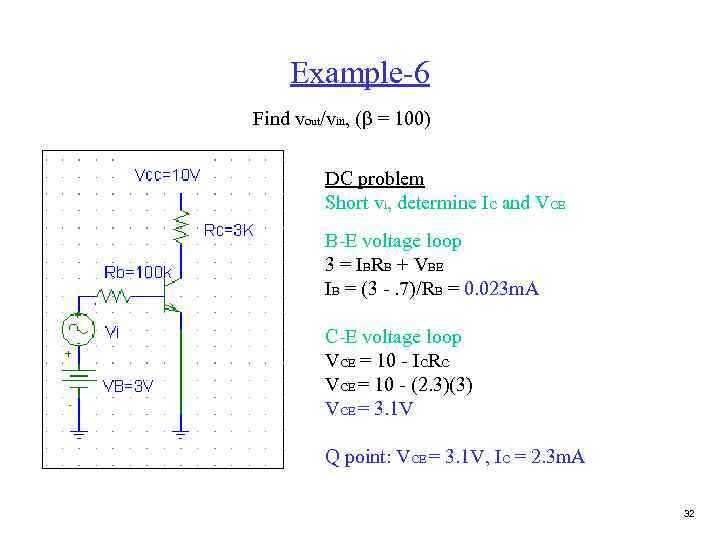
Example-6 Find vout/vin, (b = 100) DC problem Short vi, determine IC and VCE B-E voltage loop 3 = IBRB + VBE IB = (3 -. 7)/RB = 0. 023 m. A C-E voltage loop VCE = 10 - ICRC VCE = 10 - (2. 3)(3) VCE = 3. 1 V Q point: VCE = 3. 1 V, IC = 2. 3 m. A 32
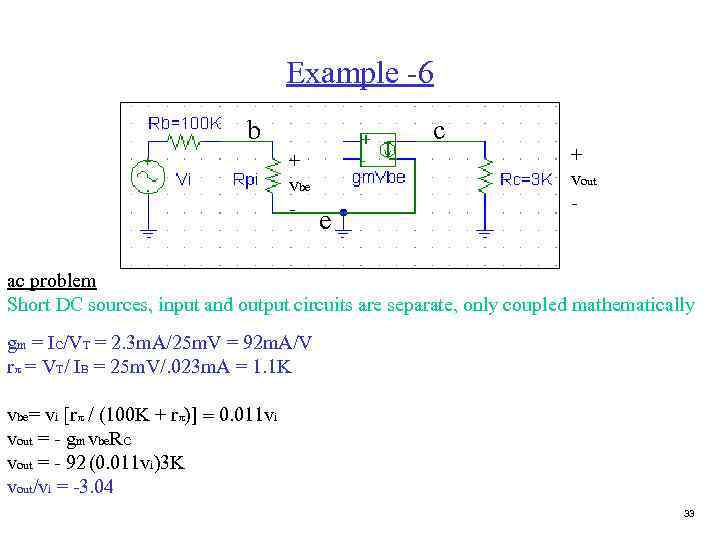
Example -6 b c + vbe - e + vout - ac problem Short DC sources, input and output circuits are separate, only coupled mathematically gm = IC/VT = 2. 3 m. A/25 m. V = 92 m. A/V rp = VT/ IB = 25 m. V/. 023 m. A = 1. 1 K vbe= vi [rp / (100 K + rp)] = 0. 011 vi vout = - gm vbe. RC vout = - 92 (0. 011 vi)3 K vout/vi = -3. 04 33

Exercise-7 Find gm, rp, and r 0, given: b = 100, VA = 100 V, IC=1 m. A gm = IC/VT = 1 m. A/25 m. V = 40 m. A/V rp = VT/ IB = 25 m. V/. 01 m. A = 2. 5 K r 0 = output resistance of transistor r 0 = 1/slope of transistor output characteristics r 0 = | VA|/IC = 100 K 34
Summary of transistor analysis • Transistor circuits are analyzed and designed in terms of DC and ac versions of the same circuit. • An ac signal is usually superimposed on the DC circuit. • The location of the operating point (values of IC and VCE) of the transistor affects the ac operation of the circuit. • There at least two ac parameters determined from DC quantities. 35

Steps to analyze a transistor circuit 1 DC Analysis quantities, IC and VCE. 2 Set ac sources to zero, solve for DC Determine ac quantities from DC parameters Find gm, rp, and ro. 3 AC Analysis Set DC sources to zero, replace transistor by hybrid-p model, find ac quantities, Rin, Rout, Av, and Ai. ro 36
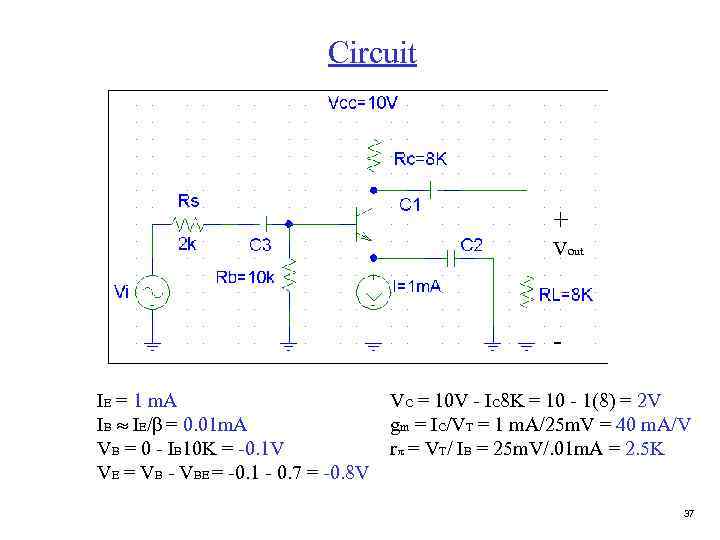
Circuit + Vout IE = 1 m. A IB IE/b = 0. 01 m. A VB = 0 - IB 10 K = -0. 1 V VE = VB - VBE = -0. 1 - 0. 7 = -0. 8 V VC = 10 V - IC 8 K = 10 - 1(8) = 2 V gm = IC/VT = 1 m. A/25 m. V = 40 m. A/V rp = VT/ IB = 25 m. V/. 01 m. A = 2. 5 K 37
![ac equivalent circuit b c e vbe = (Rb||Rpi)/ [(Rb||Rpi) +Rs]vi vbe = 0. ac equivalent circuit b c e vbe = (Rb||Rpi)/ [(Rb||Rpi) +Rs]vi vbe = 0.](https://present5.com/presentation/133265323_177823045/image-39.jpg)
ac equivalent circuit b c e vbe = (Rb||Rpi)/ [(Rb||Rpi) +Rs]vi vbe = 0. 5 vi + vout - Neglecting Ro vout = -(gmvbe)||(Rc ||RL) Av = vout/vi = - 80 vout = -(gmvbe)||(Ro||Rc ||RL) vout = -154 vbe Av = vout/vi = - 77 38
Prob. b=100 + Vout - 39

Prob. b c + ib e Rin (a) Find Rin = Rpi = VT/IB = (25 m. V)100/. 1 = 2. 5 KW (c) Find Rout = Rc = 47 KW Vout = bib Rout (b) Find Av = vout/vin vout = - bib Rc vin = ib (R + Rpi) Av = vout/vin = - bib Rc/ ib (R + Rpi = - b. Rc/(R + Rpi) = - 100(47 K)/(100 K + 2. 5 K) = - 37. 6 40
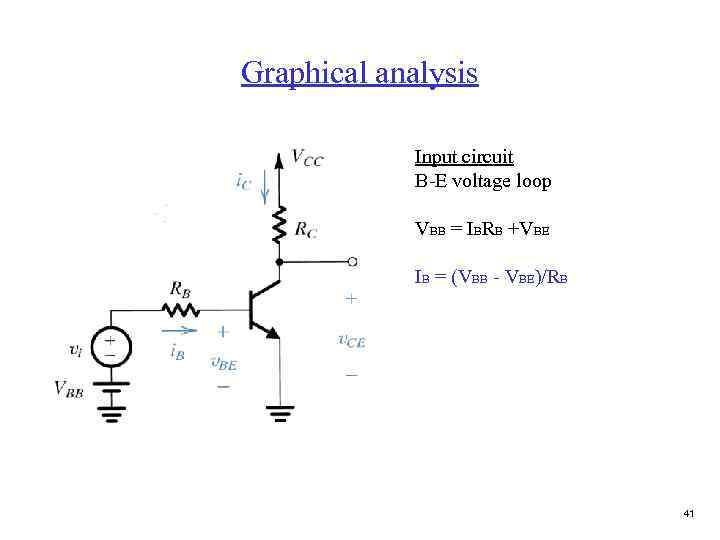
Graphical analysis Input circuit B-E voltage loop VBB = IBRB +VBE IB = (VBB - VBE)/RB 41
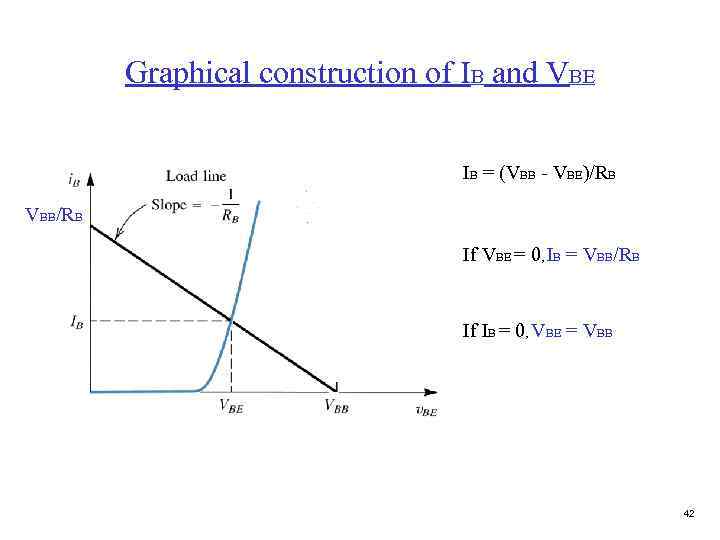
Graphical construction of IB and VBE IB = (VBB - VBE)/RB VBB/RB If VBE = 0, IB = VBB/RB If IB = 0, VBE = VBB 42

Load line Output circuit C-E voltage loop VCC = ICRC +VCE IC = (VCC - VCE)/RC 43
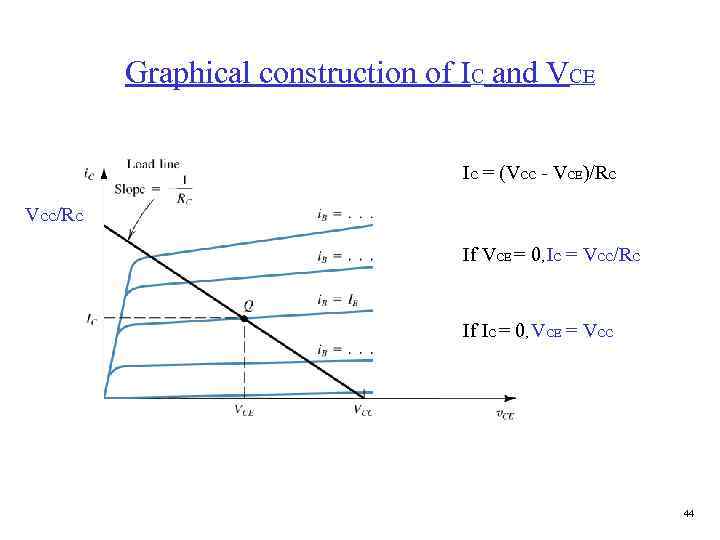
Graphical construction of IC and VCE IC = (VCC - VCE)/RC VCC/RC If VCE = 0, IC = VCC/RC If IC = 0, VCE = VCC 44
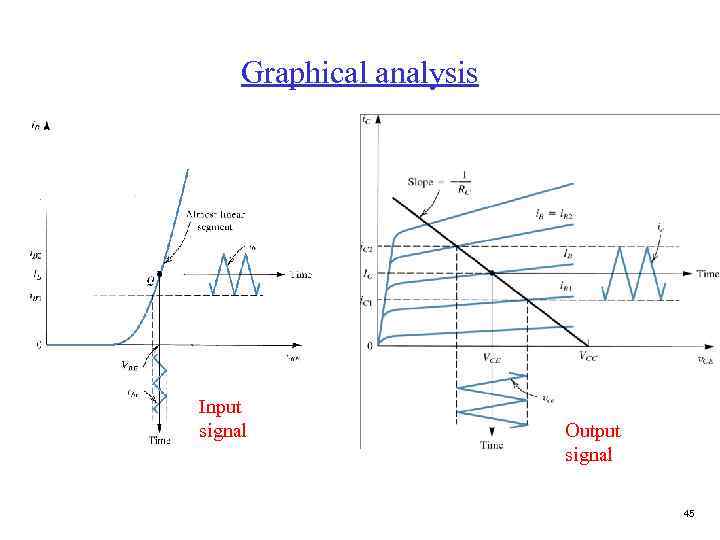
Graphical analysis Input signal Output signal 45
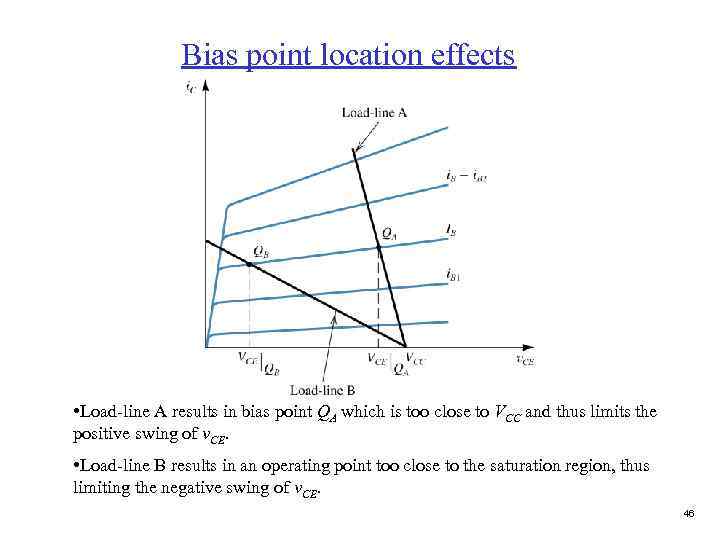
Bias point location effects • Load-line A results in bias point QA which is too close to VCC and thus limits the positive swing of v. CE. • Load-line B results in an operating point too close to the saturation region, thus limiting the negative swing of v. CE. 46
Basic single-stage BJT amplifier configurations We will study 3 types of BJT amplifiers • CE - common emitter, used for AV, Ai, and general purpose • CE with RE - common emitter with RE, same as CE but more stable • CC common collector, used for Ai, low output resistance, used as an output stage CB common base (not covered) 47
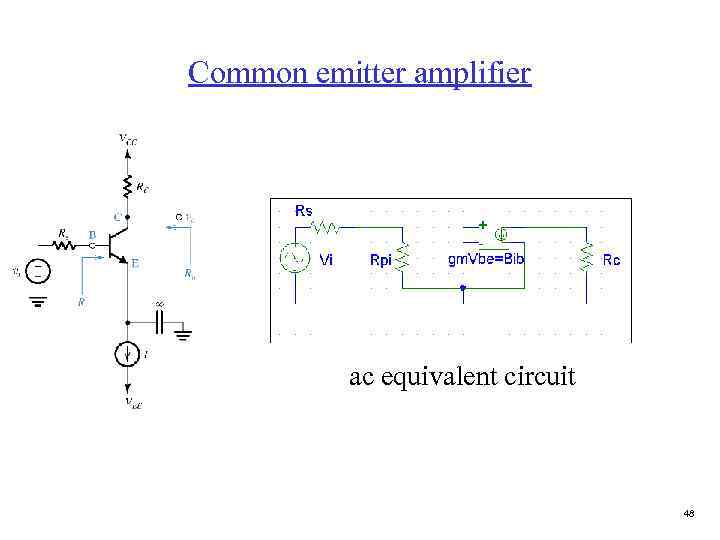
Common emitter amplifier ac equivalent circuit 48
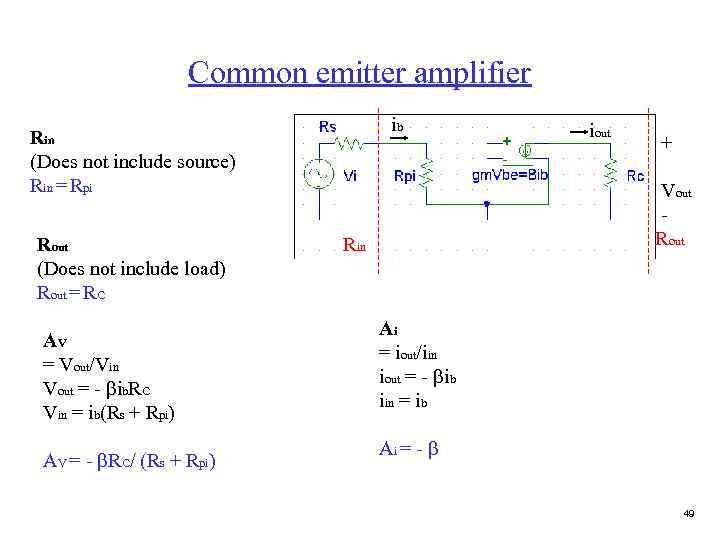
Common emitter amplifier ib Rin (Does not include source) Rin = Rpi Rout (Does not include load) Rout = RC AV = Vout/Vin Vout = - bib. RC Vin = ib(Rs + Rpi) AV = - b. RC/ (Rs + Rpi) iout + Vout Rin Ai = iout/iin iout = - bib iin = ib Ai = - b 49
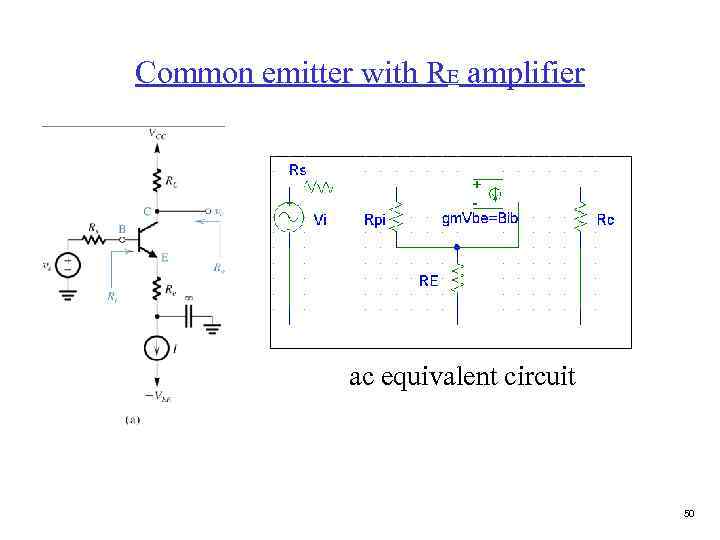
Common emitter with RE amplifier ac equivalent circuit 50
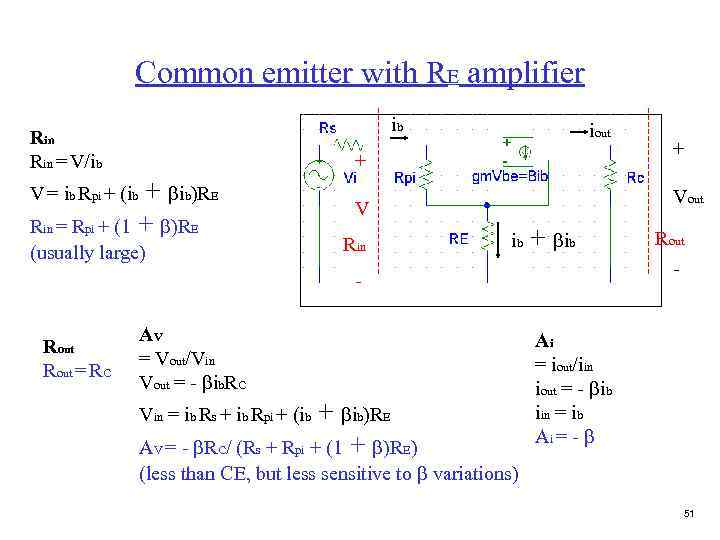
Common emitter with RE amplifier ib Rin = V/ib iout + + bib)RE Rin = Rpi + (1 + b)RE V = ib Rpi + (ib (usually large) Vout V Rin ib + bib Rout = RC AV = Vout/Vin Vout = - bib. RC + bib)RE AV = - b. RC/ (Rs + Rpi + (1 + b)RE) + Vin = ib Rs + ib Rpi + (ib Rout - Ai = iout/iin iout = - bib iin = ib Ai = - b (less than CE, but less sensitive to b variations) 51
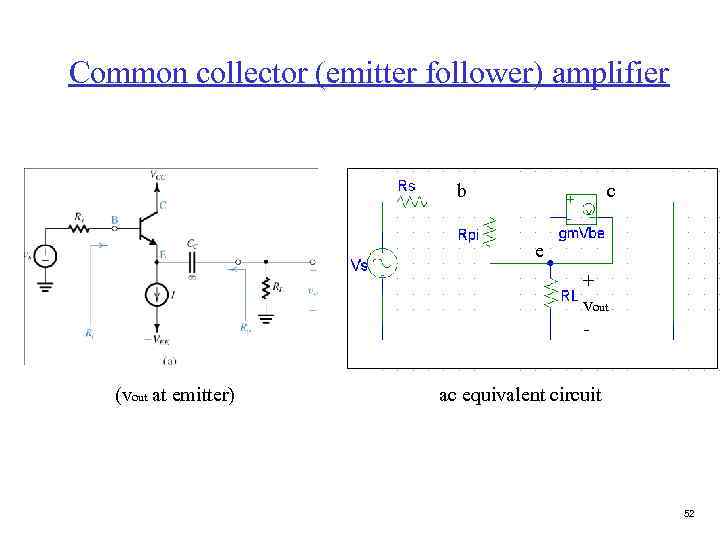
Common collector (emitter follower) amplifier b c e + vout - (vout at emitter) ac equivalent circuit 52

Common collector amplifier ib + Rin = V/ib + bib)RL Rin = Rpi + (1 + b)RL V = ib Rpi + (ib V ib + bib Rin + vout - AV = vout/vs vout = (ib + bib)RL AV = (1+ b)RL/ (Rs + Rpi + (1 + b)RL) vs = ib Rs + ib Rpi + (ib (always < 1) 53
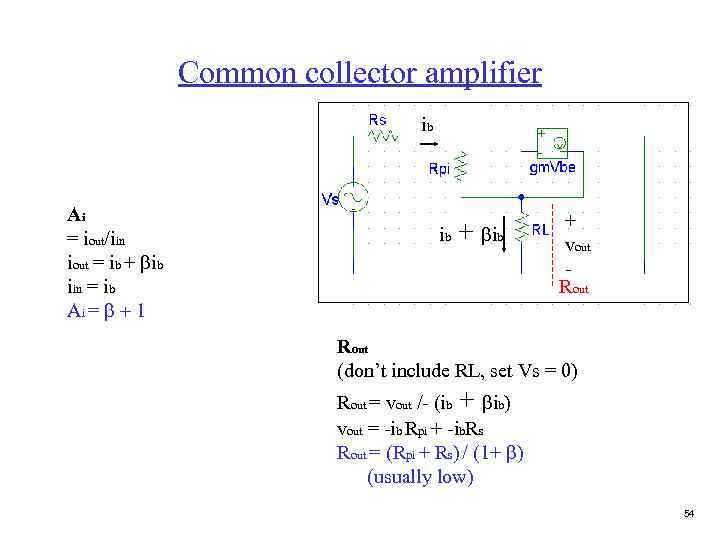
Common collector amplifier ib Ai = iout/iin iout = ib + bib iin = ib Ai = b + 1 ib + bib + vout Rout (don’t include RL, set Vs = 0) Rout = vout /- (ib + bib) vout = -ib Rpi + -ib. Rs Rout = (Rpi + Rs) / (1+ b) (usually low) 54
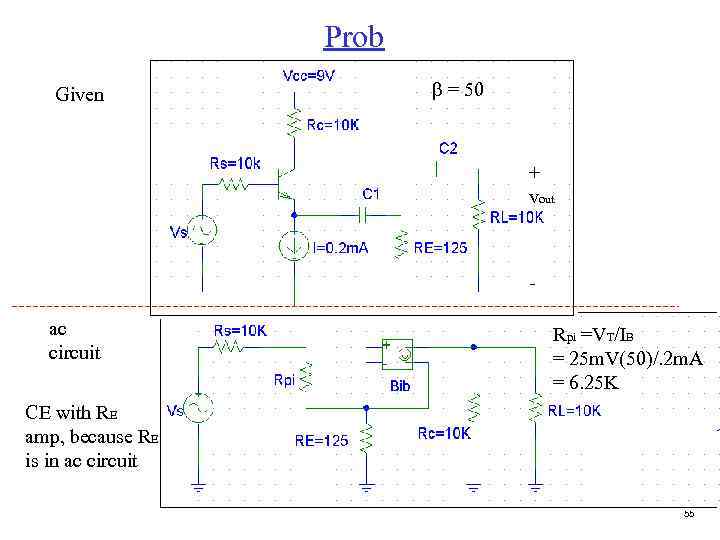
Prob Given b = 50 + vout ac circuit Rpi =VT/IB = 25 m. V(50)/. 2 m. A = 6. 25 K CE with RE amp, because RE is in ac circuit 55
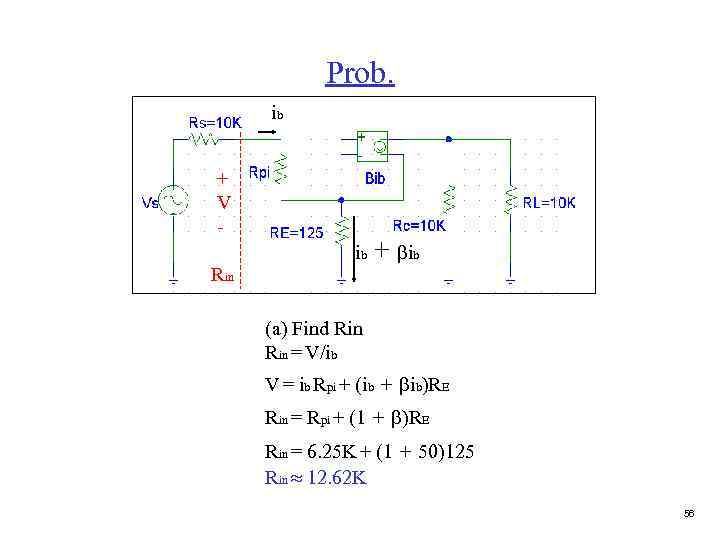
Prob. ib + V ib Rin + bib (a) Find Rin = V/ib V = ib Rpi + (ib + bib)RE Rin = Rpi + (1 + b)RE Rin = 6. 25 K + (1 + 50)125 Rin 12. 62 K 56

Prob. ib -bib + vout ib + bib - (b) Find AV = vout/vs vout = - bib(RC||RL) vs = ib Rs + ib Rpi + (ib + bib)RE AV = - b (RC||RL) / (Rs + Rpi + (1 + b)RE) AV = - 50 (10 K||10 K) /(10 K + 6. 25 Ki + (1 + 50)125) AV - 11 57
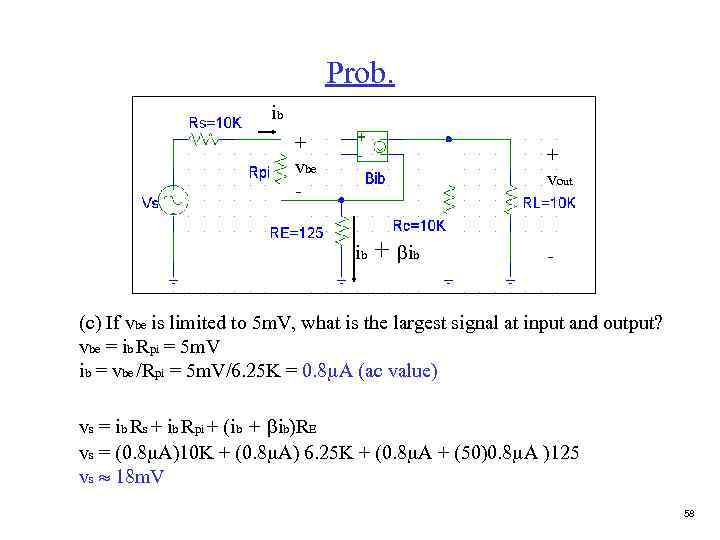
Prob. ib + vbe - + vout ib + bib - (c) If vbe is limited to 5 m. V, what is the largest signal at input and output? vbe = ib Rpi = 5 m. V ib = vbe /Rpi = 5 m. V/6. 25 K = 0. 8 m. A (ac value) vs = ib Rs + ib Rpi + (ib + bib)RE vs = (0. 8 m. A)10 K + (0. 8 m. A) 6. 25 K + (0. 8 m. A + (50)0. 8 m. A )125 vs 18 m. V 58
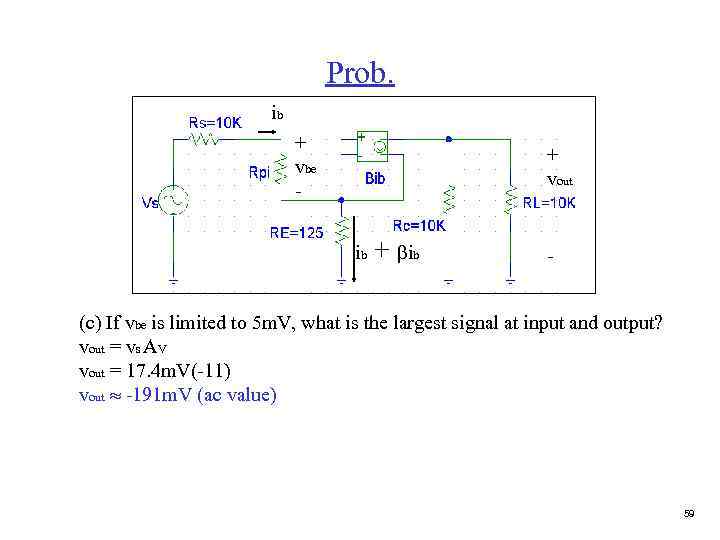
Prob. ib + vbe - + vout ib + bib - (c) If vbe is limited to 5 m. V, what is the largest signal at input and output? vout = vs AV vout = 17. 4 m. V(-11) vout -191 m. V (ac value) 59

Prob. b = 100 Using this circuit, design an amp with: IE = 2 m. A AV = -8 current in voltage divider I = 0. 2 m. A (CE amp because RE is not in ac circuit) Voltage divider Vcc/I = 9/0. 2 m. A = 45 K = R 1 + R 2 Choose VB 1/3 Vcc to put operating point near the center of the transistor characteristics R 2/(R 1 + R 2) = 3 V Combining gives, R 1 = 30 K, R 2 = 15 K 60
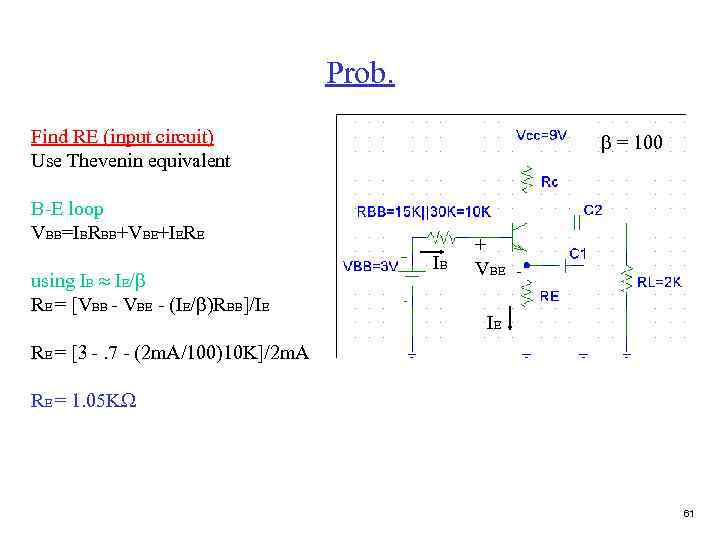
Prob. Find RE (input circuit) Use Thevenin equivalent b = 100 B-E loop VBB=IBRBB+VBE+IERE using IB IE/b RE = [VBB - VBE - (IE/b)RBB]/IE IB + VBE - IE RE = [3 -. 7 - (2 m. A/100)10 K]/2 m. A RE = 1. 05 KW 61
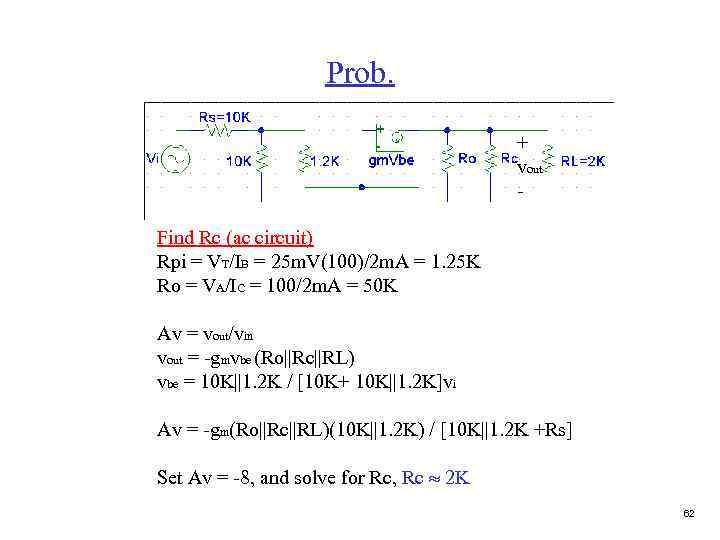
Prob. + vout Find Rc (ac circuit) Rpi = VT/IB = 25 m. V(100)/2 m. A = 1. 25 K Ro = VA/IC = 100/2 m. A = 50 K Av = vout/vin vout = -gmvbe (Ro||Rc||RL) vbe = 10 K||1. 2 K / [10 K+ 10 K||1. 2 K]vi Av = -gm(Ro||Rc||RL)(10 K||1. 2 K) / [10 K||1. 2 K +Rs] Set Av = -8, and solve for Rc, Rc 2 K 62

CE amplifier 63
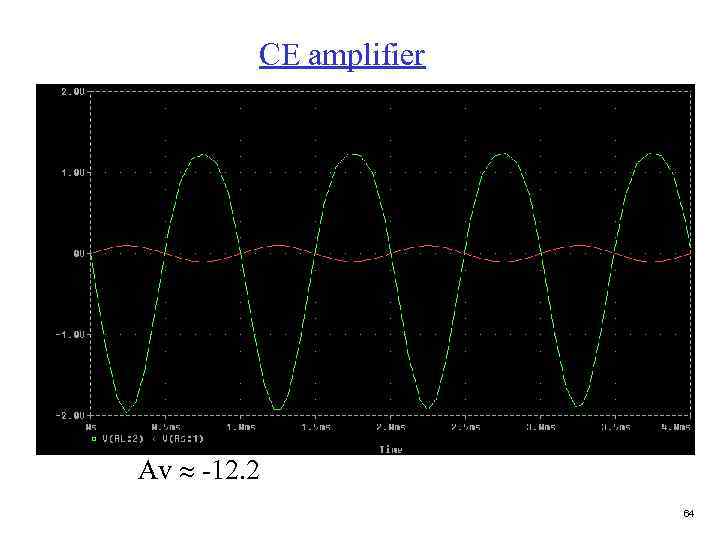
CE amplifier Av -12. 2 64
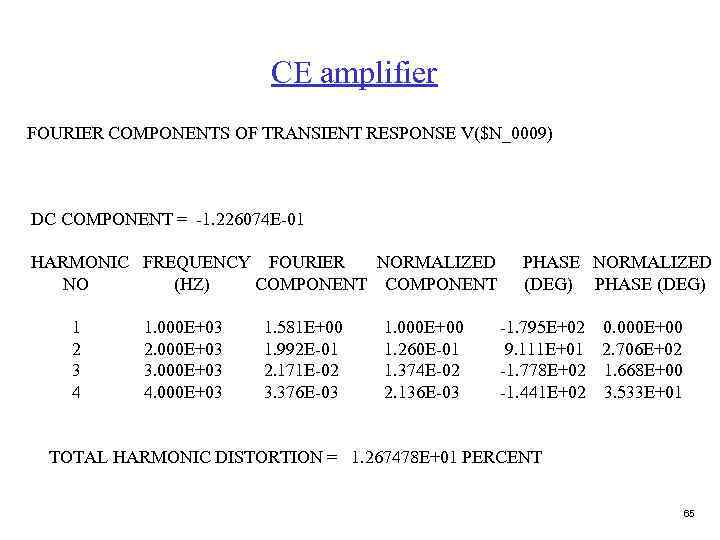
CE amplifier FOURIER COMPONENTS OF TRANSIENT RESPONSE V($N_0009) DC COMPONENT = -1. 226074 E-01 HARMONIC FREQUENCY FOURIER NORMALIZED NO (HZ) COMPONENT 1 2 3 4 1. 000 E+03 2. 000 E+03 3. 000 E+03 4. 000 E+03 1. 581 E+00 1. 992 E-01 2. 171 E-02 3. 376 E-03 1. 000 E+00 1. 260 E-01 1. 374 E-02 2. 136 E-03 PHASE NORMALIZED (DEG) PHASE (DEG) -1. 795 E+02 0. 000 E+00 9. 111 E+01 2. 706 E+02 -1. 778 E+02 1. 668 E+00 -1. 441 E+02 3. 533 E+01 TOTAL HARMONIC DISTORTION = 1. 267478 E+01 PERCENT 65
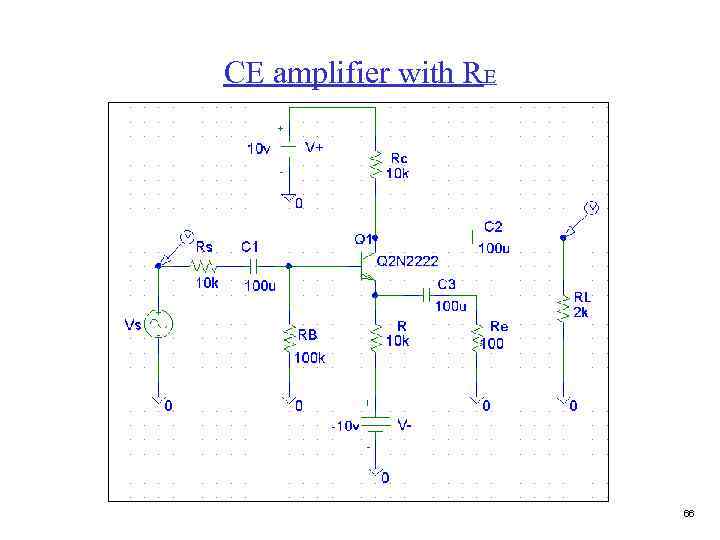
CE amplifier with RE 66
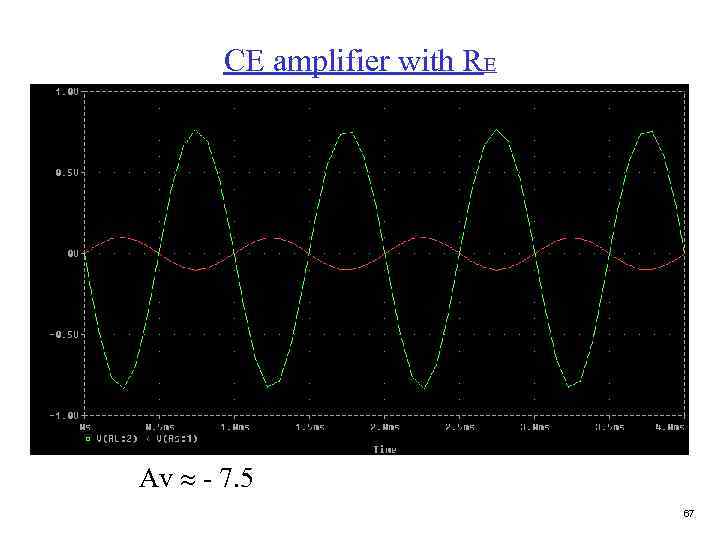
CE amplifier with RE Av - 7. 5 67
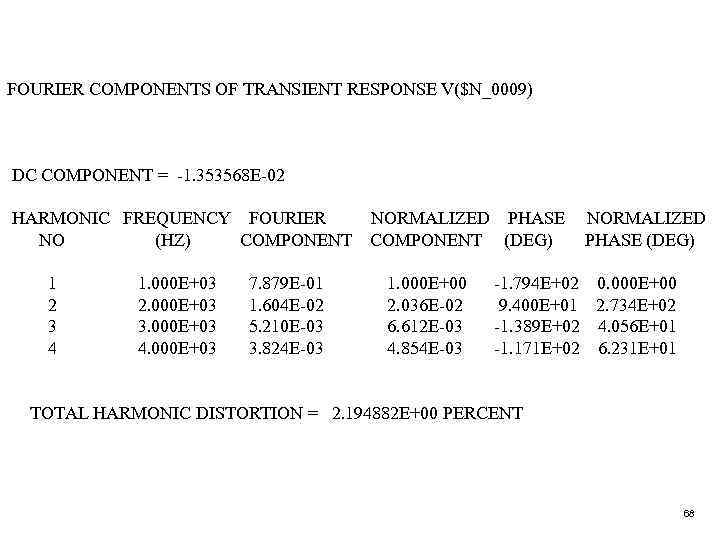
FOURIER COMPONENTS OF TRANSIENT RESPONSE V($N_0009) DC COMPONENT = -1. 353568 E-02 HARMONIC FREQUENCY FOURIER NO (HZ) COMPONENT 1 2 3 4 1. 000 E+03 2. 000 E+03 3. 000 E+03 4. 000 E+03 7. 879 E-01 1. 604 E-02 5. 210 E-03 3. 824 E-03 NORMALIZED PHASE COMPONENT (DEG) 1. 000 E+00 2. 036 E-02 6. 612 E-03 4. 854 E-03 NORMALIZED PHASE (DEG) -1. 794 E+02 0. 000 E+00 9. 400 E+01 2. 734 E+02 -1. 389 E+02 4. 056 E+01 -1. 171 E+02 6. 231 E+01 TOTAL HARMONIC DISTORTION = 2. 194882 E+00 PERCENT 68
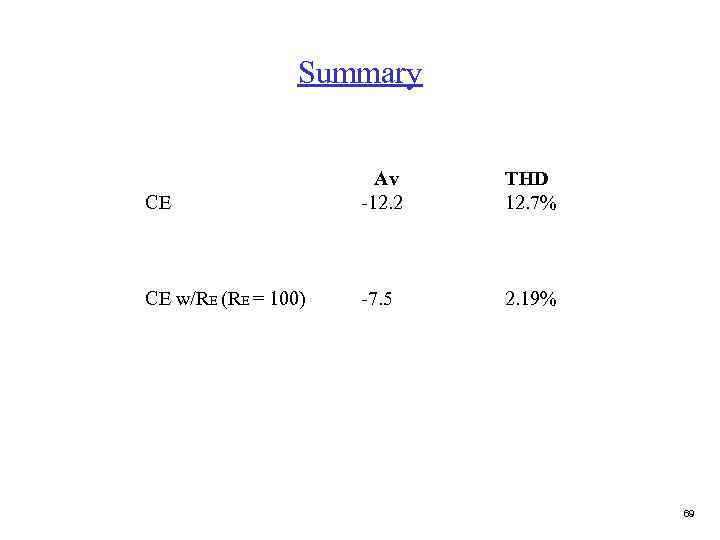
Summary CE Av -12. 2 THD 12. 7% CE w/RE (RE = 100) -7. 5 2. 19% 69
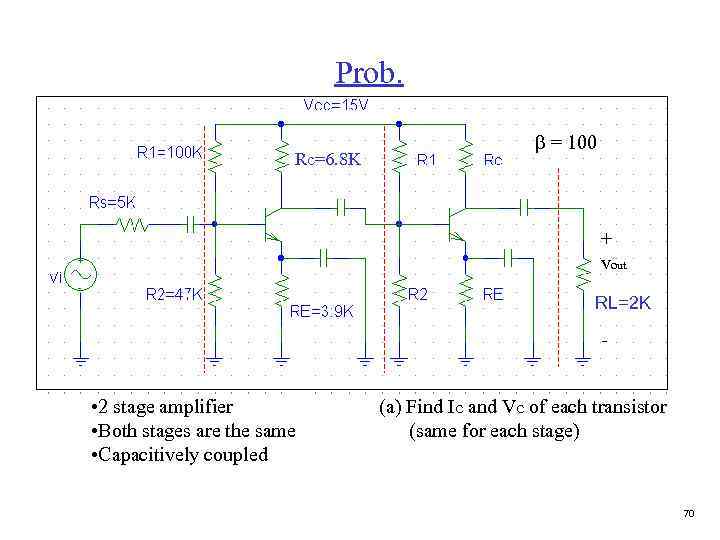
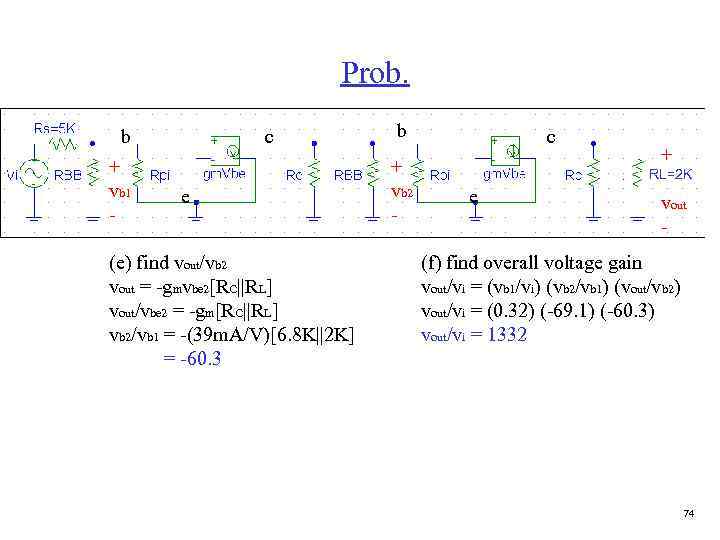
Prob. Rc=6. 8 K b = 100 + vout RL=2 K - • 2 stage amplifier • Both stages are the same • Capacitively coupled (a) Find IC and VC of each transistor (same for each stage) 70
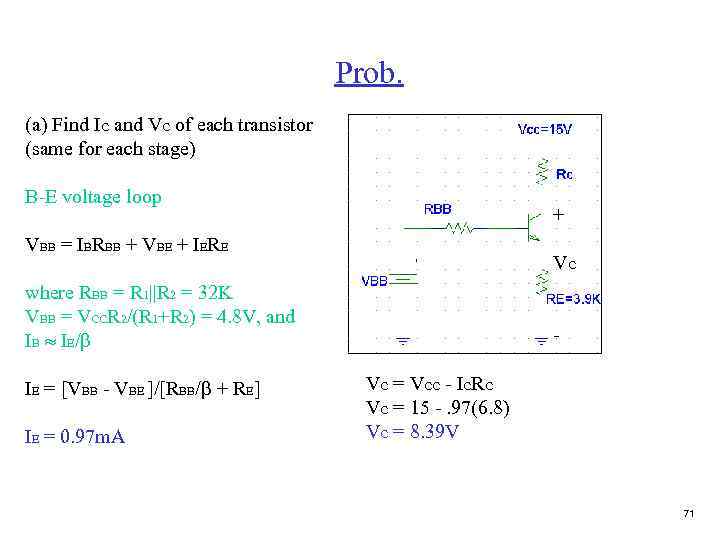
Prob. (a) Find IC and VC of each transistor (same for each stage) B-E voltage loop + VBB = IBRBB + VBE + IERE VC where RBB = R 1||R 2 = 32 K VBB = VCCR 2/(R 1+R 2) = 4. 8 V, and IB IE/b IE = [VBB - VBE ]/[RBB/b + RE] IE = 0. 97 m. A VC = VCC - ICRC VC = 15 -. 97(6. 8) VC = 8. 39 V 71
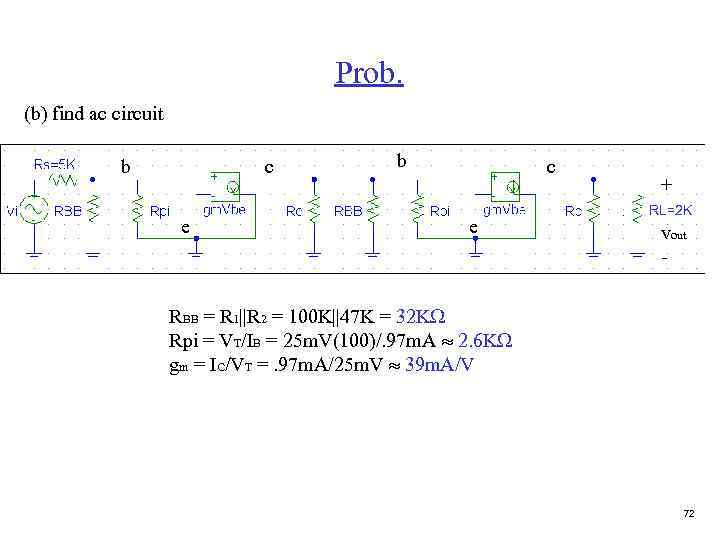
Prob. (b) find ac circuit b c e + RL=2 K vout - RBB = R 1||R 2 = 100 K||47 K = 32 KW Rpi = VT/IB = 25 m. V(100)/. 97 m. A 2. 6 KW gm = IC/VT =. 97 m. A/25 m. V 39 m. A/V 72
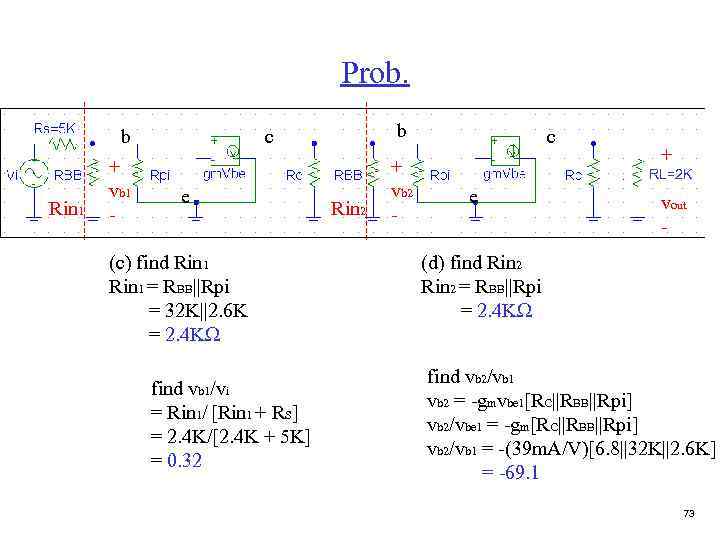
Prob. b Rin 1 + vb 1 - b c e (c) find Rin 1 = RBB||Rpi = 32 K||2. 6 K = 2. 4 KW find vb 1/vi = Rin 1/ [Rin 1 + RS] = 2. 4 K/[2. 4 K + 5 K] = 0. 32 Rin 2 + vb 2 - c + RL=2 K e vout - (d) find Rin 2 = RBB||Rpi = 2. 4 KW find vb 2/vb 1 vb 2 = -gmvbe 1[RC||RBB||Rpi] vb 2/vbe 1 = -gm[RC||RBB||Rpi] vb 2/vb 1 = -(39 m. A/V)[6. 8||32 K||2. 6 K] = -69. 1 73
Prob. b + vb 1 - c e (e) find vout/vb 2 vout = -gmvbe 2[RC||RL] vout/vbe 2 = -gm[RC||RL] vb 2/vb 1 = -(39 m. A/V)[6. 8 K||2 K] = -60. 3 b + vb 2 - c + RL=2 K e vout - (f) find overall voltage gain vout/vi = (vb 1/vi) (vb 2/vb 1) (vout/vb 2) vout/vi = (0. 32) (-69. 1) (-60. 3) vout/vi = 1332 74
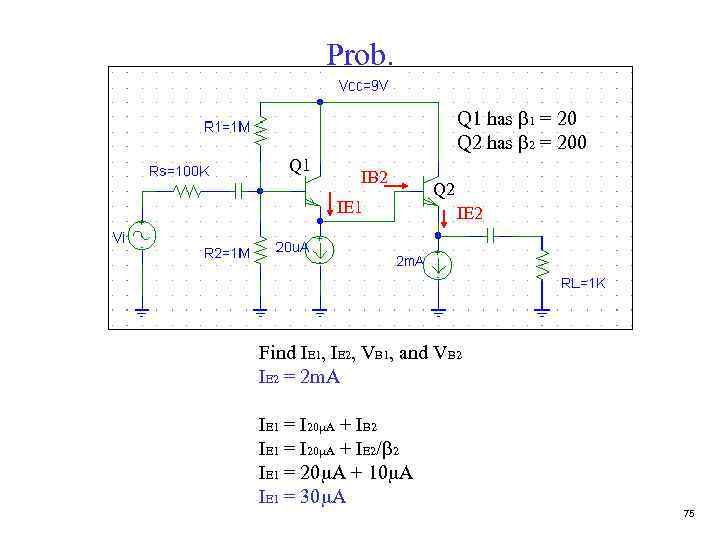
Prob. Q 1 has b 1 = 20 Q 2 has b 2 = 200 Q 1 IB 2 IE 1 Q 2 IE 2 Find IE 1, IE 2, VB 1, and VB 2 IE 2 = 2 m. A IE 1 = I 20 m. A + IB 2 IE 1 = I 20 m. A + IE 2/b 2 IE 1 = 20 m. A + 10 m. A IE 1 = 30 m. A 75
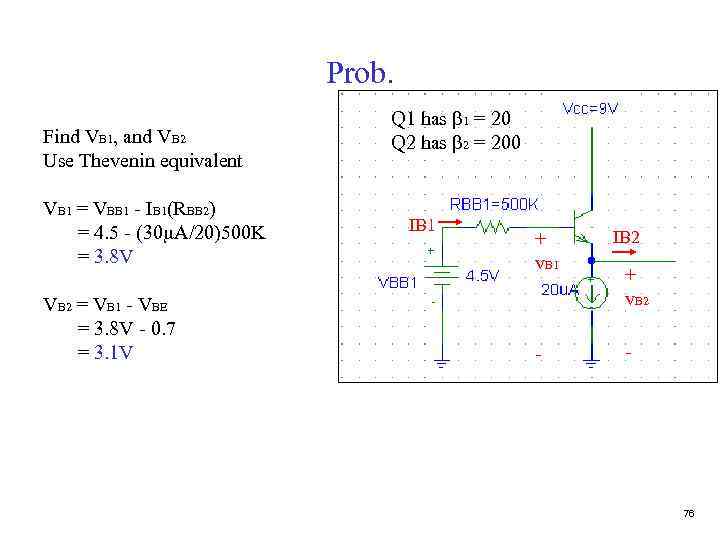
Prob. Find VB 1, and VB 2 Use Thevenin equivalent VB 1 = VBB 1 - IB 1(RBB 2) = 4. 5 - (30 m. A/20)500 K = 3. 8 V VB 2 = VB 1 - VBE = 3. 8 V - 0. 7 = 3. 1 V Q 1 has b 1 = 20 Q 2 has b 2 = 200 IB 1 + v. B 1 - IB 2 + v. B 2 - 76

Prob. b 1 c 1 e 1 + v. B 2 b 2 c 2 e 2 - Rpi 2 = VT/IB 2 = VT b 2/IE 2 = 25 m. V(200)/2 m. A = 2. 5 KW + vout - (b) find vout/vb 2 vout = (ib 2 + b 2 ib 2)RL vb 2 = (ib 2 + b 2 ib 2)RL + ib 2 Rpi 2 vout/vb 2 = (1 + b 2)RL/[(1 + b 2)RL + Rpi 2] = (1 + 200)1 K/[(1 + 200)1 K + 2. 5 K] 0. 988 77
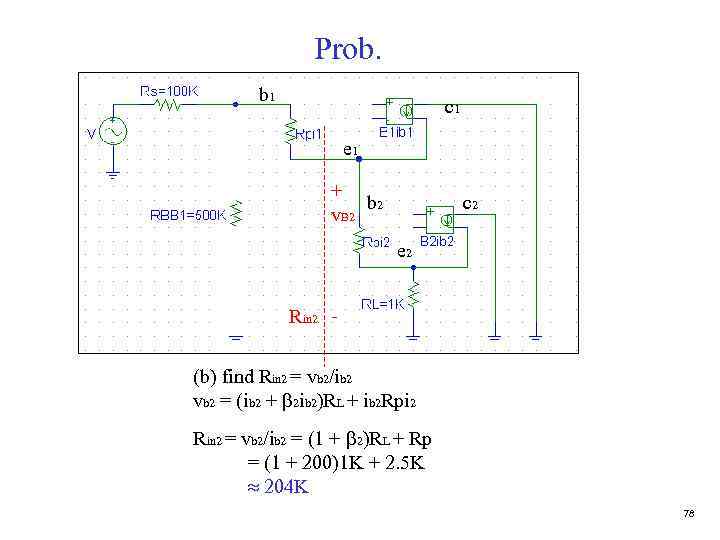
Prob. b 1 c 1 e 1 + b 2 v. B 2 c 2 e 2 Rin 2 (b) find Rin 2 = vb 2/ib 2 vb 2 = (ib 2 + b 2 ib 2)RL + ib 2 Rpi 2 Rin 2 = vb 2/ib 2 = (1 + b 2)RL + Rp = (1 + 200)1 K + 2. 5 K 204 K 78
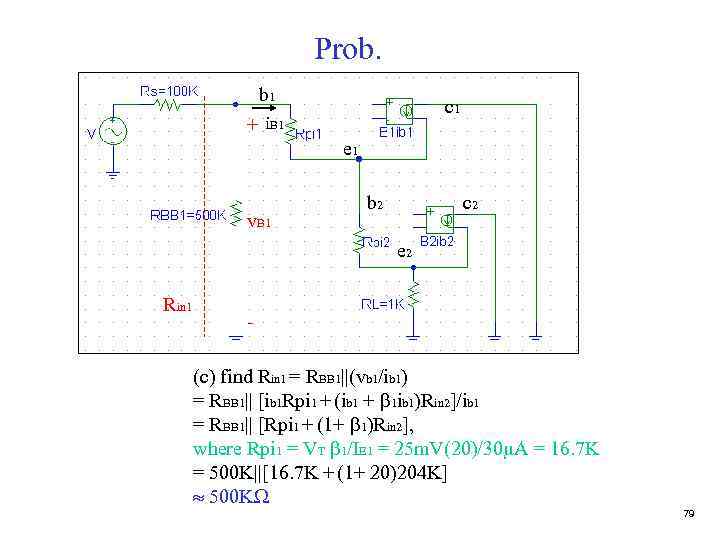
Prob. b 1 c 1 + i. B 1 e 1 v. B 1 b 2 c 2 e 2 Rin 1 (c) find Rin 1 = RBB 1||(vb 1/ib 1) = RBB 1|| [ib 1 Rpi 1 + (ib 1 + b 1 ib 1)Rin 2]/ib 1 = RBB 1|| [Rpi 1 + (1+ b 1)Rin 2], where Rpi 1 = VT b 1/IE 1 = 25 m. V(20)/30 m. A = 16. 7 K = 500 K||[16. 7 K + (1+ 20)204 K] 500 KW 79
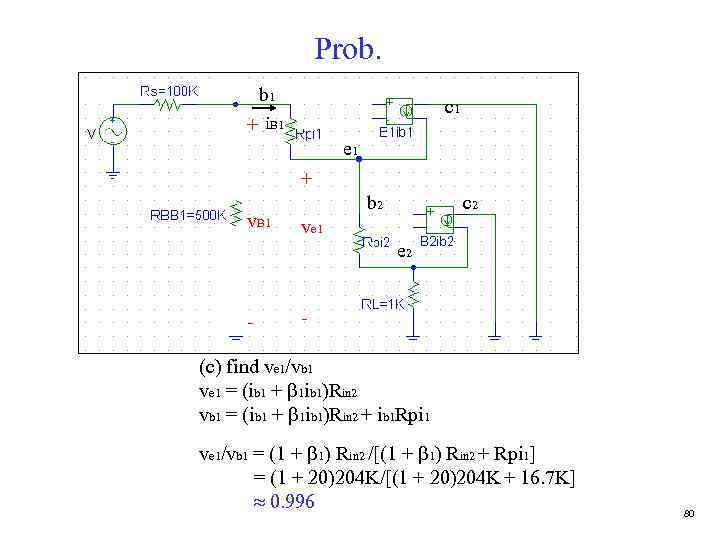
Prob. b 1 c 1 + i. B 1 e 1 + v. B 1 b 2 c 2 ve 1 e 2 - - (c) find ve 1/vb 1 ve 1 = (ib 1 + b 1 ib 1)Rin 2 vb 1 = (ib 1 + b 1 ib 1)Rin 2 + ib 1 Rpi 1 ve 1/vb 1 = (1 + b 1) Rin 2 /[(1 + b 1) Rin 2 + Rpi 1] = (1 + 20)204 K/[(1 + 20)204 K + 16. 7 K] 0. 996 80
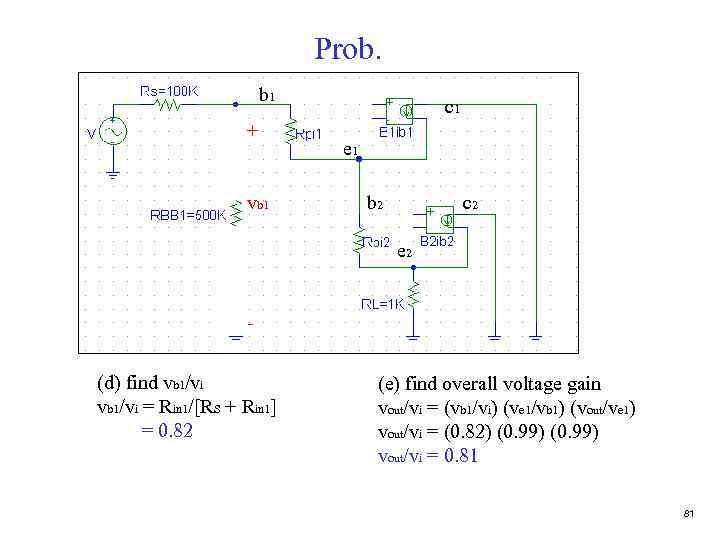
Prob. b 1 + vb 1 c 1 e 1 b 2 c 2 e 2 (d) find vb 1/vi = Rin 1/[RS + Rin 1] = 0. 82 (e) find overall voltage gain vout/vi = (vb 1/vi) (ve 1/vb 1) (vout/ve 1) vout/vi = (0. 82) (0. 99) vout/vi = 0. 81 81

Voltage outputs at each stage Output of stage 2 Output of stage 1 Input 82

Current Input to stage 2 (ib 2) Input current 83
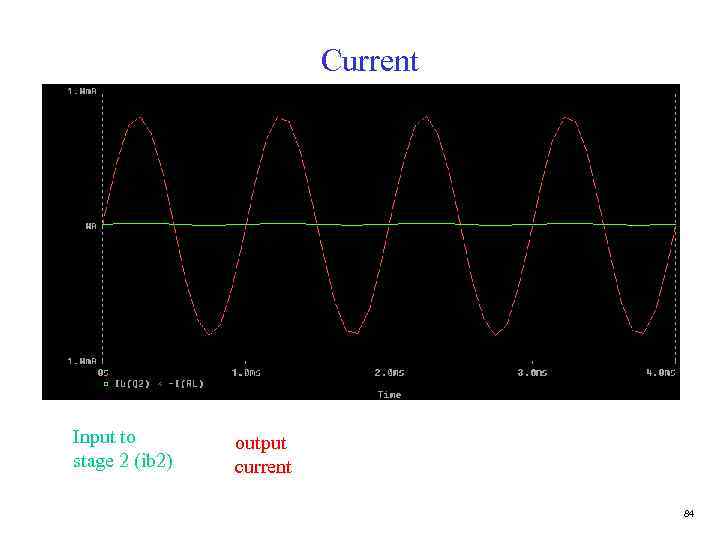
Current Input to stage 2 (ib 2) output current 84
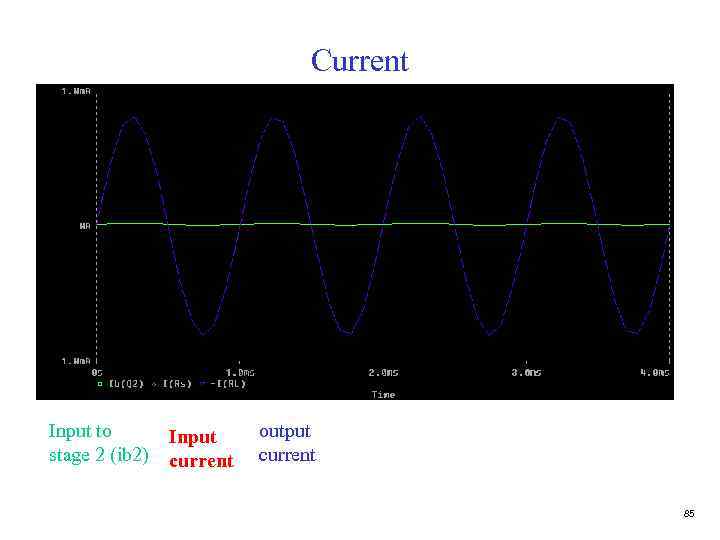
Current Input to stage 2 (ib 2) Input current output current 85

Power and current gain Input current = (Vi)/Rin = 1/500 K = 2. 0 m. A output current= (Vout)/RL = (0. 81 V)/1 K= 0. 81 m. A current gain = 0. 81 m. A/ 2. 0 m. A = 405 Input power = (Vi)/Rin = 1 x 1/500 K = 2. 0 m. W output power = (Vout)/RL = (0. 81 V)/1 K= 656 m. W power gain = 656 m. W/2 m. W = 329 86
BJT Output Characteristics • Plot Ic vs. Vce for multiple values of Vce and Ib • From Analysis menu use DC Sweep • Use Nested sweep in DC Sweep section 87
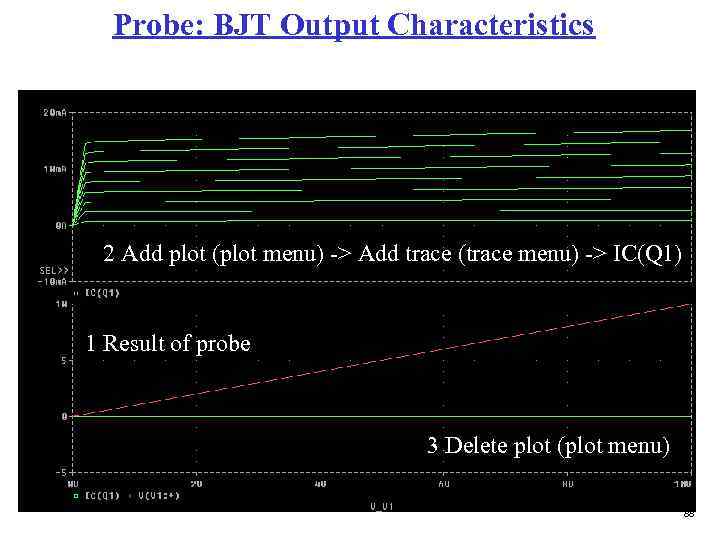
Probe: BJT Output Characteristics 2 Add plot (plot menu) -> Add trace (trace menu) -> IC(Q 1) 1 Result of probe 3 Delete plot (plot menu) 88
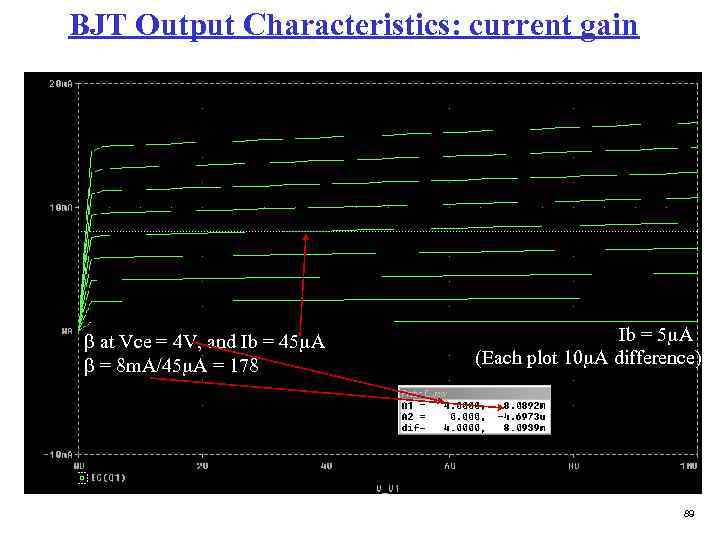
BJT Output Characteristics: current gain b at Vce = 4 V, and Ib = 45 m. A b = 8 m. A/45 m. A = 178 Ib = 5 m. A (Each plot 10 m. A difference) 89
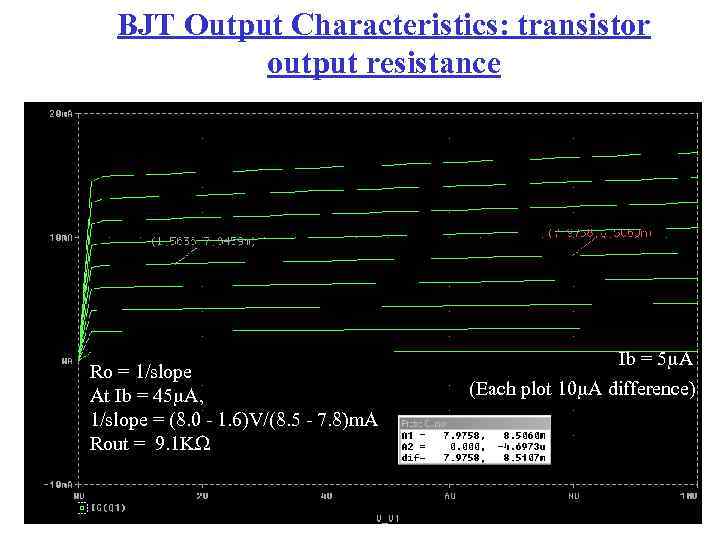
BJT Output Characteristics: transistor output resistance Ro = 1/slope At Ib = 45 m. A, 1/slope = (8. 0 - 1. 6)V/(8. 5 - 7. 8)m. A Rout = 9. 1 KW Ib = 5 m. A (Each plot 10 m. A difference) 90
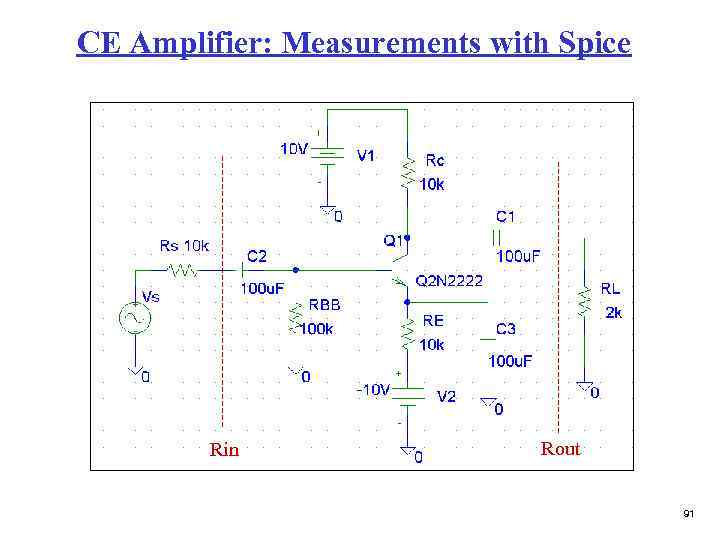
CE Amplifier: Measurements with Spice Rin Rout 91

Input Resistance Measurement Using SPICE • Replace source, Vs and Rs with Vin, measure Rin = Vin/Iin • Do not change DC problem: keep capacitive coupling if present • Source (Vin) should be a high enough frequency so that capacitors act as shorts: Rcap = |1/w. C|. For C = 100 m. F, w = 1 KHz, Rcap = 1/2 p(1 K)(100 E-6) 1. 6 W • Vin should have a small value so operating point does not change Vin 1 m. V 92
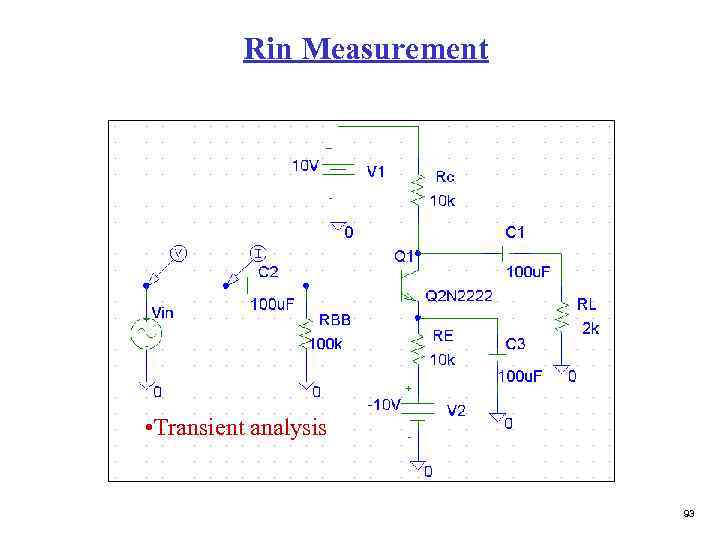
Rin Measurement • Transient analysis 93
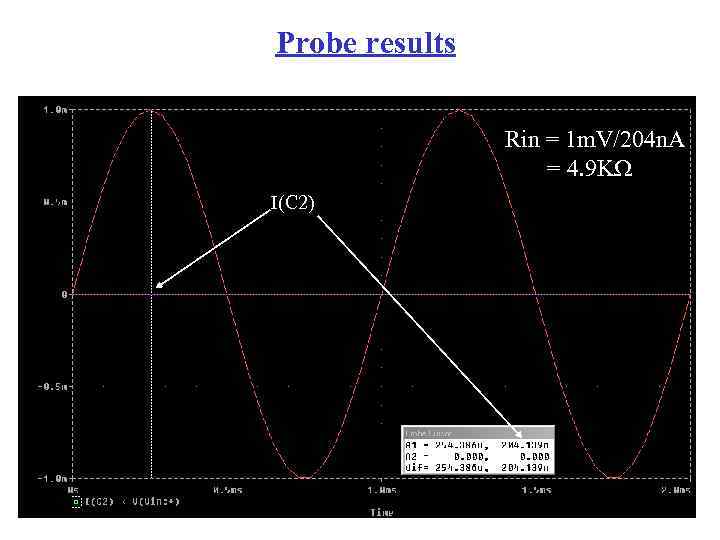
Probe results Rin = 1 m. V/204 n. A = 4. 9 KW I(C 2) 94

Output Resistance Measurement Using SPICE • Replace load, RL with Vin, measure Rin = Vin/Iin • Set Vs = 0 • Do not change DC problem: keep capacitive coupling if present • Source (Vin) should be a high enough frequency so that capacitors act as shorts: Rcap = |1/w. C|. For C = 100 m. F, w = 1 KHz, Rcap = 1/2 p(1 K)(100 E-6) 1. 6 W • Vin should have a small value so operating point does not change Vin 1 m. V 95
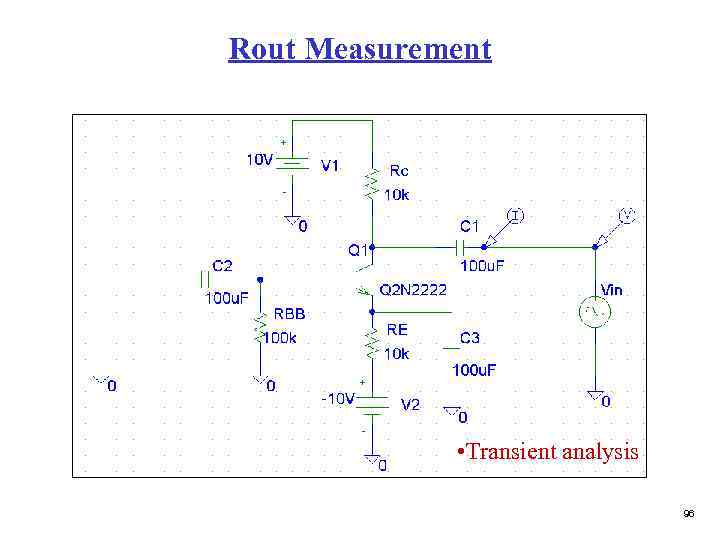
Rout Measurement • Transient analysis 96
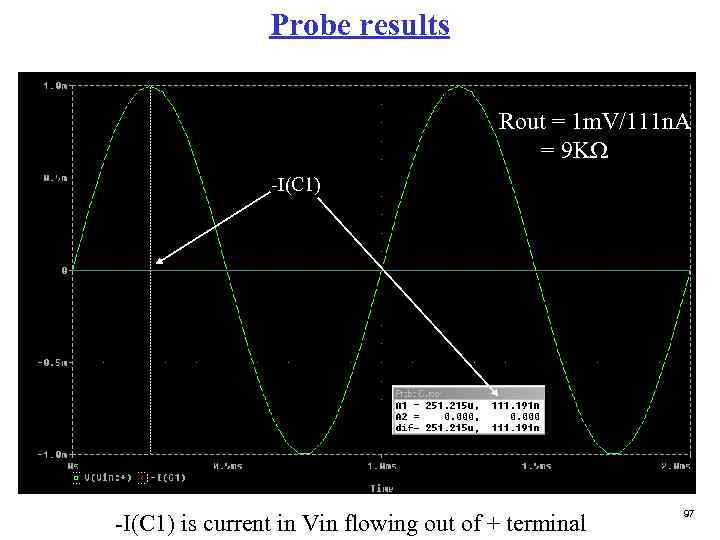
Probe results Rout = 1 m. V/111 n. A = 9 KW -I(C 1) is current in Vin flowing out of + terminal 97
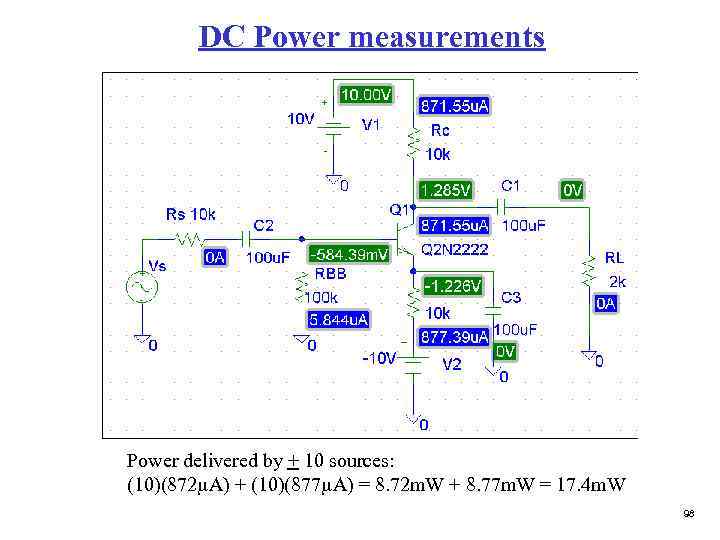
DC Power measurements Power delivered by + 10 sources: (10)(872 m. A) + (10)(877 m. A) = 8. 72 m. W + 8. 77 m. W = 17. 4 m. W 98
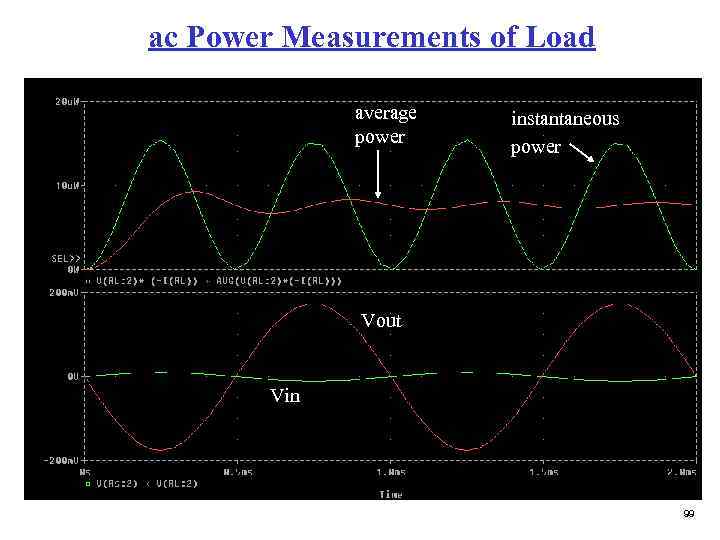
ac Power Measurements of Load average power instantaneous power Vout Vin 99
BJT-Transistors-Class+note.ppt