e264909ed4c610c229de7f819f5b8f66.ppt
- Количество слайдов: 45
 An Introduction to X-Analysis Integration (XAI) Part 4: Advanced Topics & Current Research Georgia Tech Engineering Information Systems Lab eislab. gatech. edu Contact: Russell S. Peak Revision: March 15, 2001 Copyright © 1993 -2001 by Georgia Tech Research Corporation, Atlanta, Georgia 30332 -0415 USA. All Rights Reserved. Developed by eislab. gatech. edu. Permission to use for non-commercial purposes is hereby granted provided this notice is included.
An Introduction to X-Analysis Integration (XAI) Part 4: Advanced Topics & Current Research Georgia Tech Engineering Information Systems Lab eislab. gatech. edu Contact: Russell S. Peak Revision: March 15, 2001 Copyright © 1993 -2001 by Georgia Tech Research Corporation, Atlanta, Georgia 30332 -0415 USA. All Rights Reserved. Developed by eislab. gatech. edu. Permission to use for non-commercial purposes is hereby granted provided this notice is included.
 An Introduction to X-Analysis Integration (XAI) Short Course Outline Part 1: Constrained Objects (COBs) Primer – Nomenclature Part 2: Multi-Representation Architecture (MRA) Primer – Analysis Integration Challenges – Overview of COB-based XAI – Ubiquitization Methodology Part 3: Example Applications » Airframe Structural Analysis » Circuit Board Thermomechanical Analysis » Chip Package Thermal Analysis – Summary Part 4: Advanced Topics & Current Research © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 2
An Introduction to X-Analysis Integration (XAI) Short Course Outline Part 1: Constrained Objects (COBs) Primer – Nomenclature Part 2: Multi-Representation Architecture (MRA) Primer – Analysis Integration Challenges – Overview of COB-based XAI – Ubiquitization Methodology Part 3: Example Applications » Airframe Structural Analysis » Circuit Board Thermomechanical Analysis » Chip Package Thermal Analysis – Summary Part 4: Advanced Topics & Current Research © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 2
 Advanced Topics & Current Research Outline Advanced Product Information-Driven FEA Modeling – Focus on cases with: » Variable topology multi-body geometries » Different design & analysis geometries » Mixed analytical bodies and idealized interfaces Constrained Object (COB) Extensions – Automating support for multiple views – Next-generation capabilities Optimization and the MRA © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 3
Advanced Topics & Current Research Outline Advanced Product Information-Driven FEA Modeling – Focus on cases with: » Variable topology multi-body geometries » Different design & analysis geometries » Mixed analytical bodies and idealized interfaces Constrained Object (COB) Extensions – Automating support for multiple views – Next-generation capabilities Optimization and the MRA © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 3
 Variable Topology Multi-Body (VTMB) FEA Meshing Challenges Analytical Bodies Labor-intensive “chopping” FEA Model Decomposed Volumes 1 a 1 b 1 2 1 c 2 3 a 3 3 b 3 c original 1 a 1 b 1 c 2 1 d 1 e 3 a 3 b 1 2 3 topology change (no body change) 1 a 2 3 1 4 © 1993 -2001 GTRC variable body change (includes topology change) 1 b 2 3 1 c 1 d 4 a 4 b Engineering Information Systems Lab eislab. gatech. edu 4 c 4
Variable Topology Multi-Body (VTMB) FEA Meshing Challenges Analytical Bodies Labor-intensive “chopping” FEA Model Decomposed Volumes 1 a 1 b 1 2 1 c 2 3 a 3 3 b 3 c original 1 a 1 b 1 c 2 1 d 1 e 3 a 3 b 1 2 3 topology change (no body change) 1 a 2 3 1 4 © 1993 -2001 GTRC variable body change (includes topology change) 1 b 2 3 1 c 1 d 4 a 4 b Engineering Information Systems Lab eislab. gatech. edu 4 c 4
![Product Information-Driven FEA Methodology Purpose of VTMB Methodology [Gen. 1 - Koo, 2000] thermal Product Information-Driven FEA Methodology Purpose of VTMB Methodology [Gen. 1 - Koo, 2000] thermal](https://present5.com/presentation/e264909ed4c610c229de7f819f5b8f66/image-5.jpg) Product Information-Driven FEA Methodology Purpose of VTMB Methodology [Gen. 1 - Koo, 2000] thermal stress CBAMs PWB APMs VTMB Methodology Chip package APMs VTMB FEA Models thermal resistance CBAMs create algorithmij once algorithmij use algorithmij many times ANSYS SMMs Design Instances Analysis Instances Design Types i = 1…m Analysis Types j = 1…n for a given ij j {1…n} (not all design types have all analysis types) e. g. ) for i=1(EBGA), j=1(thermal resistance) j=2 (thermal stress) for i=2 (PWB), j=1 (warpage) VTMB= variable topology multi-body © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 5
Product Information-Driven FEA Methodology Purpose of VTMB Methodology [Gen. 1 - Koo, 2000] thermal stress CBAMs PWB APMs VTMB Methodology Chip package APMs VTMB FEA Models thermal resistance CBAMs create algorithmij once algorithmij use algorithmij many times ANSYS SMMs Design Instances Analysis Instances Design Types i = 1…m Analysis Types j = 1…n for a given ij j {1…n} (not all design types have all analysis types) e. g. ) for i=1(EBGA), j=1(thermal resistance) j=2 (thermal stress) for i=2 (PWB), j=1 (warpage) VTMB= variable topology multi-body © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 5
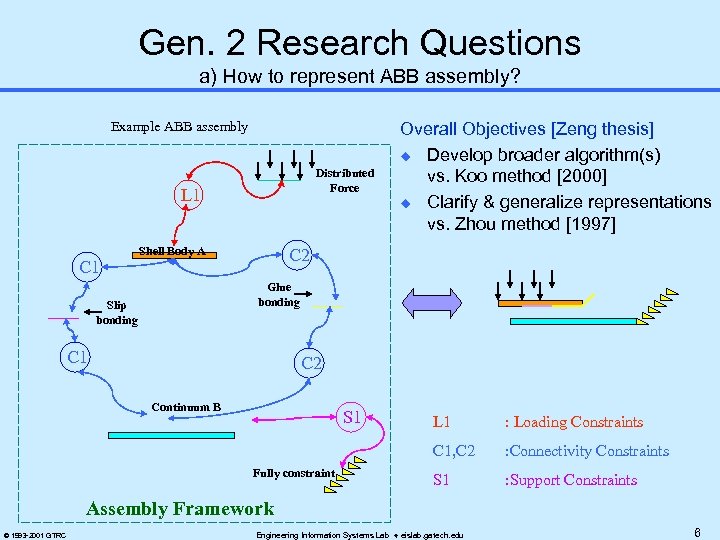 Gen. 2 Research Questions a) How to represent ABB assembly? Example ABB assembly Distributed Force L 1 C 1 Shell Body A Overall Objectives [Zeng thesis] u Develop broader algorithm(s) vs. Koo method [2000] u Clarify & generalize representations vs. Zhou method [1997] C 2 Glue bonding Slip bonding C 1 C 2 Continuum B S 1 : Loading Constraints C 1, C 2 Fully constraint L 1 : Connectivity Constraints S 1 : Support Constraints Assembly Framework © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 6
Gen. 2 Research Questions a) How to represent ABB assembly? Example ABB assembly Distributed Force L 1 C 1 Shell Body A Overall Objectives [Zeng thesis] u Develop broader algorithm(s) vs. Koo method [2000] u Clarify & generalize representations vs. Zhou method [1997] C 2 Glue bonding Slip bonding C 1 C 2 Continuum B S 1 : Loading Constraints C 1, C 2 Fully constraint L 1 : Connectivity Constraints S 1 : Support Constraints Assembly Framework © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 6
 Research Questions b) How represent Preprocessor Solution Method Model (PSMM)? (FEA model specific) ABB assembly view © 1993 -2001 GTRC ABB assembly view combined with ANSYSspecific consideration Engineering Information Systems Lab eislab. gatech. edu 7
Research Questions b) How represent Preprocessor Solution Method Model (PSMM)? (FEA model specific) ABB assembly view © 1993 -2001 GTRC ABB assembly view combined with ANSYSspecific consideration Engineering Information Systems Lab eislab. gatech. edu 7
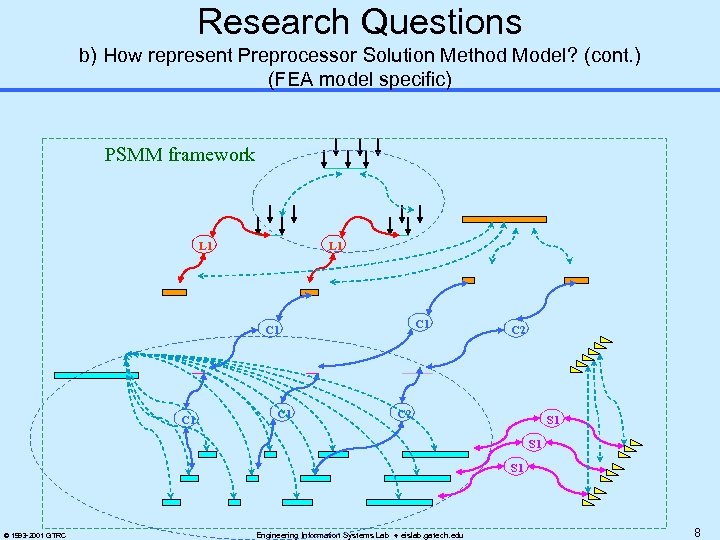 Research Questions b) How represent Preprocessor Solution Method Model? (cont. ) (FEA model specific) PSMM framework L 1 C 1 C 1 C 2 S 1 S 1 © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 8
Research Questions b) How represent Preprocessor Solution Method Model? (cont. ) (FEA model specific) PSMM framework L 1 C 1 C 1 C 2 S 1 S 1 © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 8
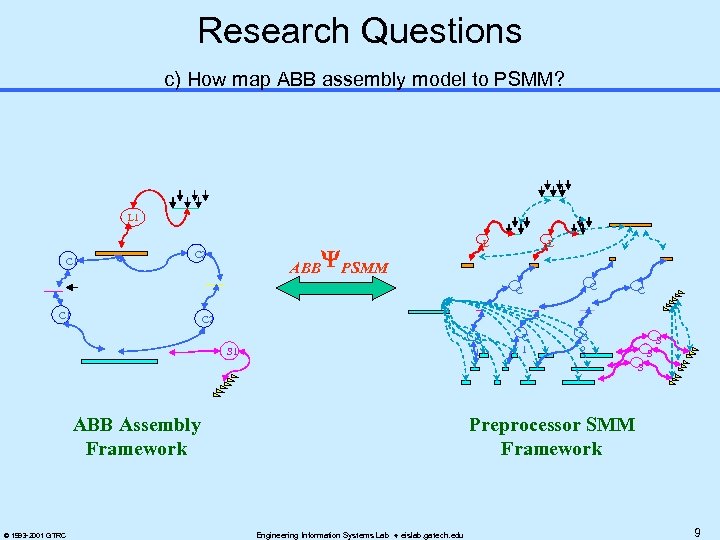 Research Questions c) How map ABB assembly model to PSMM? L 1 C 1 L ABB PSMM C 2 L C C C 1 C C 2 C 1 S 1 C 2 S S S ABB Assembly Framework © 1993 -2001 GTRC Preprocessor SMM Framework Engineering Information Systems Lab eislab. gatech. edu 9
Research Questions c) How map ABB assembly model to PSMM? L 1 C 1 L ABB PSMM C 2 L C C C 1 C C 2 C 1 S 1 C 2 S S S ABB Assembly Framework © 1993 -2001 GTRC Preprocessor SMM Framework Engineering Information Systems Lab eislab. gatech. edu 9
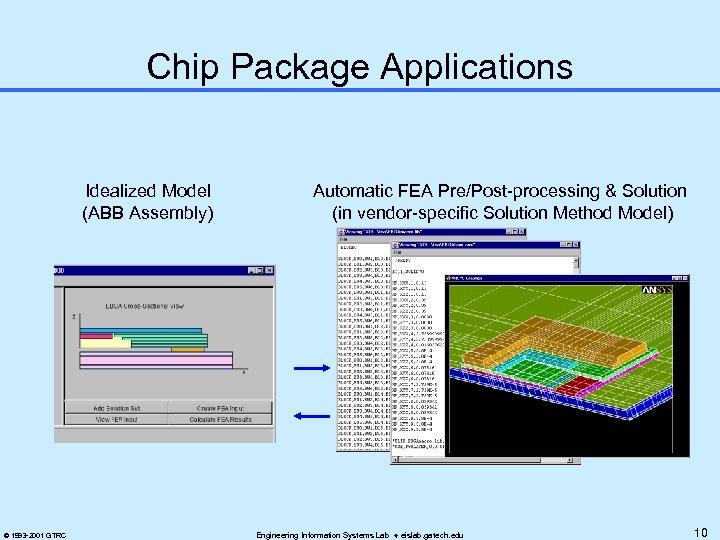 Chip Package Applications Idealized Model (ABB Assembly) © 1993 -2001 GTRC Automatic FEA Pre/Post-processing & Solution (in vendor-specific Solution Method Model) Engineering Information Systems Lab eislab. gatech. edu 10
Chip Package Applications Idealized Model (ABB Assembly) © 1993 -2001 GTRC Automatic FEA Pre/Post-processing & Solution (in vendor-specific Solution Method Model) Engineering Information Systems Lab eislab. gatech. edu 10
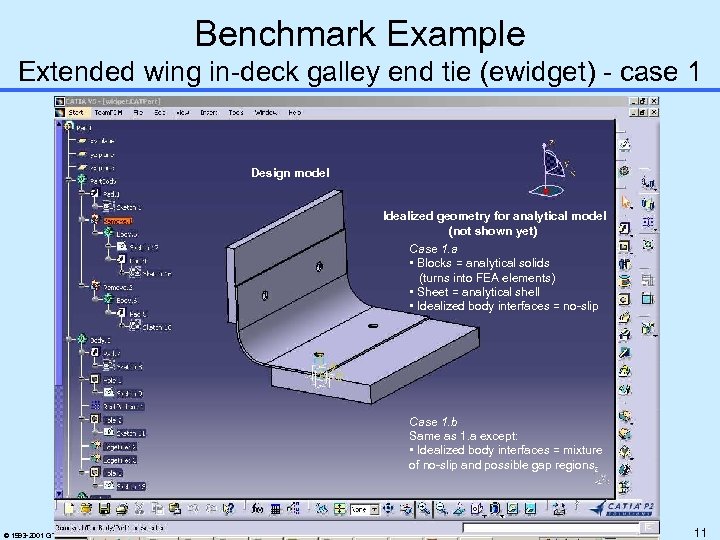 Benchmark Example Extended wing in-deck galley end tie (ewidget) - case 1 Design model Idealized geometry for analytical model (not shown yet) Case 1. a • Blocks = analytical solids (turns into FEA elements) • Sheet = analytical shell • Idealized body interfaces = no-slip Case 1. b Same as 1. a except: • Idealized body interfaces = mixture of no-slip and possible gap regions © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 11
Benchmark Example Extended wing in-deck galley end tie (ewidget) - case 1 Design model Idealized geometry for analytical model (not shown yet) Case 1. a • Blocks = analytical solids (turns into FEA elements) • Sheet = analytical shell • Idealized body interfaces = no-slip Case 1. b Same as 1. a except: • Idealized body interfaces = mixture of no-slip and possible gap regions © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 11
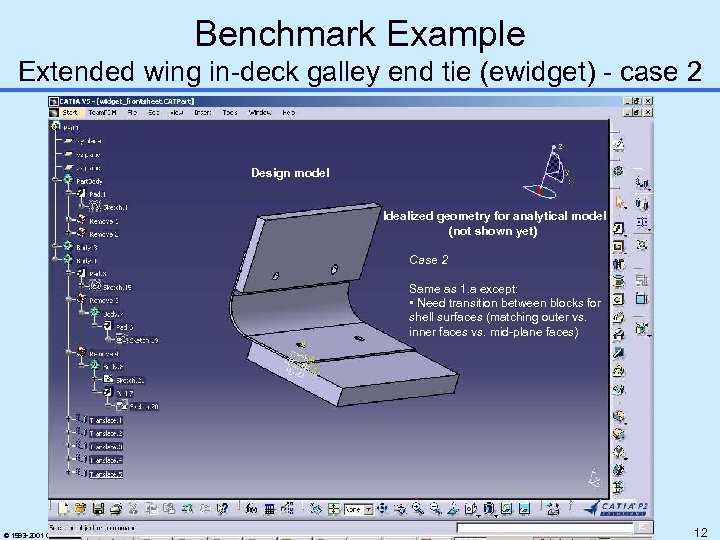 Benchmark Example Extended wing in-deck galley end tie (ewidget) - case 2 Design model Idealized geometry for analytical model (not shown yet) Case 2 Same as 1. a except: • Need transition between blocks for shell surfaces (matching outer vs. inner faces vs. mid-plane faces) © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 12
Benchmark Example Extended wing in-deck galley end tie (ewidget) - case 2 Design model Idealized geometry for analytical model (not shown yet) Case 2 Same as 1. a except: • Need transition between blocks for shell surfaces (matching outer vs. inner faces vs. mid-plane faces) © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 12
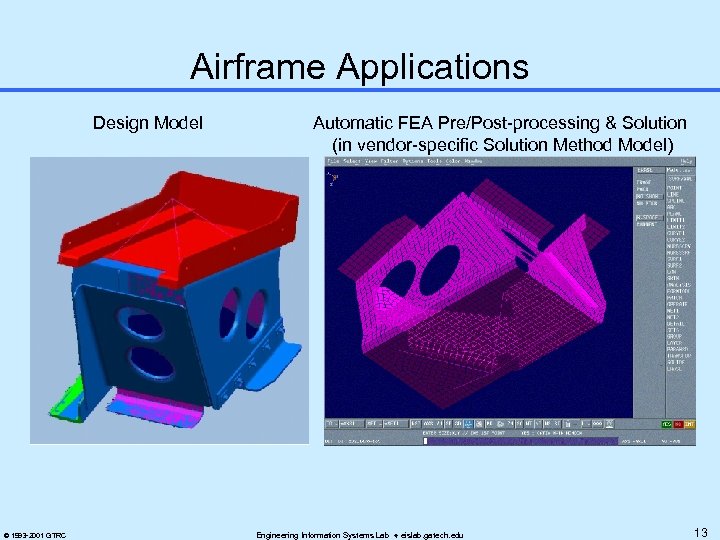 Airframe Applications Design Model © 1993 -2001 GTRC Automatic FEA Pre/Post-processing & Solution (in vendor-specific Solution Method Model) Engineering Information Systems Lab eislab. gatech. edu 13
Airframe Applications Design Model © 1993 -2001 GTRC Automatic FEA Pre/Post-processing & Solution (in vendor-specific Solution Method Model) Engineering Information Systems Lab eislab. gatech. edu 13
 Status: Advanced Product Info-Driven FEA Modeling u u u Building on previous work Ph. D thesis proposal underway [Zeng] Target applications identified & work underway: – Chip package thermal analysis (Shinko) – Airframe structural analysis (Boeing) © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 14
Status: Advanced Product Info-Driven FEA Modeling u u u Building on previous work Ph. D thesis proposal underway [Zeng] Target applications identified & work underway: – Chip package thermal analysis (Shinko) – Airframe structural analysis (Boeing) © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 14
 Advanced Topics & Current Research Outline Advanced Product Information-Driven FEA Modeling Constrained Object (COB) Extensions – Automating support for multiple views – Next-generation capabilities Optimization and the MRA © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 15
Advanced Topics & Current Research Outline Advanced Product Information-Driven FEA Modeling Constrained Object (COB) Extensions – Automating support for multiple views – Next-generation capabilities Optimization and the MRA © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 15
 Constrained Object (COB) Representation Current Technical Capabilities - Generation 2 u Capabilities & features: – – u Various forms: computable lexical forms, graphical forms, etc. Sub/supertypes, basic aggregates, multi-fidelity objects Multi-directionality (I/O change) Wrapping external programs as white box relations Analysis module/template applications: – – Product model idealizations Explicit associativity relations with design models & other analyses White box reuse of existing tools (e. g. , FEA, in-house codes) Reusable, adaptable analysis building blocks – Synthesis (sizing) and verification (analysis) © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 16
Constrained Object (COB) Representation Current Technical Capabilities - Generation 2 u Capabilities & features: – – u Various forms: computable lexical forms, graphical forms, etc. Sub/supertypes, basic aggregates, multi-fidelity objects Multi-directionality (I/O change) Wrapping external programs as white box relations Analysis module/template applications: – – Product model idealizations Explicit associativity relations with design models & other analyses White box reuse of existing tools (e. g. , FEA, in-house codes) Reusable, adaptable analysis building blocks – Synthesis (sizing) and verification (analysis) © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 16
 Constrained Objects (cont. ) Representation Characteristics & Advantages - Gen. 2 u Overall characteristics – Declarative knowledge representation (non-causal) – Combining object & constraint graph techniques – COBs = (STEP EXPRESS subset) + (constraint graph concepts & views) u Advantages over traditional analysis representations – Greater solution control – Richer semantics (e. g. , equations wrapped in engineering context) – Capture of reusable knowledge – Enhanced development of complex analysis models u Toolkit status (Xai. Tools v 0. 4) – Basic framework, single user-oriented, file-based © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 17
Constrained Objects (cont. ) Representation Characteristics & Advantages - Gen. 2 u Overall characteristics – Declarative knowledge representation (non-causal) – Combining object & constraint graph techniques – COBs = (STEP EXPRESS subset) + (constraint graph concepts & views) u Advantages over traditional analysis representations – Greater solution control – Richer semantics (e. g. , equations wrapped in engineering context) – Capture of reusable knowledge – Enhanced development of complex analysis models u Toolkit status (Xai. Tools v 0. 4) – Basic framework, single user-oriented, file-based © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 17
 Planned Generation 3 + COB Enhancements u u u Use standard forms: Express v 3, STEP Parametrics, XML, UML OCL, … Leverage standard content: STEP generic resources, APs, . . . Support concurrent multiple users (block points/buffering, synchronization, …) Enable interactive COS and COI construction Provide variety of interaction views/forms: – – © 1993 -2001 GTRC textual/graphical geometric/logical definition/solution/documentation traditional (e. g. , classical equation form) Engineering Information Systems Lab eislab. gatech. edu 18
Planned Generation 3 + COB Enhancements u u u Use standard forms: Express v 3, STEP Parametrics, XML, UML OCL, … Leverage standard content: STEP generic resources, APs, . . . Support concurrent multiple users (block points/buffering, synchronization, …) Enable interactive COS and COI construction Provide variety of interaction views/forms: – – © 1993 -2001 GTRC textual/graphical geometric/logical definition/solution/documentation traditional (e. g. , classical equation form) Engineering Information Systems Lab eislab. gatech. edu 18
 Interaction Views/Forms u u u u information structure navigation template/instance Each textual/graphical form has its n geometric/logical iche definition/solution/documentation traditional (e. g. , classical equation form) native CAD/CAE tool specialized application view Novice Users: Graphical forms and specialized applications Expert Users: All forms © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 19
Interaction Views/Forms u u u u information structure navigation template/instance Each textual/graphical form has its n geometric/logical iche definition/solution/documentation traditional (e. g. , classical equation form) native CAD/CAE tool specialized application view Novice Users: Graphical forms and specialized applications Expert Users: All forms © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 19
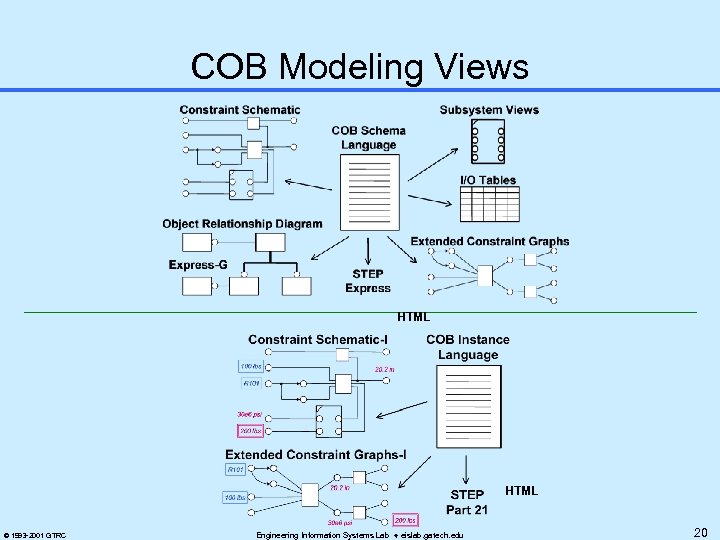 COB Modeling Views HTML © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 20
COB Modeling Views HTML © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 20
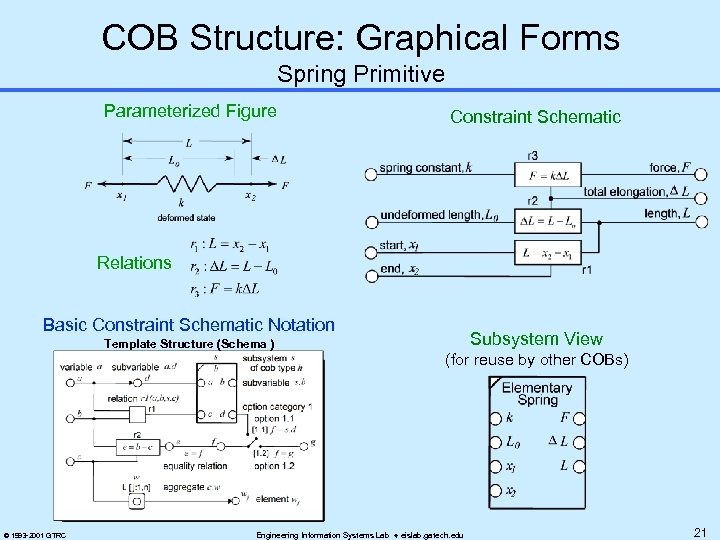 COB Structure: Graphical Forms Spring Primitive Parameterized Figure Constraint Schematic Relations Basic Constraint Schematic Notation Subsystem View Template Structure (Schema ) (for reuse by other COBs) © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 21
COB Structure: Graphical Forms Spring Primitive Parameterized Figure Constraint Schematic Relations Basic Constraint Schematic Notation Subsystem View Template Structure (Schema ) (for reuse by other COBs) © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 21
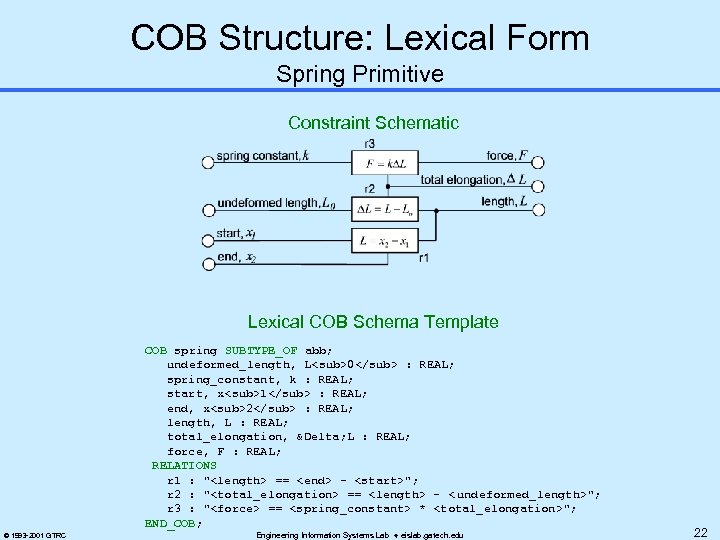 COB Structure: Lexical Form Spring Primitive Constraint Schematic Lexical COB Schema Template COB spring SUBTYPE_OF abb; undeformed_length, L0 : REAL; spring_constant, k : REAL; start, x1 : REAL; end, x2 : REAL; length, L : REAL; total_elongation, Δ L : REAL; force, F : REAL; RELATIONS r 1 : "
COB Structure: Lexical Form Spring Primitive Constraint Schematic Lexical COB Schema Template COB spring SUBTYPE_OF abb; undeformed_length, L0 : REAL; spring_constant, k : REAL; start, x1 : REAL; end, x2 : REAL; length, L : REAL; total_elongation, Δ L : REAL; force, F : REAL; RELATIONS r 1 : "
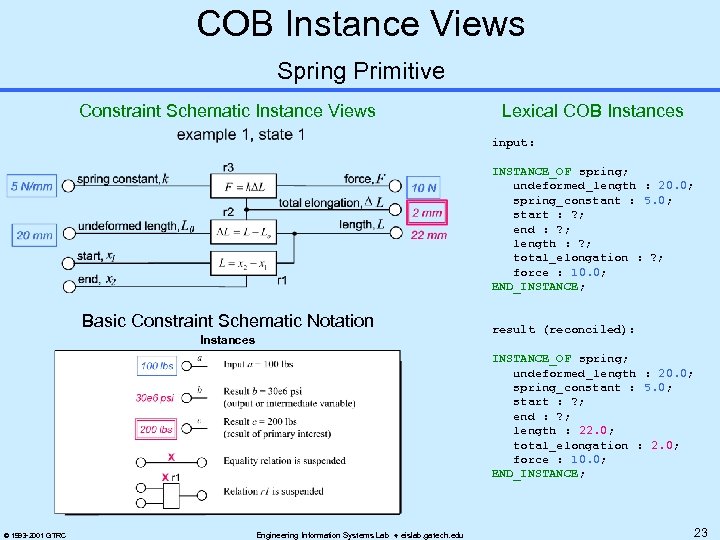 COB Instance Views Spring Primitive Constraint Schematic Instance Views Lexical COB Instances input: INSTANCE_OF spring; undeformed_length : 20. 0; spring_constant : 5. 0; start : ? ; end : ? ; length : ? ; total_elongation : ? ; force : 10. 0; END_INSTANCE; Basic Constraint Schematic Notation Instances result (reconciled): INSTANCE_OF spring; undeformed_length : 20. 0; spring_constant : 5. 0; start : ? ; end : ? ; length : 22. 0; total_elongation : 2. 0; force : 10. 0; END_INSTANCE; © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 23
COB Instance Views Spring Primitive Constraint Schematic Instance Views Lexical COB Instances input: INSTANCE_OF spring; undeformed_length : 20. 0; spring_constant : 5. 0; start : ? ; end : ? ; length : ? ; total_elongation : ? ; force : 10. 0; END_INSTANCE; Basic Constraint Schematic Notation Instances result (reconciled): INSTANCE_OF spring; undeformed_length : 20. 0; spring_constant : 5. 0; start : ? ; end : ? ; length : 22. 0; total_elongation : 2. 0; force : 10. 0; END_INSTANCE; © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 23
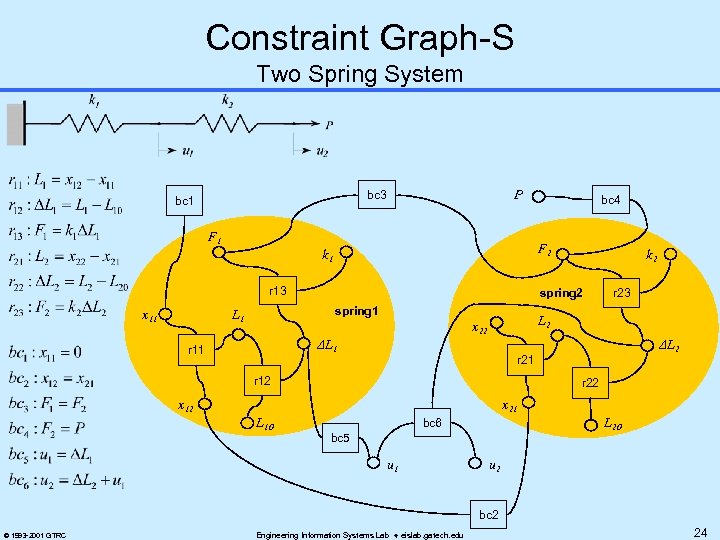 Constraint Graph-S Two Spring System P bc 3 bc 1 F 2 k 1 r 13 x 11 r 23 L 2 x 22 L 1 r 11 k 2 spring 1 L 1 bc 4 L 2 r 21 r 12 r 22 x 12 x 21 L 10 L 20 bc 6 bc 5 u 1 u 2 bc 2 © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 24
Constraint Graph-S Two Spring System P bc 3 bc 1 F 2 k 1 r 13 x 11 r 23 L 2 x 22 L 1 r 11 k 2 spring 1 L 1 bc 4 L 2 r 21 r 12 r 22 x 12 x 21 L 10 L 20 bc 6 bc 5 u 1 u 2 bc 2 © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 24
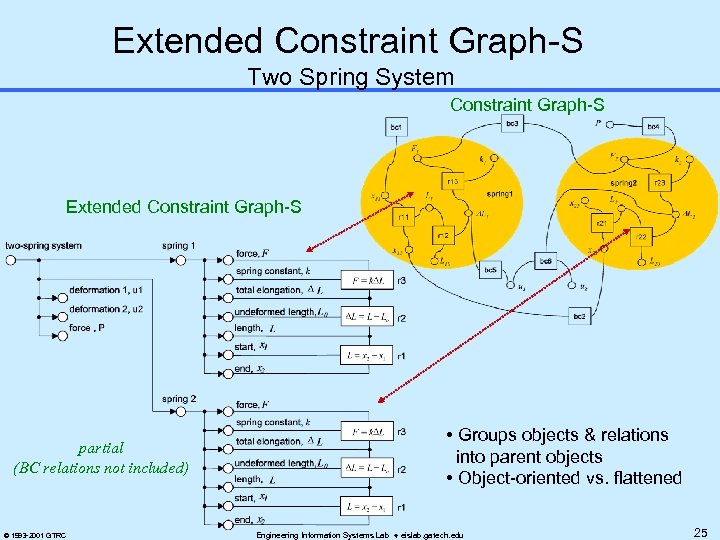 Extended Constraint Graph-S Two Spring System Constraint Graph-S Extended Constraint Graph-S partial (BC relations not included) © 1993 -2001 GTRC • Groups objects & relations into parent objects • Object-oriented vs. flattened Engineering Information Systems Lab eislab. gatech. edu 25
Extended Constraint Graph-S Two Spring System Constraint Graph-S Extended Constraint Graph-S partial (BC relations not included) © 1993 -2001 GTRC • Groups objects & relations into parent objects • Object-oriented vs. flattened Engineering Information Systems Lab eislab. gatech. edu 25
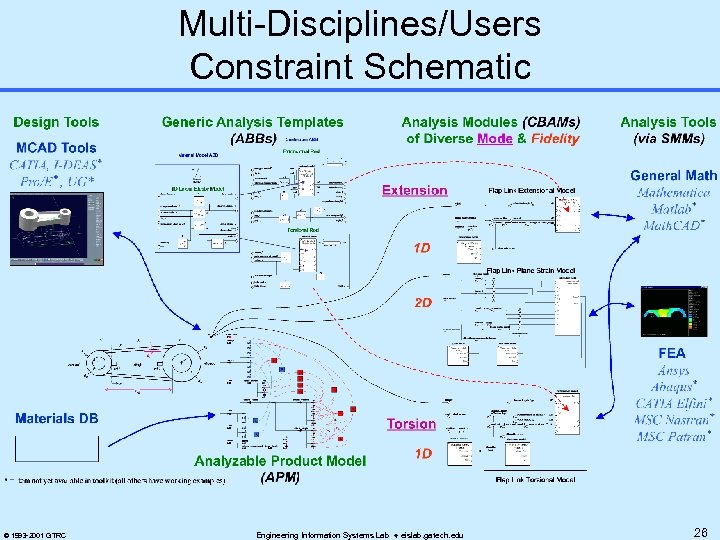 Multi-Disciplines/Users Constraint Schematic © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 26
Multi-Disciplines/Users Constraint Schematic © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 26
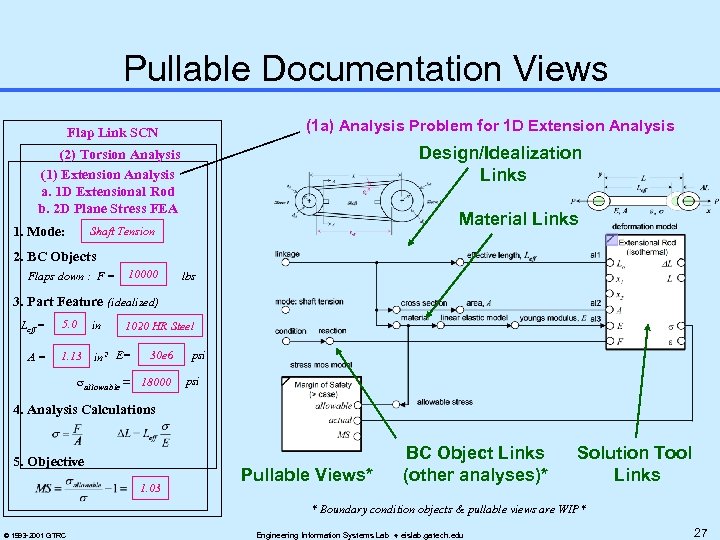 Pullable Documentation Views (1 a) Analysis Problem for 1 D Extension Analysis Flap Link SCN Design/Idealization Links (2) Torsion Analysis (1) Extension Analysis a. 1 D Extensional Rod b. 2 D Plane Stress FEA 1. Mode: Material Links Shaft Tension 2. BC Objects Flaps down : F = 10000 lbs 3. Part Feature (idealized) Leff = A= 5. 0 in 1. 13 in 2 E= 1020 HR Steel 30 e 6 allowable = 18000 psi 4. Analysis Calculations 5. Objective 1. 03 Pullable Views* BC Object Links (other analyses)* Solution Tool Links * Boundary condition objects & pullable views are WIP* © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 27
Pullable Documentation Views (1 a) Analysis Problem for 1 D Extension Analysis Flap Link SCN Design/Idealization Links (2) Torsion Analysis (1) Extension Analysis a. 1 D Extensional Rod b. 2 D Plane Stress FEA 1. Mode: Material Links Shaft Tension 2. BC Objects Flaps down : F = 10000 lbs 3. Part Feature (idealized) Leff = A= 5. 0 in 1. 13 in 2 E= 1020 HR Steel 30 e 6 allowable = 18000 psi 4. Analysis Calculations 5. Objective 1. 03 Pullable Views* BC Object Links (other analyses)* Solution Tool Links * Boundary condition objects & pullable views are WIP* © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 27
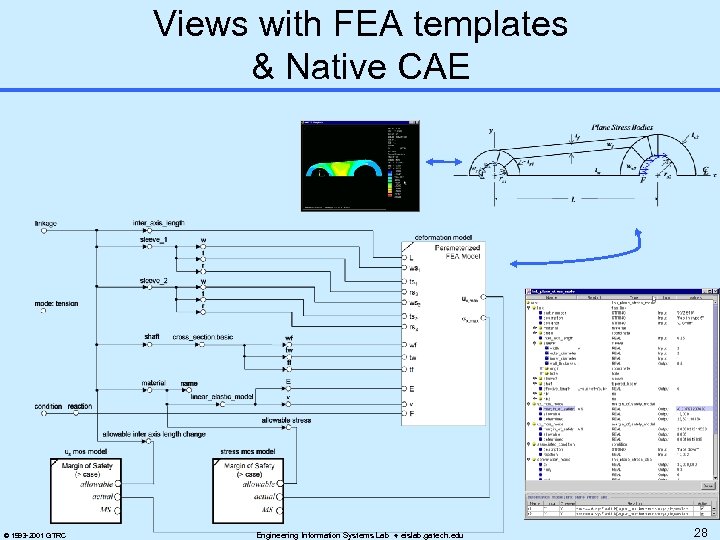 Views with FEA templates & Native CAE © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 28
Views with FEA templates & Native CAE © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 28
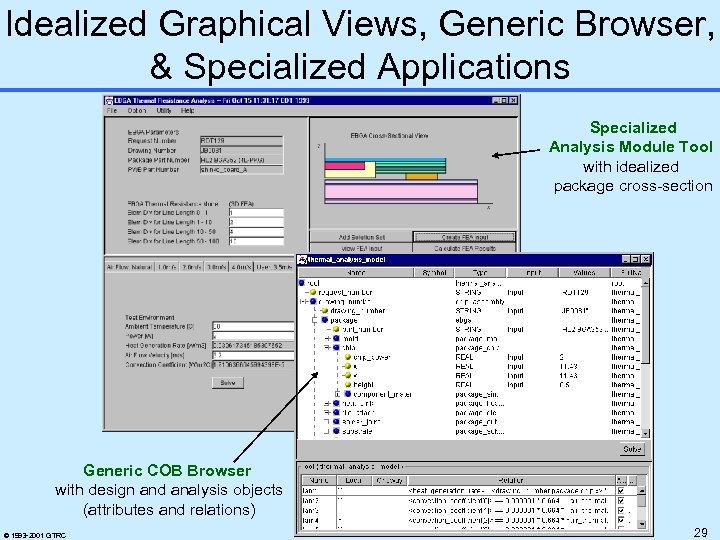 Idealized Graphical Views, Generic Browser, & Specialized Applications Specialized Analysis Module Tool with idealized package cross-section Generic COB Browser with design and analysis objects (attributes and relations) © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 29
Idealized Graphical Views, Generic Browser, & Specialized Applications Specialized Analysis Module Tool with idealized package cross-section Generic COB Browser with design and analysis objects (attributes and relations) © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 29
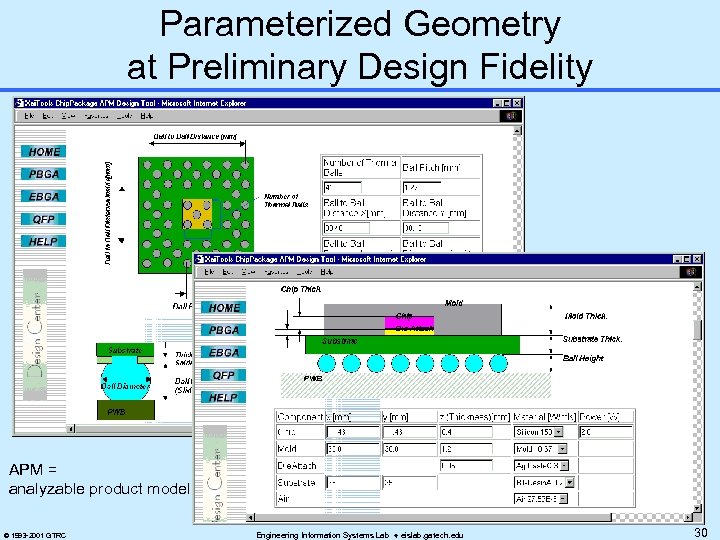 Parameterized Geometry at Preliminary Design Fidelity APM = analyzable product model © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 30
Parameterized Geometry at Preliminary Design Fidelity APM = analyzable product model © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 30
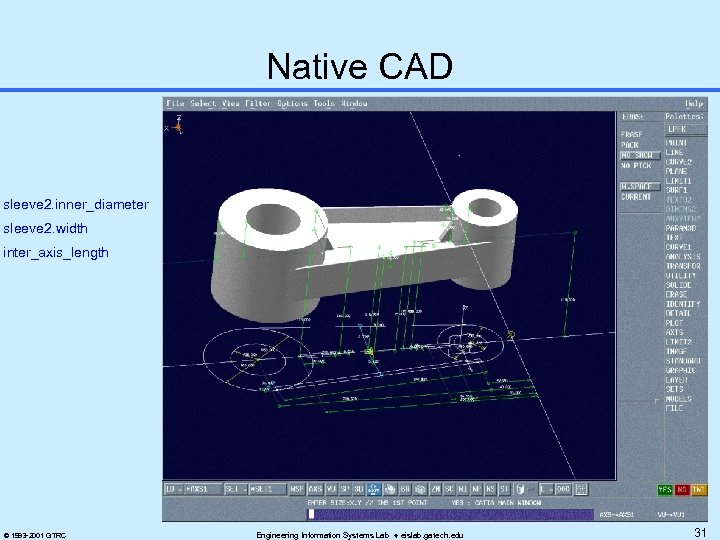 Native CAD sleeve 2. inner_diameter sleeve 2. width inter_axis_length © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 31
Native CAD sleeve 2. inner_diameter sleeve 2. width inter_axis_length © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 31
 Planned Generation 3 + Other COB Enhancements u u u Support units and automatic conversions Extend COI language capabilities Improve constraint graph algorithms – Support structural loops – Support multiple subsolvers (for specified subgraphs) u u u Enable hybrid declarative/procedural approaches Allow constraint hierarchies (i. e. , relations with variable satisfaction priorities) Support enhanced relations Support explicit COS categories (e. g. , APMs, CBAMs, ABBs) Versioning & configuration management of structure © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 32
Planned Generation 3 + Other COB Enhancements u u u Support units and automatic conversions Extend COI language capabilities Improve constraint graph algorithms – Support structural loops – Support multiple subsolvers (for specified subgraphs) u u u Enable hybrid declarative/procedural approaches Allow constraint hierarchies (i. e. , relations with variable satisfaction priorities) Support enhanced relations Support explicit COS categories (e. g. , APMs, CBAMs, ABBs) Versioning & configuration management of structure © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 32
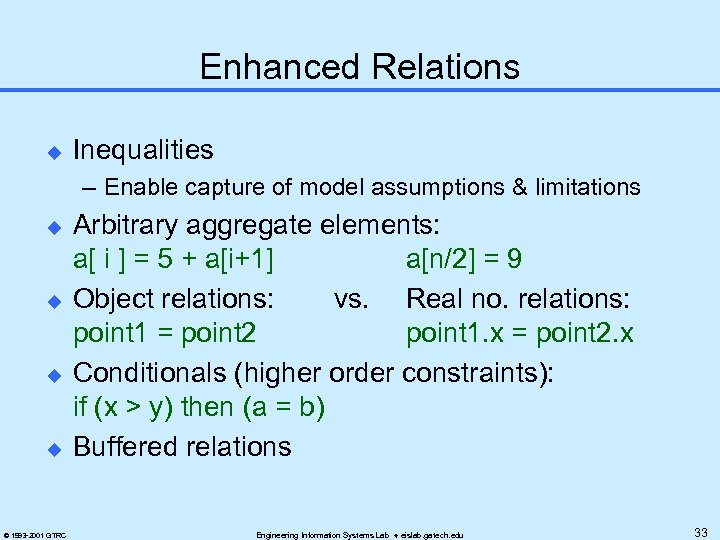 Enhanced Relations u Inequalities – Enable capture of model assumptions & limitations u u © 1993 -2001 GTRC Arbitrary aggregate elements: a[ i ] = 5 + a[i+1] a[n/2] = 9 Object relations: vs. Real no. relations: point 1 = point 2 point 1. x = point 2. x Conditionals (higher order constraints): if (x > y) then (a = b) Buffered relations Engineering Information Systems Lab eislab. gatech. edu 33
Enhanced Relations u Inequalities – Enable capture of model assumptions & limitations u u © 1993 -2001 GTRC Arbitrary aggregate elements: a[ i ] = 5 + a[i+1] a[n/2] = 9 Object relations: vs. Real no. relations: point 1 = point 2 point 1. x = point 2. x Conditionals (higher order constraints): if (x > y) then (a = b) Buffered relations Engineering Information Systems Lab eislab. gatech. edu 33
 Status: Next Gen. COBs and Views u u u © 1993 -2001 GTRC Building on previous work Needs and anticipated approaches identified Seeking extension opportunities Engineering Information Systems Lab eislab. gatech. edu 34
Status: Next Gen. COBs and Views u u u © 1993 -2001 GTRC Building on previous work Needs and anticipated approaches identified Seeking extension opportunities Engineering Information Systems Lab eislab. gatech. edu 34
 Advanced Topics & Current Research Outline Advanced Product Information-Driven FEA Modeling Constrained Object (COB) Extensions – Automating support for multiple views – Next-generation capabilities Optimization and the MRA © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 35
Advanced Topics & Current Research Outline Advanced Product Information-Driven FEA Modeling Constrained Object (COB) Extensions – Automating support for multiple views – Next-generation capabilities Optimization and the MRA © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 35
 Thesis Abstract © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 36
Thesis Abstract © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 36
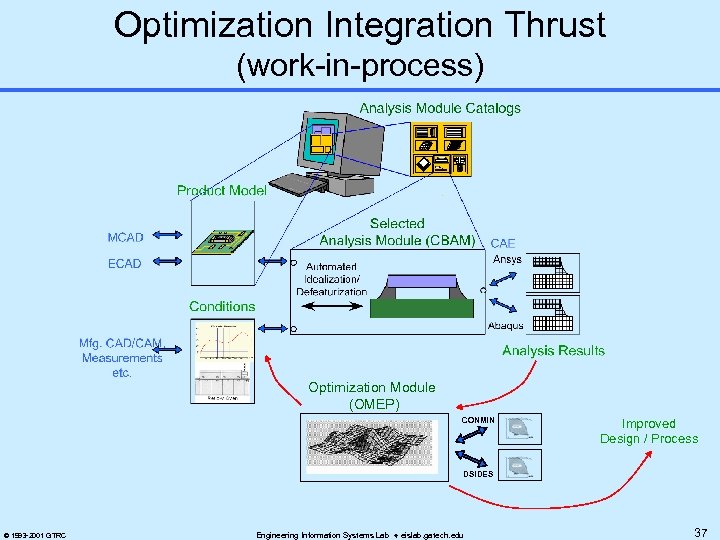 Optimization Integration Thrust (work-in-process) Optimization Module (OMEP) CONMIN Improved Design / Process DSIDES © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 37
Optimization Integration Thrust (work-in-process) Optimization Module (OMEP) CONMIN Improved Design / Process DSIDES © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 37
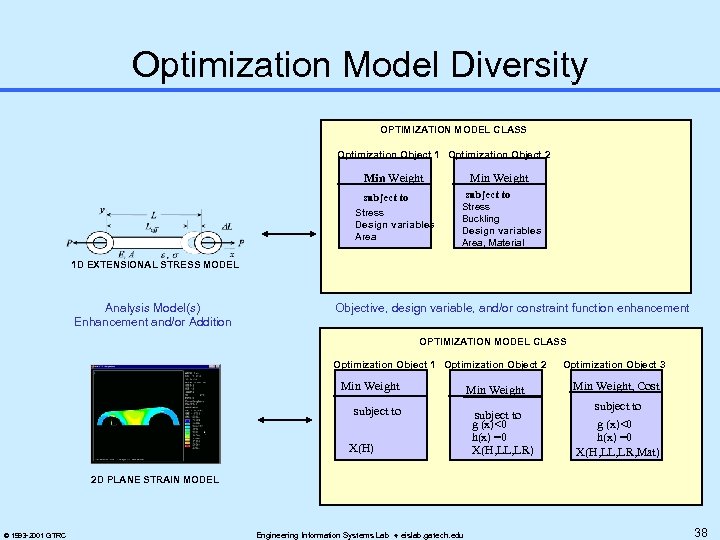 Optimization Model Diversity OPTIMIZATION MODEL CLASS Optimization Object 1 Optimization Object 2 Min Weight subject to Stress Design variables Area Stress Buckling Design variables Area, Material 1 D EXTENSIONAL STRESS MODEL Analysis Model(s) Enhancement and/or Addition Objective, design variable, and/or constraint function enhancement OPTIMIZATION MODEL CLASS Optimization Object 1 Optimization Object 2 Min Weight subject to g (x)<0 h(x) =0 X(H) Min Weight subject to g (x)<0 h(x) =0 X(H, LL, LR) Optimization Object 3 Min Weight, Cost subject to g (x)<0 h(x) =0 X(H, LL, LR, Mat) 2 D PLANE STRAIN MODEL © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 38
Optimization Model Diversity OPTIMIZATION MODEL CLASS Optimization Object 1 Optimization Object 2 Min Weight subject to Stress Design variables Area Stress Buckling Design variables Area, Material 1 D EXTENSIONAL STRESS MODEL Analysis Model(s) Enhancement and/or Addition Objective, design variable, and/or constraint function enhancement OPTIMIZATION MODEL CLASS Optimization Object 1 Optimization Object 2 Min Weight subject to g (x)<0 h(x) =0 X(H) Min Weight subject to g (x)<0 h(x) =0 X(H, LL, LR) Optimization Object 3 Min Weight, Cost subject to g (x)<0 h(x) =0 X(H, LL, LR, Mat) 2 D PLANE STRAIN MODEL © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 38
 Optimization Model Enhancement OPTIMIZATION MODEL I Minimize f 1 = r. LA Weight Subject to g 1 = MS (A) ³ 0 stress Normal Stress Margin of Safety Design variables X={A} Minimize OPTIMIZATION MODEL II f 1 = r. LA Weight Subject to g 1 = MS (A) ³ 0 stress Normal Stress Margin of Safety Design variables X={A, material} © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 39
Optimization Model Enhancement OPTIMIZATION MODEL I Minimize f 1 = r. LA Weight Subject to g 1 = MS (A) ³ 0 stress Normal Stress Margin of Safety Design variables X={A} Minimize OPTIMIZATION MODEL II f 1 = r. LA Weight Subject to g 1 = MS (A) ³ 0 stress Normal Stress Margin of Safety Design variables X={A, material} © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 39
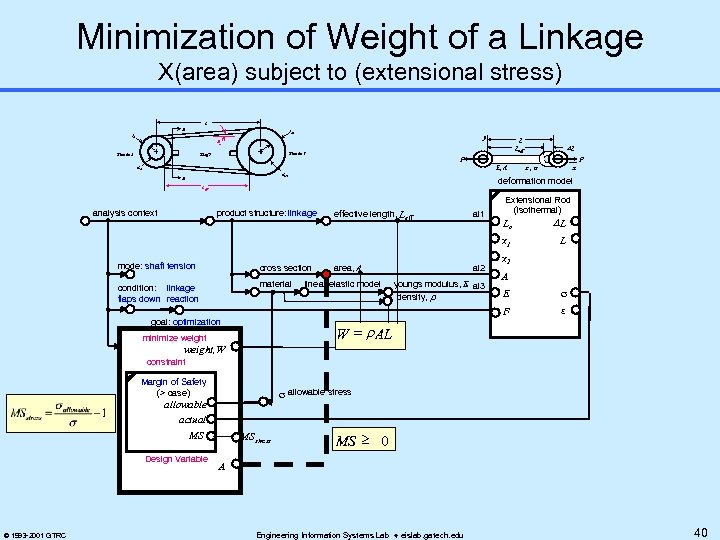 Minimization of Weight of a Linkage X(area) subject to (extensional stress) L A ts 1 ts 2 y qs Sleeve 1 L Leff Sleeve 2 Shaft P E, A ds 1 ds 2 A e, s x product structure: linkage effective length, Leff al 1 Extensional Rod (isothermal) mode: shaft tension cross section condition: linkage flaps down reaction material area, A linear elastic model al 2 youngs modulus, E al 3 density, r Lo L x 1 L x 2 A E F goal: optimization P deformation model Leff analysis context L e W = r. AL minimize weight, W constraint Margin of Safety (> case) allowable stress allowable Leff actual MS Design Variable © 1993 -2001 GTRC MSstress MS ³ 0 A Engineering Information Systems Lab eislab. gatech. edu 40
Minimization of Weight of a Linkage X(area) subject to (extensional stress) L A ts 1 ts 2 y qs Sleeve 1 L Leff Sleeve 2 Shaft P E, A ds 1 ds 2 A e, s x product structure: linkage effective length, Leff al 1 Extensional Rod (isothermal) mode: shaft tension cross section condition: linkage flaps down reaction material area, A linear elastic model al 2 youngs modulus, E al 3 density, r Lo L x 1 L x 2 A E F goal: optimization P deformation model Leff analysis context L e W = r. AL minimize weight, W constraint Margin of Safety (> case) allowable stress allowable Leff actual MS Design Variable © 1993 -2001 GTRC MSstress MS ³ 0 A Engineering Information Systems Lab eislab. gatech. edu 40
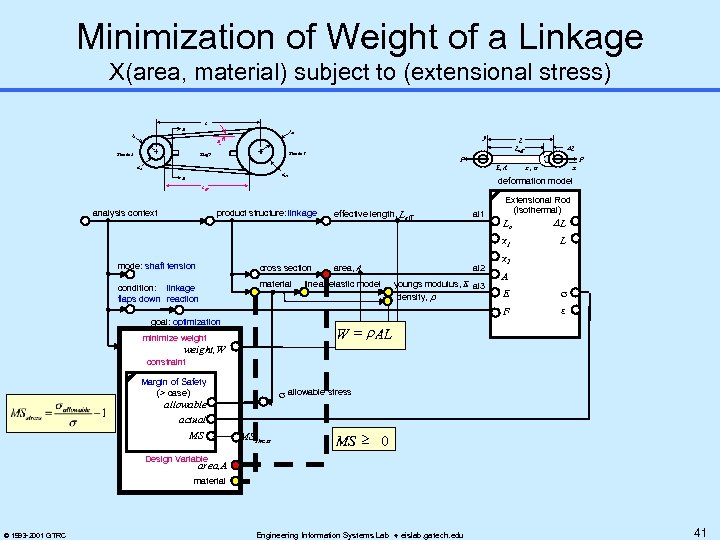 Minimization of Weight of a Linkage X(area, material) subject to (extensional stress) L A ts 1 ts 2 y qs Sleeve 1 L Leff Sleeve 2 Shaft P E, A ds 1 ds 2 A e, s x product structure: linkage effective length, Leff al 1 Extensional Rod (isothermal) mode: shaft tension cross section condition: linkage flaps down reaction material area, A linear elastic model al 2 youngs modulus, E al 3 density, r Lo L x 1 L x 2 A E F goal: optimization P deformation model Leff analysis context L e W = r. AL minimize weight, W constraint Margin of Safety (> case) allowable Leff actual MS allowable stress MS ³ 0 Design Variable area, A material © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 41
Minimization of Weight of a Linkage X(area, material) subject to (extensional stress) L A ts 1 ts 2 y qs Sleeve 1 L Leff Sleeve 2 Shaft P E, A ds 1 ds 2 A e, s x product structure: linkage effective length, Leff al 1 Extensional Rod (isothermal) mode: shaft tension cross section condition: linkage flaps down reaction material area, A linear elastic model al 2 youngs modulus, E al 3 density, r Lo L x 1 L x 2 A E F goal: optimization P deformation model Leff analysis context L e W = r. AL minimize weight, W constraint Margin of Safety (> case) allowable Leff actual MS allowable stress MS ³ 0 Design Variable area, A material © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 41
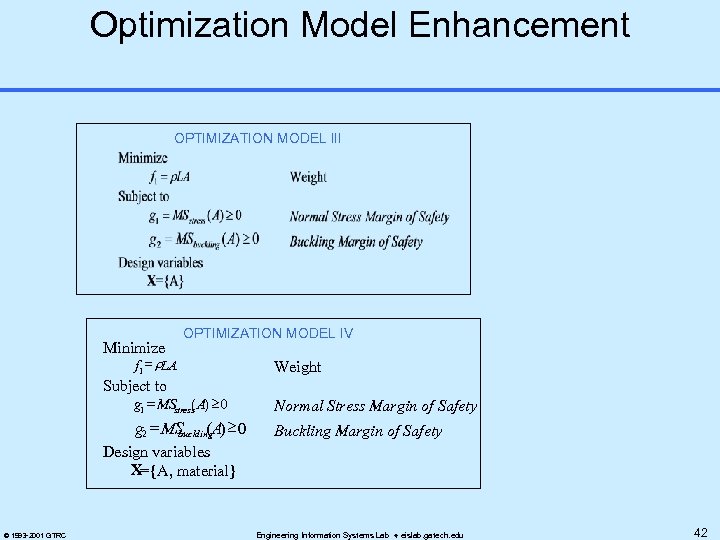 Optimization Model Enhancement OPTIMIZATION MODEL III Minimize OPTIMIZATION MODEL IV f 1 = r. LA Weight Subject to g 1 = MS (A) ³ 0 stress Normal Stress Margin of Safety g 2 = MS (A) ³ 0 buckling Buckling Margin of Safety Design variables X={A, material} © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 42
Optimization Model Enhancement OPTIMIZATION MODEL III Minimize OPTIMIZATION MODEL IV f 1 = r. LA Weight Subject to g 1 = MS (A) ³ 0 stress Normal Stress Margin of Safety g 2 = MS (A) ³ 0 buckling Buckling Margin of Safety Design variables X={A, material} © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 42
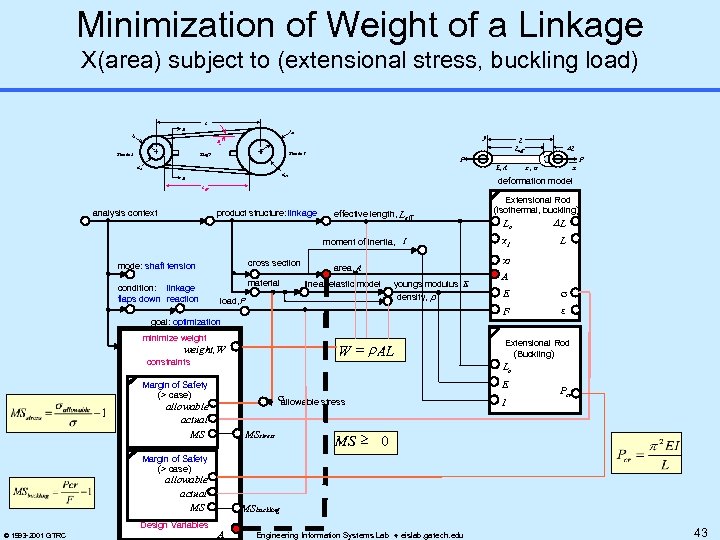 Minimization of Weight of a Linkage X(area) subject to (extensional stress, buckling load) L A ts 1 ts 2 y qs Sleeve 1 L Leff Sleeve 2 Shaft P E, A ds 1 ds 2 A product structure: linkage effective length, Leff moment of inertia, I cross section mode: shaft tension condition: linkage flaps down reaction material e, s x area, A linear elastic model youngs modulus, E density, r Extensional Rod (isothermal, buckling) Lo L x 1 L x 2 A goal: optimization W = r. AL weight, W constraints E F load, P minimize weight P deformation model Leff analysis context L e Extensional Rod (Buckling) Lo Margin of Safety (> case) allowable stress allowable Leff actual MS MSstress E I Pcr MS ³ 0 Margin of Safety (> case) allowable actual MS Design Variables © 1993 -2001 GTRC MSbuckling A Engineering Information Systems Lab eislab. gatech. edu 43
Minimization of Weight of a Linkage X(area) subject to (extensional stress, buckling load) L A ts 1 ts 2 y qs Sleeve 1 L Leff Sleeve 2 Shaft P E, A ds 1 ds 2 A product structure: linkage effective length, Leff moment of inertia, I cross section mode: shaft tension condition: linkage flaps down reaction material e, s x area, A linear elastic model youngs modulus, E density, r Extensional Rod (isothermal, buckling) Lo L x 1 L x 2 A goal: optimization W = r. AL weight, W constraints E F load, P minimize weight P deformation model Leff analysis context L e Extensional Rod (Buckling) Lo Margin of Safety (> case) allowable stress allowable Leff actual MS MSstress E I Pcr MS ³ 0 Margin of Safety (> case) allowable actual MS Design Variables © 1993 -2001 GTRC MSbuckling A Engineering Information Systems Lab eislab. gatech. edu 43
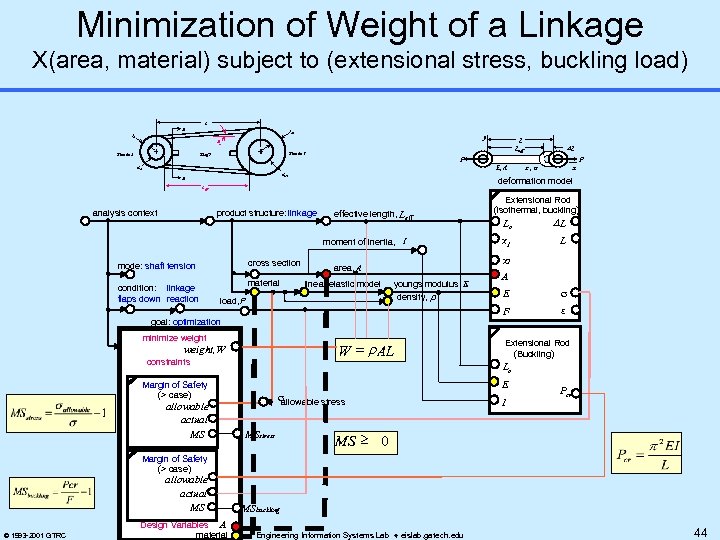 Minimization of Weight of a Linkage X(area, material) subject to (extensional stress, buckling load) L A ts 1 ts 2 y qs Sleeve 1 L Leff Sleeve 2 Shaft P E, A ds 1 ds 2 A product structure: linkage effective length, Leff moment of inertia, I cross section mode: shaft tension condition: linkage flaps down reaction material e, s x area, A linear elastic model youngs modulus, E density, r Extensional Rod (isothermal, buckling) Lo L x 1 L x 2 A goal: optimization W = r. AL weight, W constraints E F load, P minimize weight P deformation model Leff analysis context L e Extensional Rod (Buckling) Lo Margin of Safety (> case) allowable stress allowable Leff actual MS MSstress E I Pcr MS ³ 0 Margin of Safety (> case) allowable actual MS MSbuckling A © 1993 -2001 GTRC Design Variables material Engineering Information Systems Lab eislab. gatech. edu 44
Minimization of Weight of a Linkage X(area, material) subject to (extensional stress, buckling load) L A ts 1 ts 2 y qs Sleeve 1 L Leff Sleeve 2 Shaft P E, A ds 1 ds 2 A product structure: linkage effective length, Leff moment of inertia, I cross section mode: shaft tension condition: linkage flaps down reaction material e, s x area, A linear elastic model youngs modulus, E density, r Extensional Rod (isothermal, buckling) Lo L x 1 L x 2 A goal: optimization W = r. AL weight, W constraints E F load, P minimize weight P deformation model Leff analysis context L e Extensional Rod (Buckling) Lo Margin of Safety (> case) allowable stress allowable Leff actual MS MSstress E I Pcr MS ³ 0 Margin of Safety (> case) allowable actual MS MSbuckling A © 1993 -2001 GTRC Design Variables material Engineering Information Systems Lab eislab. gatech. edu 44
![Status: Optimization u u u Initial Ph. D thesis completed [Cimtalay, 2001] Seeking insertion Status: Optimization u u u Initial Ph. D thesis completed [Cimtalay, 2001] Seeking insertion](https://present5.com/presentation/e264909ed4c610c229de7f819f5b8f66/image-45.jpg) Status: Optimization u u u Initial Ph. D thesis completed [Cimtalay, 2001] Seeking insertion & extension opportunities Need to leverage recent optimization tools – Ex. i. SIGHT, Product. Center, … – Provide enhanced modularity & knowledge capture © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 45
Status: Optimization u u u Initial Ph. D thesis completed [Cimtalay, 2001] Seeking insertion & extension opportunities Need to leverage recent optimization tools – Ex. i. SIGHT, Product. Center, … – Provide enhanced modularity & knowledge capture © 1993 -2001 GTRC Engineering Information Systems Lab eislab. gatech. edu 45


