Adsorption Lecture Notes ENVE 542 Air Pollution Control

Adsorption Lecture Notes ENVE 542 Air Pollution Control Technologies By Dr. Pınar Ergenekon GYTE 2010 Fall

Useful When • The pollutant gas is noncombustible or difficult to burn • The pollutant is sufficiently valuable to warrant recovery • The pollutant is in very dilute concentration • It is also used for purification of gases containing only small amounts of pollutants that are difficult to clean by other means ENVE 542 GYTE Çevre Müh. 2

Adsorption Process • Classified as Physical and Chemical Ads. – 1) Physical adsorption • The gas molecules adhere to the surface of the solid adsorbent as a result of intermolecular attractive forces (van der Waals forces) between them • The process is exothermic: the heat liberated is in the order of the enthalpy of condensation of vapor (2 -20 k. J/gmole) • The process is reversible (recovery of adsorbent material or adsorbed gas is possible) by increasing the temperature or lowering the adsorbate conc. • Physical adsorption usually directly proportional to the amount of solid surface area • Adsorbate can be adsorbed on a monolayer or a number of layers • The adsorption rate is generally quite rapid ENVE 542 GYTE Çevre Müh. 3

Adsorption Process – 2) Chemical adsorption • Results from a chemical interaction between the adsorbate and adsorbent. Therefore formed bond is much stronger than that for physical adsorption • Heat liberated during chemisorption is in the range of 20 -400 kj/g mole • It is frequently irreversible. On desorption the chemical nature of the original adsorbate will have undergone a change. • Only a monomolecular layer of adsorbate appears on the adsorbing medium ENVE 542 GYTE Çevre Müh. 4

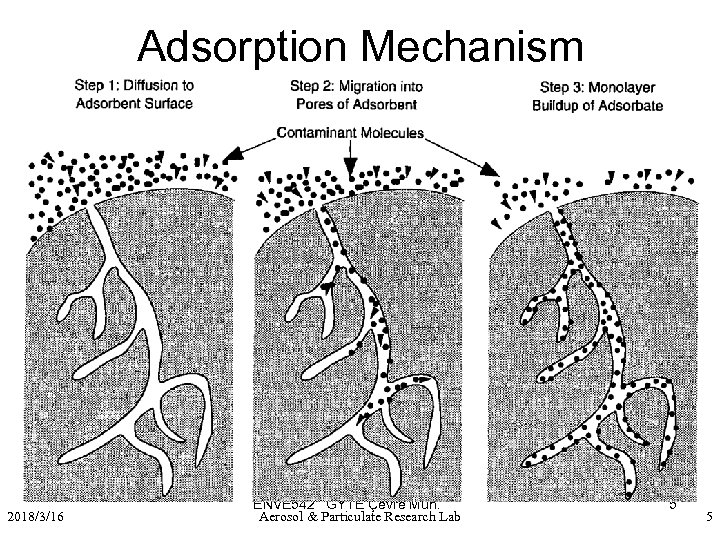Adsorption Mechanism – 2) Chemical adsorption • Results from a chemical interaction between the adsorbate and adsorbent. Therefore formed bond is much stronger than that for physical adsorption • Heat liberated during chemisorption is in the range of 20 -400 kj/g mole 2018/3/16 ENVE 542 GYTE Çevre Müh. Aerosol & Particulate Research Lab 5 5

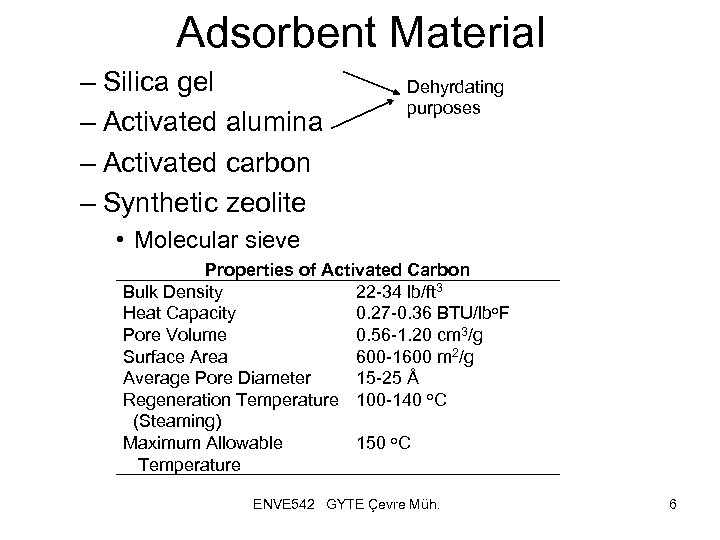Adsorbent Material – Silica gel – Activated alumina – Activated carbon – Synthetic zeolite Dehyrdating purposes • Molecular sieve Properties of Activated Carbon Bulk Density 22 -34 lb/ft 3 Heat Capacity 0. 27 -0. 36 BTU/lbo. F Pore Volume 0. 56 -1. 20 cm 3/g Surface Area 600 -1600 m 2/g Average Pore Diameter 15 -25 Å Regeneration Temperature 100 -140 o. C (Steaming) Maximum Allowable 150 o. C Temperature ENVE 542 GYTE Çevre Müh. 6

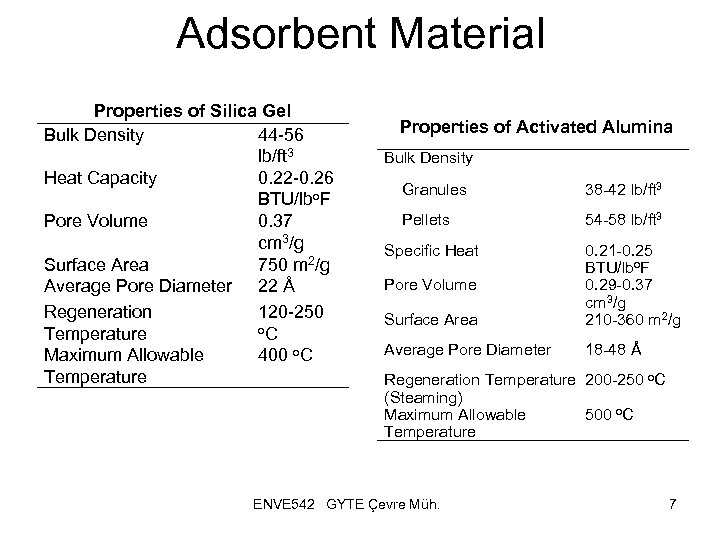Adsorbent Material Properties of Silica Gel Bulk Density 44 -56 lb/ft 3 Heat Capacity 0. 22 -0. 26 BTU/lbo. F Pore Volume 0. 37 cm 3/g Surface Area 750 m 2/g Average Pore Diameter 22 Å Regeneration 120 -250 o. C Temperature Maximum Allowable 400 o. C Temperature Properties of Activated Alumina Bulk Density Granules 38 -42 lb/ft 3 Pellets 54 -58 lb/ft 3 Specific Heat Surface Area 0. 21 -0. 25 BTU/lbo. F 0. 29 -0. 37 cm 3/g 210 -360 m 2/g Average Pore Diameter 18 -48 Å Pore Volume Regeneration Temperature 200 -250 o. C (Steaming) Maximum Allowable 500 o. C Temperature ENVE 542 GYTE Çevre Müh. 7

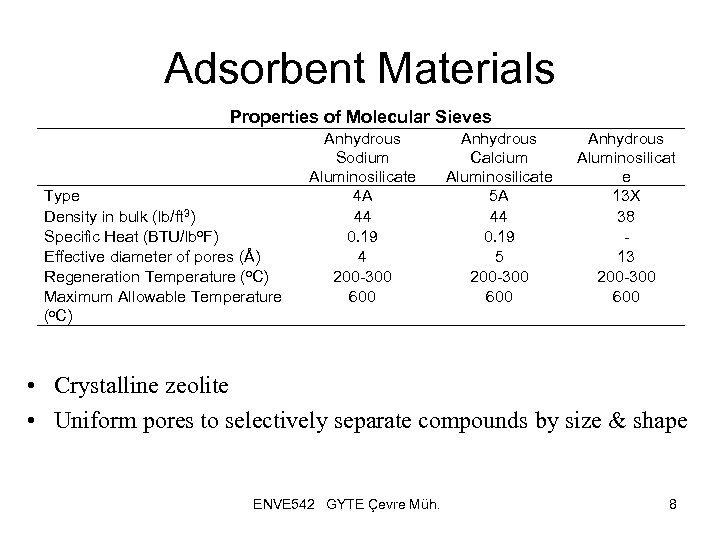Adsorbent Materials Properties of Molecular Sieves Type Density in bulk (lb/ft 3) Specific Heat (BTU/lbo. F) Effective diameter of pores (Å) Regeneration Temperature (o. C) Maximum Allowable Temperature (o. C) Anhydrous Sodium Aluminosilicate 4 A 44 0. 19 4 200 -300 600 Anhydrous Calcium Aluminosilicate 5 A 44 0. 19 5 200 -300 600 Anhydrous Aluminosilicat e 13 X 38 13 200 -300 600 • Crystalline zeolite • Uniform pores to selectively separate compounds by size & shape ENVE 542 GYTE Çevre Müh. 8

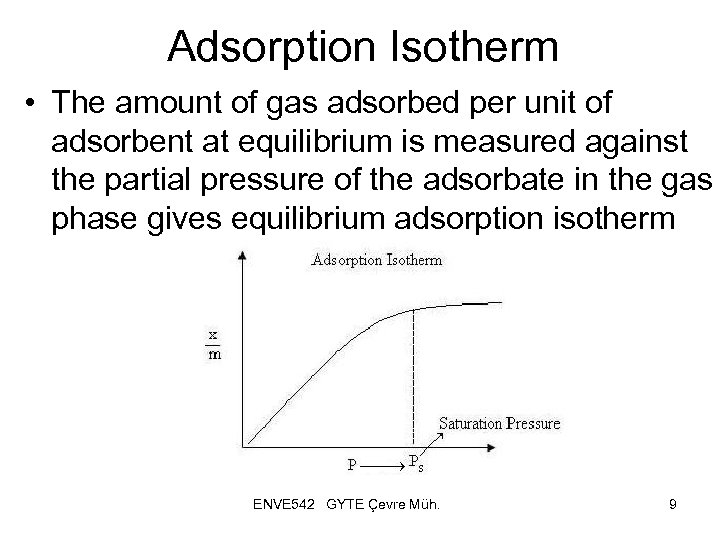Adsorption Isotherm • The amount of gas adsorbed per unit of adsorbent at equilibrium is measured against the partial pressure of the adsorbate in the gas phase gives equilibrium adsorption isotherm ENVE 542 GYTE Çevre Müh. 9

Adsorption Isotherm • In general, an adsorption isotherm relates the volume or mass adsorbed to the partial pressure or concentration of the adsorbate in the main gas stream at a given temperature • The equilibrium concentration adsorbed is very sensitive to T • There are many equations proposed to fit analyticaly the various experimental istoherms ENVE 542 GYTE Çevre Müh. 10

Adsorption Isotherms • In physical adsorption Brunauer, Emmett, and Teller (BET) is frequenlty used V: volume the amount of adsorbed gas would fill at a given pressure and temperature Vm: volume adsorbed it a layer one molecule thick fills the surface Po: vapor pressure of the adsorbate at the temperature of the system P: actual partial pressure of the adsorbate c: a parameter of the particular adsorption process ENVE 542 GYTE Çevre Müh. 11

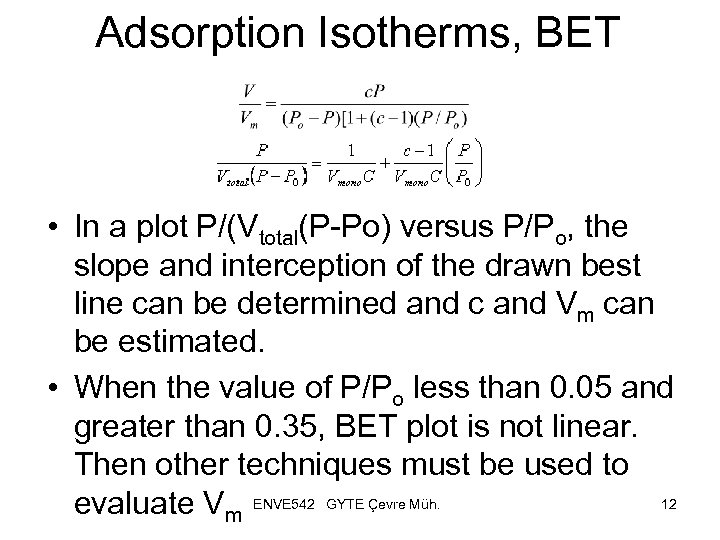Adsorption Isotherms, BET • In a plot P/(Vtotal(P-Po) versus P/Po, the slope and interception of the drawn best line can be determined and c and Vm can be estimated. • When the value of P/Po less than 0. 05 and greater than 0. 35, BET plot is not linear. Then other techniques must be used to 12 evaluate Vm ENVE 542 GYTE Çevre Müh.

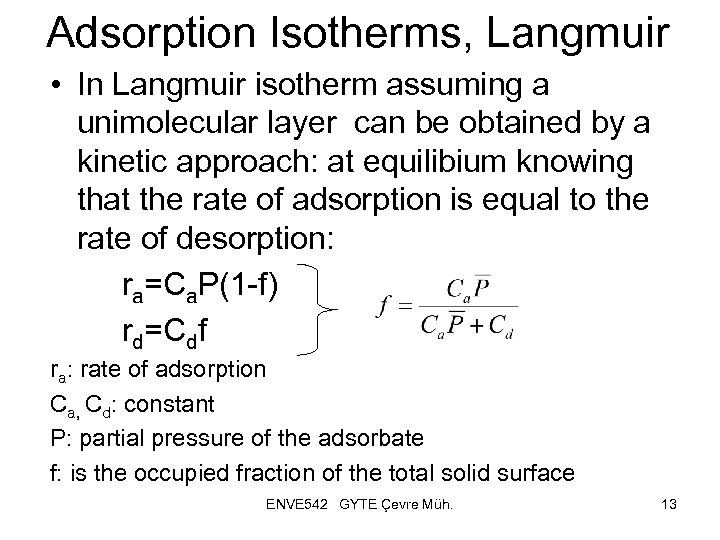Adsorption Isotherms, Langmuir • In Langmuir isotherm assuming a unimolecular layer can be obtained by a kinetic approach: at equilibium knowing that the rate of adsorption is equal to the rate of desorption: ra=Ca. P(1 -f) rd=Cdf ra: rate of adsorption Ca, Cd: constant P: partial pressure of the adsorbate f: is the occupied fraction of the total solid surface ENVE 542 GYTE Çevre Müh. 13

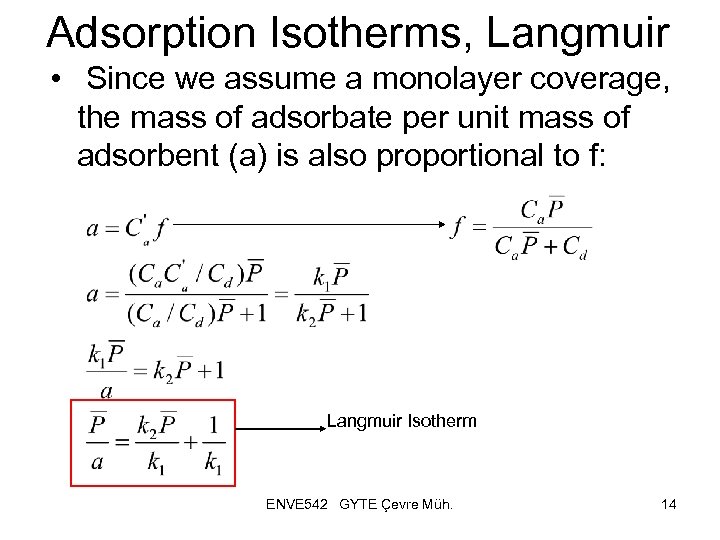Adsorption Isotherms, Langmuir • Since we assume a monolayer coverage, the mass of adsorbate per unit mass of adsorbent (a) is also proportional to f: Langmuir Isotherm ENVE 542 GYTE Çevre Müh. 14

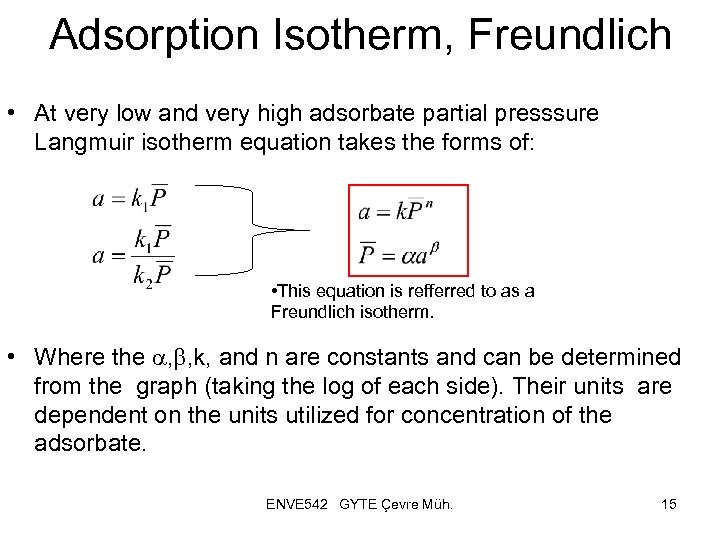Adsorption Isotherm, Freundlich • At very low and very high adsorbate partial presssure Langmuir isotherm equation takes the forms of: • This equation is refferred to as a Freundlich isotherm. • Where the a, b, k, and n are constants and can be determined from the graph (taking the log of each side). Their units are dependent on the units utilized for concentration of the adsorbate. ENVE 542 GYTE Çevre Müh. 15

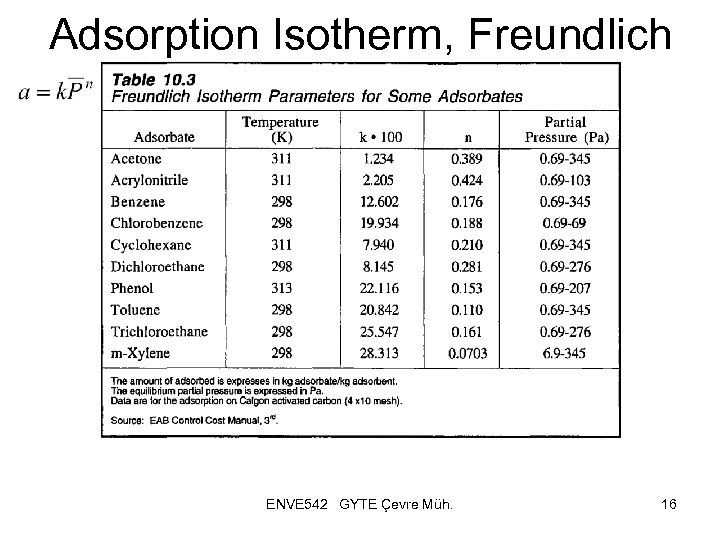Adsorption Isotherm, Freundlich ENVE 542 GYTE Çevre Müh. 16

Adsorption Isotherm Data (AID) • Yaws summarized the adsorption capacity of 283 organic compounds on activated carbon: • logx=a+blog. Ce+c(log. Ce)2 • x is the adsorption capacity (g of pollutant/g of carbon), Ce is the pollutant conc. in ppm, a, b, and c are correlation constants Pollutant a b c Benzene -1. 189 0, 288 -0, 0238 Ethyl arcrylate -1. 439 0, 27 -0, 0089 Monochlorobenzene -0. 973 0, 306 -0, 0335 Phenol -0, 544 0, 103 -0, 0109 MIBK -0, 898 0, 206 -0, 0206 Toluene -0, 885 0, 208 -0, 0202 -0, 914 0, 17 -0, 0158 Heptane ENVE 542 GYTE Çevre Müh. 17

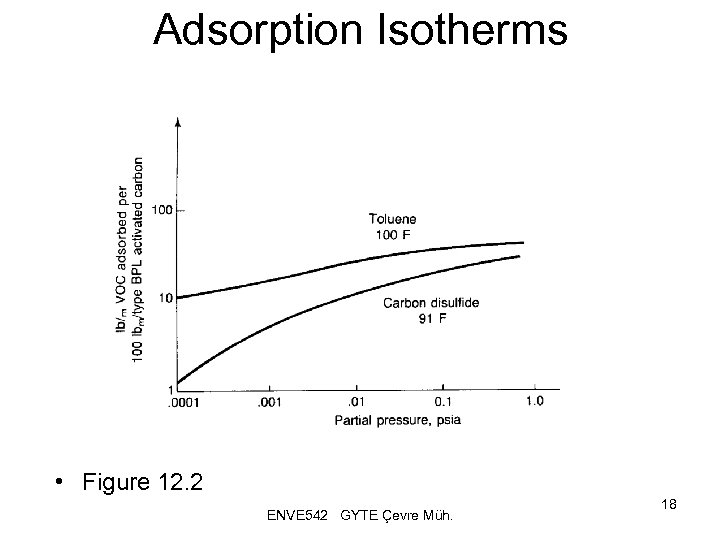Adsorption Isotherms • Figure 12. 2 ENVE 542 GYTE Çevre Müh. 18

Adsorption Isotherm Data (AID) • Other excellent sources for AID are the various vendors of the adsorbent • In the absence of experimental data on a specific carbon, an equation developed by Calgon Corporation which is a modification of Dubinin-Radushkevich equation can be used to estimate the adsorption capacity, ENVE 542 GYTE Çevre Müh. 19

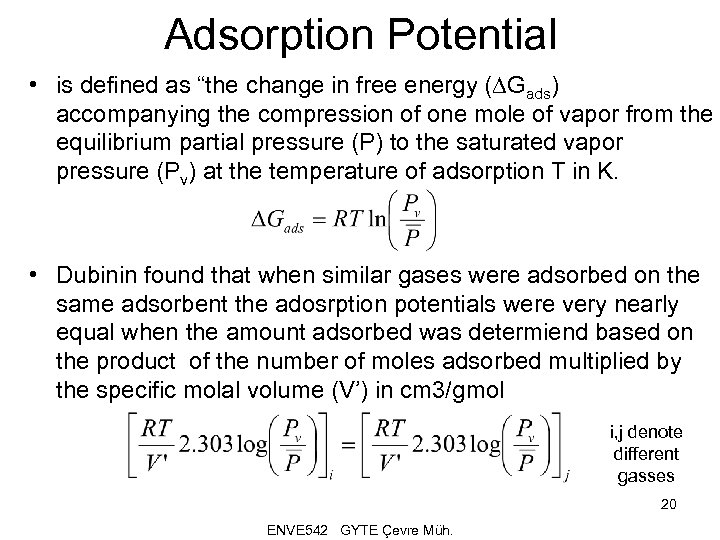Adsorption Potential • is defined as “the change in free energy (DGads) accompanying the compression of one mole of vapor from the equilibrium partial pressure (P) to the saturated vapor pressure (Pv) at the temperature of adsorption T in K. • Dubinin found that when similar gases were adsorbed on the same adsorbent the adosrption potentials were very nearly equal when the amount adsorbed was determiend based on the product of the number of moles adsorbed multiplied by the specific molal volume (V’) in cm 3/gmol i, j denote different gasses 20 ENVE 542 GYTE Çevre Müh.

Adsorption Potential • is defined as “the change in free energy (DGads) accompanying the compression of one mole of vapor from the equilibrium partial pressure (P) to the saturated vapor pressure (Pv) at the temperature of adsorption T in K. • Dubinin found that when similar gases were adsorbed on the same adsorbent the adosrption potentials were very nearly equal when the amount adsorbed was determiend based on the product of the number of moles adsorbed multiplied by the specific molal volume (V’) in cm 3/gmol i, j denote different gasses 21 ENVE 542 GYTE Çevre Müh.

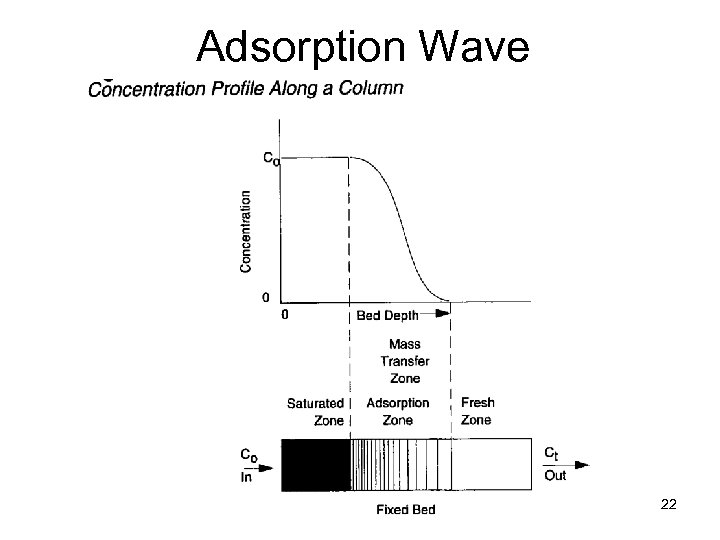Adsorption Wave ENVE 542 GYTE Çevre Müh. 22

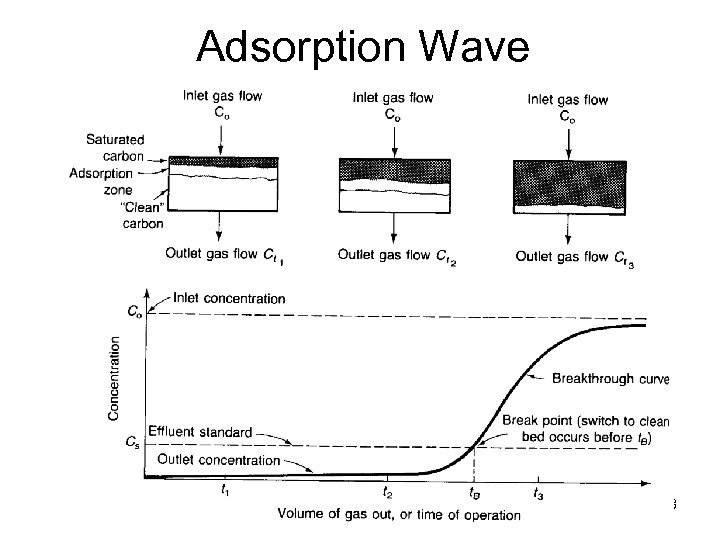Adsorption Wave ENVE 542 GYTE Çevre Müh. 23

Adsorption Zone The length of Adsorption Zone (AZ) is a function of the rate of transfer of adsorbate from the gas to the adsorbent A shallow AZ indicates good adsorbent utilization and is represented by a step breakthrough curve The length of AZ determined the minimum depth of the adsorbent bed On the next slides we will try to analyze this zone but note that under actual plant operating conditions bed capacity will seldom exceed 30 -40% of that indicated by an equilibrium isotherm Hence system design is based primarily on previous plant experment and pilot scale studies. ENVE 542 GYTE Çevre Müh. 24

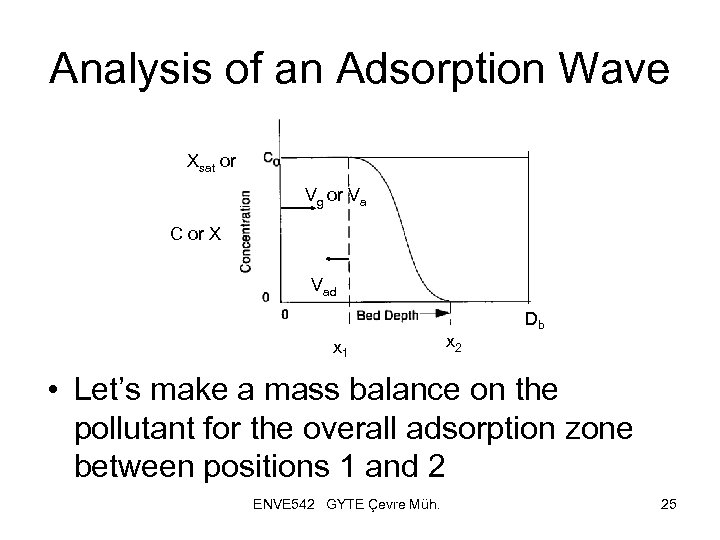Analysis of an Adsorption Wave Xsat or Vg or Va C or X Vad x 1 x 2 Db • Let’s make a mass balance on the pollutant for the overall adsorption zone between positions 1 and 2 ENVE 542 GYTE Çevre Müh. 25

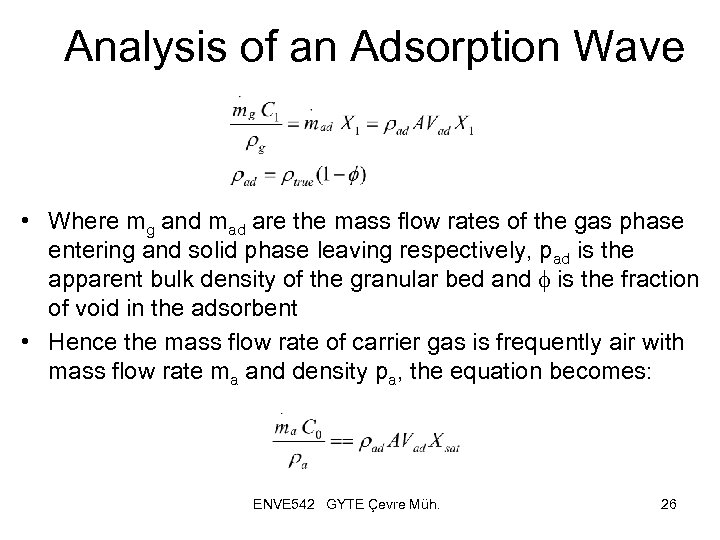Analysis of an Adsorption Wave • Where mg and mad are the mass flow rates of the gas phase entering and solid phase leaving respectively, pad is the apparent bulk density of the granular bed and f is the fraction of void in the adsorbent • Hence the mass flow rate of carrier gas is frequently air with mass flow rate ma and density pa, the equation becomes: ENVE 542 GYTE Çevre Müh. 26

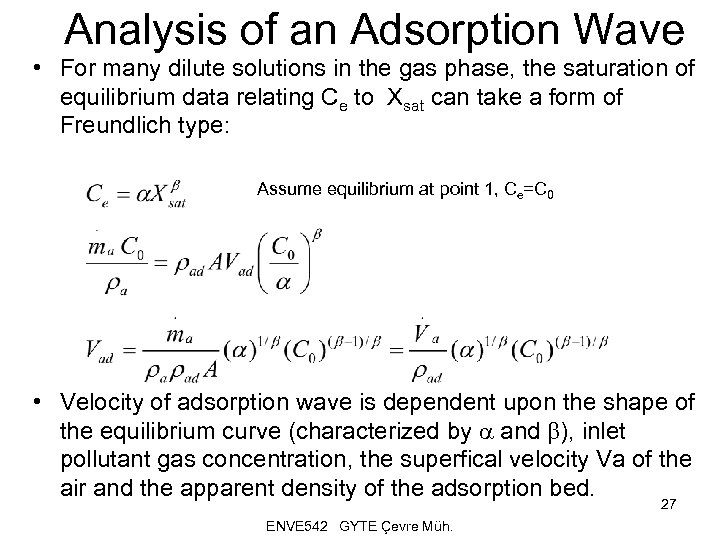Analysis of an Adsorption Wave • For many dilute solutions in the gas phase, the saturation of equilibrium data relating Ce to Xsat can take a form of Freundlich type: Assume equilibrium at point 1, Ce=C 0 • Velocity of adsorption wave is dependent upon the shape of the equilibrium curve (characterized by a and b), inlet pollutant gas concentration, the superfical velocity Va of the air and the apparent density of the adsorption bed. 27 ENVE 542 GYTE Çevre Müh.

Analysis of an Adsorption Wave • To determine thickness of the adsorption wave, develop a relationship between C and x in the adsorption zone Mp is the rate of mass transfer of pollutant gas onto the solid phase • Another independent equation for mp can be developed by general theory of mass transfer: ENVE 542 GYTE Çevre Müh. 28

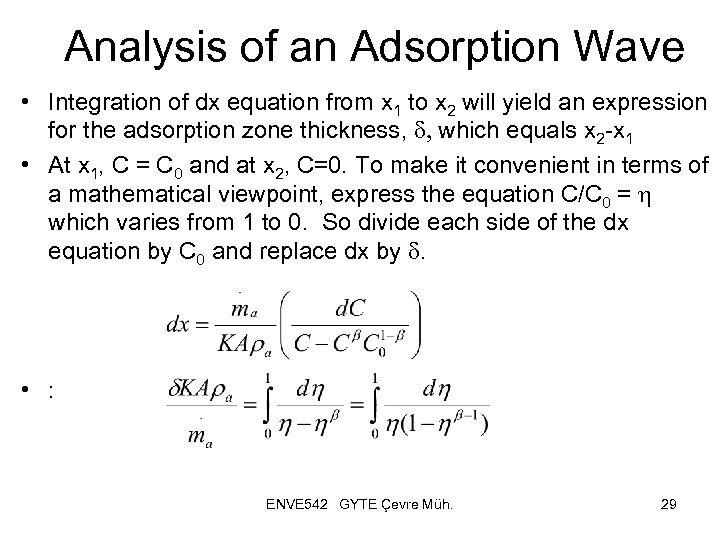Analysis of an Adsorption Wave • Integration of dx equation from x 1 to x 2 will yield an expression for the adsorption zone thickness, d, which equals x 2 -x 1 • At x 1, C = C 0 and at x 2, C=0. To make it convenient in terms of a mathematical viewpoint, express the equation C/C 0 = h which varies from 1 to 0. So divide each side of the dx equation by C 0 and replace dx by d. • : ENVE 542 GYTE Çevre Müh. 29

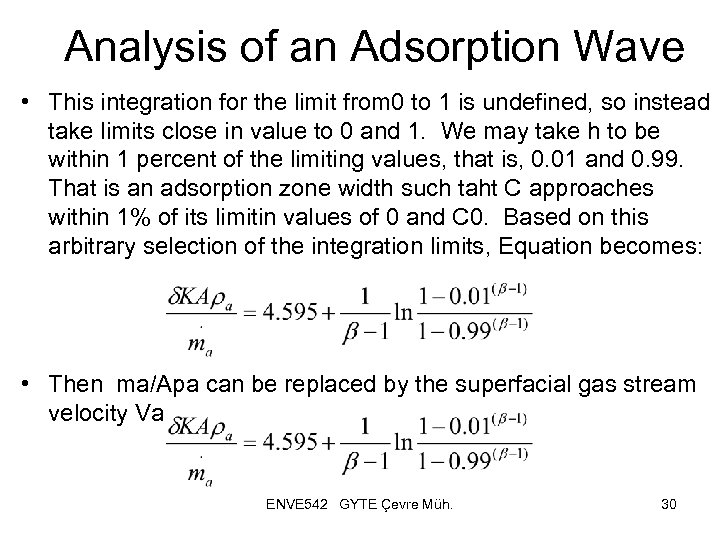Analysis of an Adsorption Wave • This integration for the limit from 0 to 1 is undefined, so instead take limits close in value to 0 and 1. We may take h to be within 1 percent of the limiting values, that is, 0. 01 and 0. 99. That is an adsorption zone width such taht C approaches within 1% of its limitin values of 0 and C 0. Based on this arbitrary selection of the integration limits, Equation becomes: • Then ma/Apa can be replaced by the superfacial gas stream velocity Va ENVE 542 GYTE Çevre Müh. 30

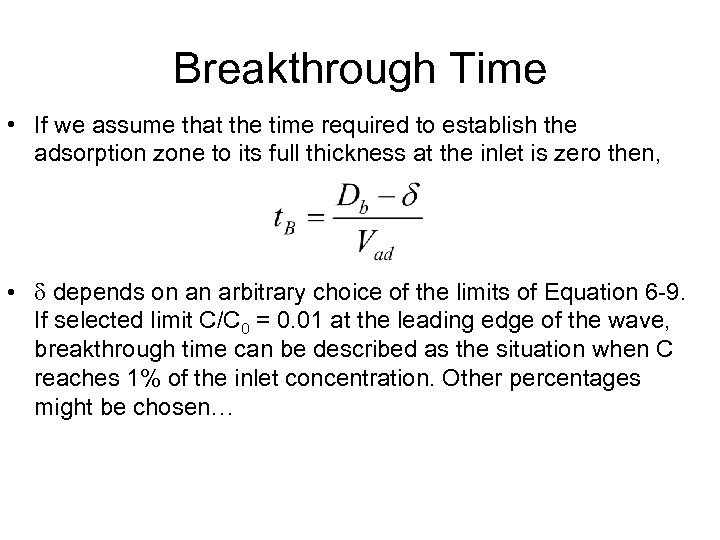Breakthrough Time • If we assume that the time required to establish the adsorption zone to its full thickness at the inlet is zero then, • d depends on an arbitrary choice of the limits of Equation 6 -9. If selected limit C/C 0 = 0. 01 at the leading edge of the wave, breakthrough time can be described as the situation when C reaches 1% of the inlet concentration. Other percentages might be chosen…

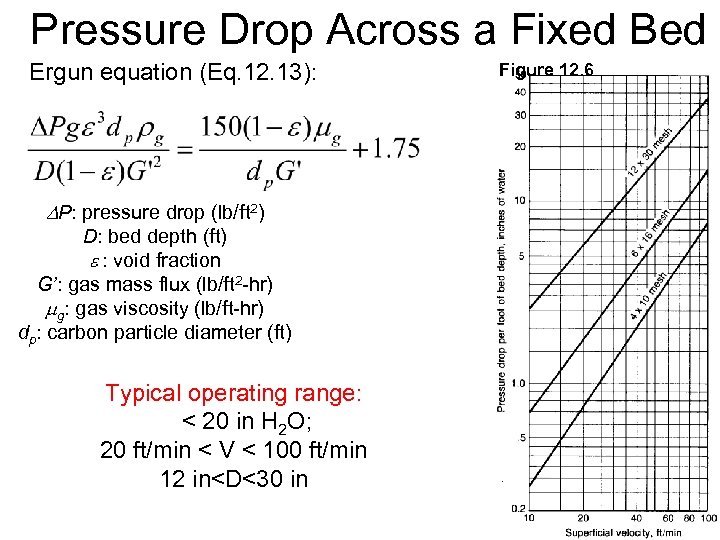Pressure Drop Across a Fixed Bed Ergun equation (Eq. 12. 13): Figure 12. 6 P: pressure drop (lb/ft 2) D: bed depth (ft) e : void fraction G’: gas mass flux (lb/ft 2 -hr) mg: gas viscosity (lb/ft-hr) dp: carbon particle diameter (ft) Typical operating range: < 20 in H 2 O; 20 ft/min < V < 100 ft/min 12 in

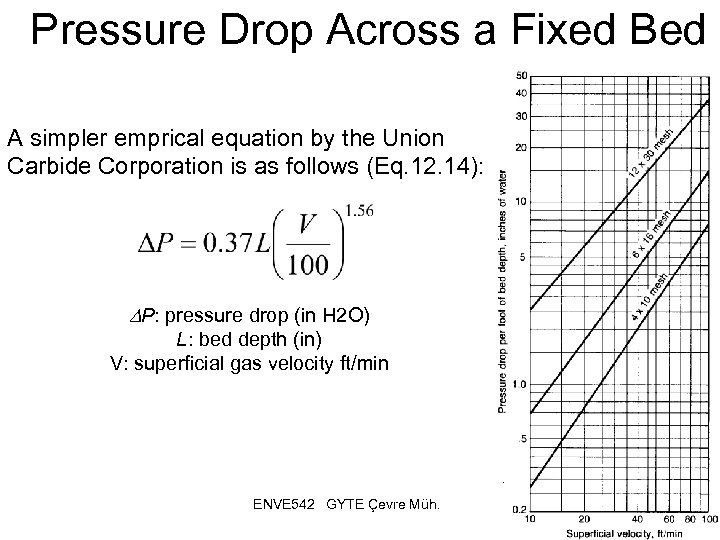Pressure Drop Across a Fixed Bed A simpler emprical equation by the Union Carbide Corporation is as follows (Eq. 12. 14): P: pressure drop (in H 2 O) L: bed depth (in) V: superficial gas velocity ft/min ENVE 542 GYTE Çevre Müh. 33

Example 12. 1 • An activated-carbon bed that is 12 ft x 6 ft x 2 ft deep is used in a benzene recovery system. The system is online for 1 hour and is then regereranted for one hour. The influent gas stream flow at 7500 acfm and contains 5000 ppmv benzene at 1 atm and 100 F. The operating cpacity of the bed is 10 lbm benzene per 100 lbm carbon. The physical properties of the carbon are as follows: bulk density: 30 lbm/ft 3, void fraction=0. 35, particle size=4 x 10 mesh (0. 011 ft) Determine the pressure drop across the bed from a)Eq 12. 13 b) Eq. 12. 14 and c) Figure 12. 6 ENVE 542 GYTE Çevre Müh. 34

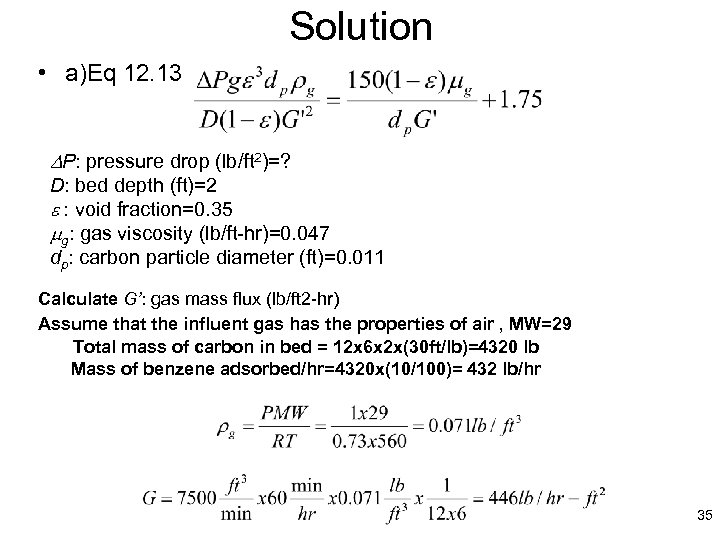Solution • a)Eq 12. 13 P: pressure drop (lb/ft 2)=? D: bed depth (ft)=2 e : void fraction=0. 35 mg: gas viscosity (lb/ft-hr)=0. 047 dp: carbon particle diameter (ft)=0. 011 Calculate G’: gas mass flux (lb/ft 2 -hr) Assume that the influent gas has the properties of air , MW=29 Total mass of carbon in bed = 12 x 6 x 2 x(30 ft/lb)=4320 lb Mass of benzene adsorbed/hr=4320 x(10/100)= 432 lb/hr 35

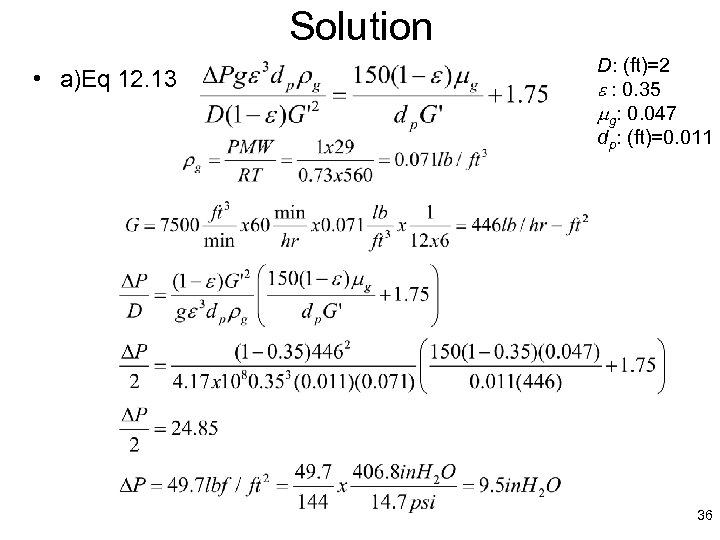Solution • a)Eq 12. 13 D: (ft)=2 e : 0. 35 mg: 0. 047 dp: (ft)=0. 011 36

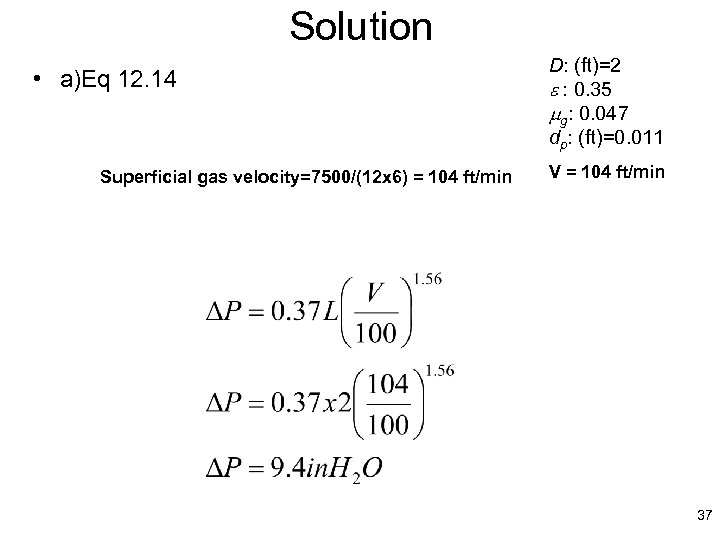Solution • a)Eq 12. 14 Superficial gas velocity=7500/(12 x 6) = 104 ft/min D: (ft)=2 e : 0. 35 mg: 0. 047 dp: (ft)=0. 011 V = 104 ft/min 37

Solution • a)Eq 12. 13 ENVE 542 GYTE Çevre Müh. 38

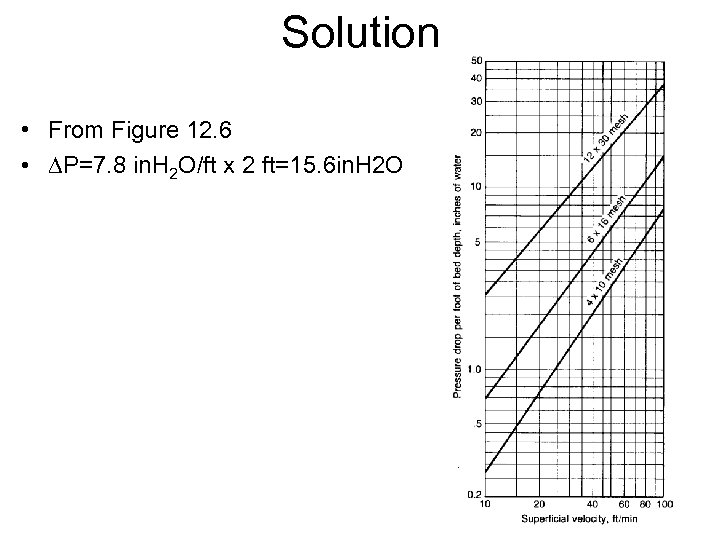Solution • From Figure 12. 6 • DP=7. 8 in. H 2 O/ft x 2 ft=15. 6 in. H 2 O

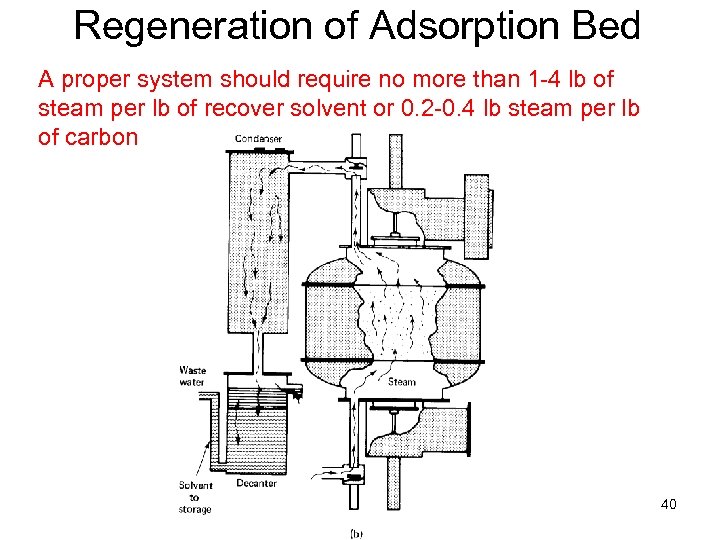Regeneration of Adsorption Bed A proper system should require no more than 1 -4 lb of steam per lb of recover solvent or 0. 2 -0. 4 lb steam per lb of carbon ENVE 542 GYTE Çevre Müh. 40

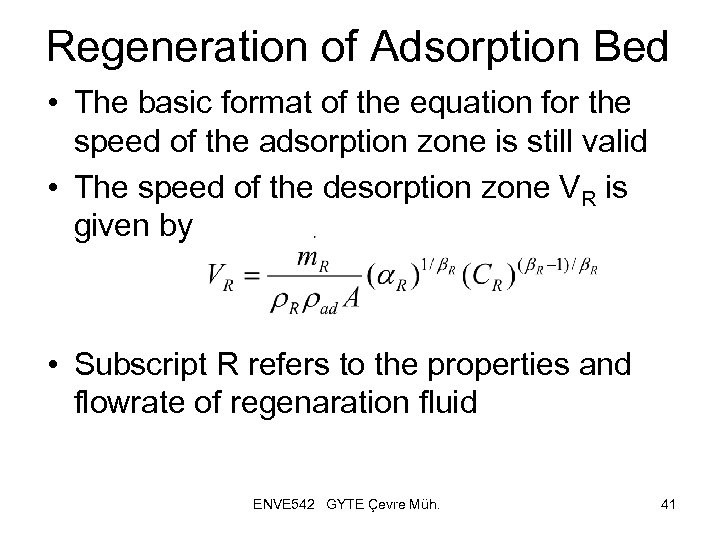Regeneration of Adsorption Bed • The basic format of the equation for the speed of the adsorption zone is still valid • The speed of the desorption zone VR is given by • Subscript R refers to the properties and flowrate of regenaration fluid ENVE 542 GYTE Çevre Müh. 41

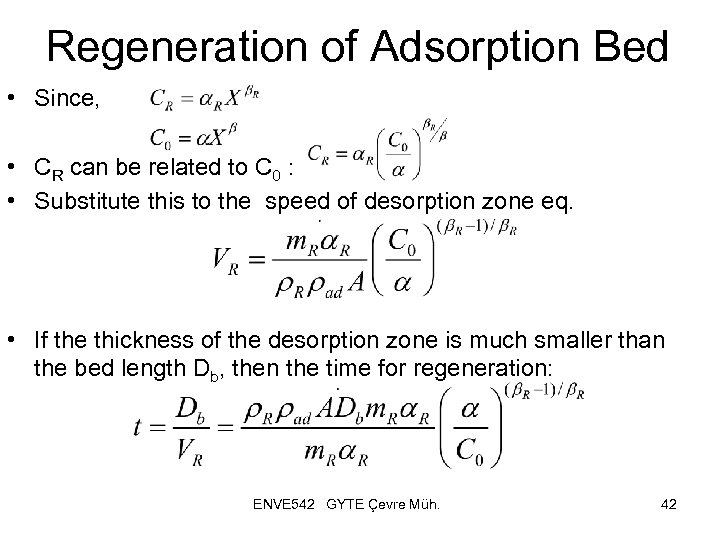Regeneration of Adsorption Bed • Since, • CR can be related to C 0 : • Substitute this to the speed of desorption zone eq. • If the thickness of the desorption zone is much smaller than the bed length Db, then the time for regeneration: ENVE 542 GYTE Çevre Müh. 42

Example 12. 2 • Prepare a preliminary design for a carbon adsroption system to control a stream of solvent laden air from a plastics extruder local exhaust system. The exhaust stream temperature is 95 F and it contains 1880 ppm of n-pentane. The plant engineer has provided the following info: – – Other gases contaminants: none PM contaminants: plant fugitive dust only. Flow rate: 5500 acfm Extruder exhaus pressure: -4. 5 in H 2 O ENVE 542 GYTE Çevre Müh. 43

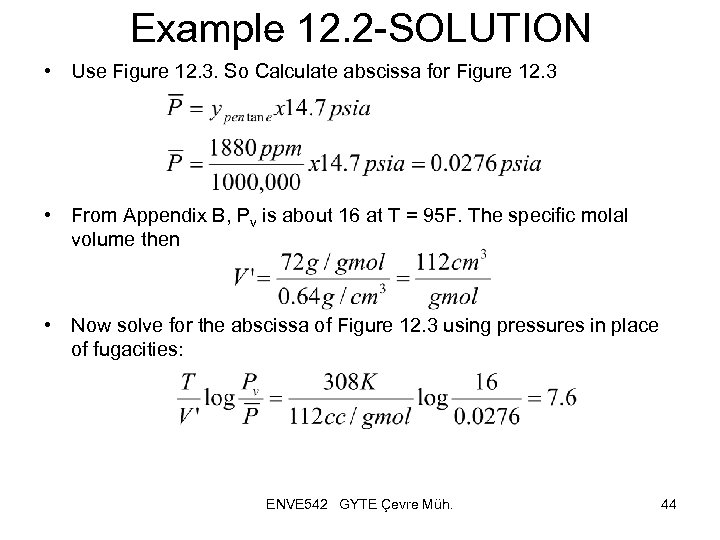Example 12. 2 -SOLUTION • Use Figure 12. 3. So Calculate abscissa for Figure 12. 3 • From Appendix B, Pv is about 16 at T = 95 F. The specific molal volume then • Now solve for the abscissa of Figure 12. 3 using pressures in place of fugacities: ENVE 542 GYTE Çevre Müh. 44

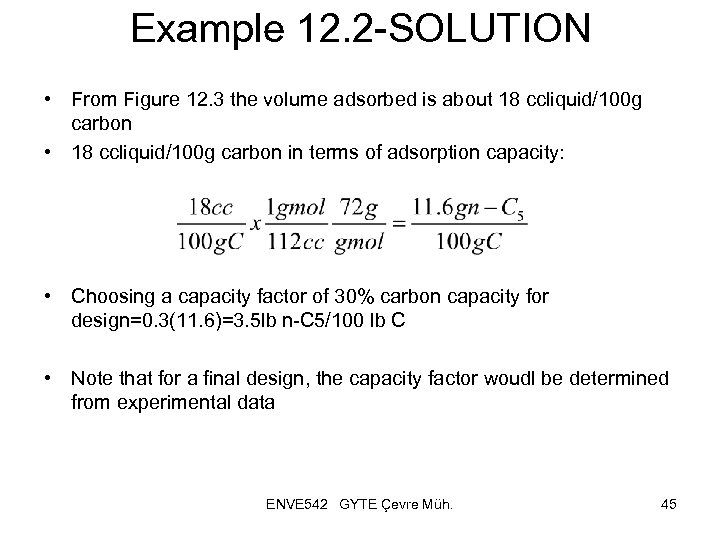Example 12. 2 -SOLUTION • From Figure 12. 3 the volume adsorbed is about 18 ccliquid/100 g carbon • 18 ccliquid/100 g carbon in terms of adsorption capacity: • Choosing a capacity factor of 30% carbon capacity for design=0. 3(11. 6)=3. 5 lb n-C 5/100 lb C • Note that for a final design, the capacity factor woudl be determined from experimental data ENVE 542 GYTE Çevre Müh. 45

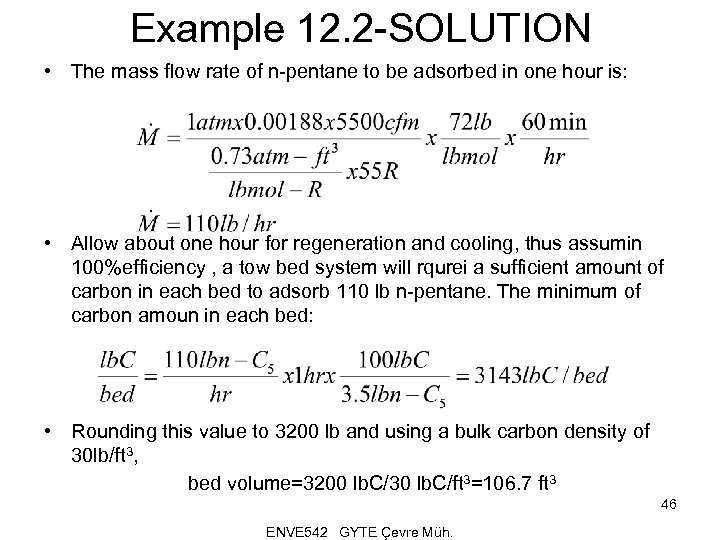Example 12. 2 -SOLUTION • The mass flow rate of n-pentane to be adsorbed in one hour is: • Allow about one hour for regeneration and cooling, thus assumin 100%efficiency , a tow bed system will rqurei a sufficient amount of carbon in each bed to adsorb 110 lb n-pentane. The minimum of carbon amoun in each bed: • Rounding this value to 3200 lb and using a bulk carbon density of 30 lb/ft 3, bed volume=3200 lb. C/30 lb. C/ft 3=106. 7 ft 3 46 ENVE 542 GYTE Çevre Müh.

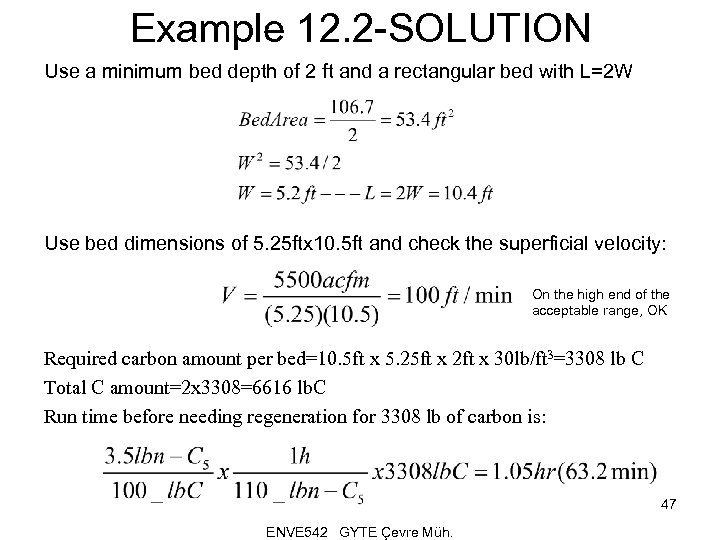Example 12. 2 -SOLUTION Use a minimum bed depth of 2 ft and a rectangular bed with L=2 W Use bed dimensions of 5. 25 ftx 10. 5 ft and check the superficial velocity: On the high end of the acceptable range, OK Required carbon amount per bed=10. 5 ft x 5. 25 ft x 2 ft x 30 lb/ft 3=3308 lb C Total C amount=2 x 3308=6616 lb. C Run time before needing regeneration for 3308 lb of carbon is: 47 ENVE 542 GYTE Çevre Müh.

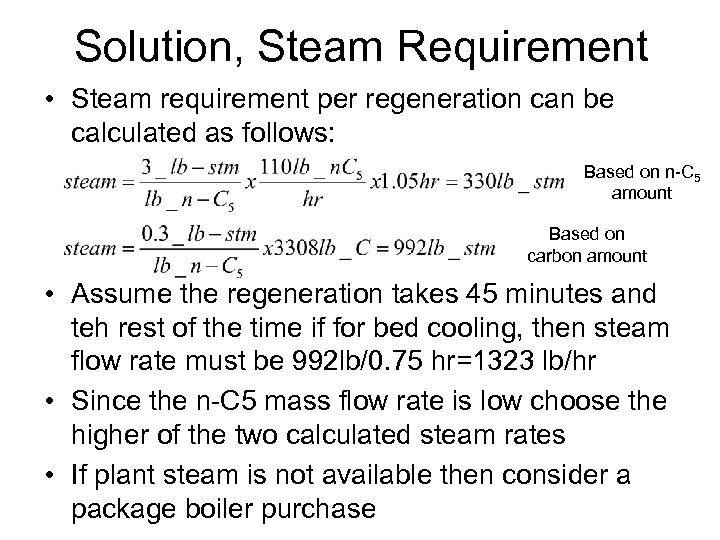Solution, Steam Requirement • Steam requirement per regeneration can be calculated as follows: Based on n-C 5 amount Based on carbon amount • Assume the regeneration takes 45 minutes and teh rest of the time if for bed cooling, then steam flow rate must be 992 lb/0. 75 hr=1323 lb/hr • Since the n-C 5 mass flow rate is low choose the higher of the two calculated steam rates • If plant steam is not available then consider a package boiler purchase

Solution, Bed Pressure Drop • From Figure 12. 6: DP 4 x 10=7. 5 in H 2 O x 2 ft = 15 in H 2 O DP 6 x 16=15 in H 2 O x 2 ft = 30 in H 2 O Seeing these high pressure values consider adding excess carbon and making the bed area larger

Solution, Blower Horsepower Requirment • The local exhaust system will be picking up plant fugitive dust, so a fabric filter or guard chamber should be installed before the carbon adsorption system • Assume a filter DP of 3 in H 2 O • Allow a pressure drop of 2 in. H 2 O for the inlet and exhust piping. Thus the fan mus provide a total DP of: • DP=3+2+15(-4. 5)=24. 5 in H 2 O • If we assume a blower efficiency of 60% then

SOLUTION • Preliminary Specification Summary Adsorber bed size=5. 25 ft x 10. 5 ft x 2 ft Mass of carbon per bed=3308 lb Steam Rqd=992 lb/regenx 24 regen/day=23800 lb/day Fan hp=35. 4 hp (buy a 40 hp motor)