1 LECTURE 31 Magnetic Fields 1 At the

1 LECTURE 31 Magnetic Fields 1 At the end of this lecture you should: • Understand how a magnetic field is defined implicitly by the Lorentz force • Be able to calculate the magnitude and direction of the force on a conductor of length, l, carrying current, I, in a magnetic field of field strength, B • Know the formula for the field strength due to a long straight wire carrying current, I, at a distance, r, from the wire • Understand how the definition of the ampere arises and be able to give the definition • Understand the concept of field lines for a magnetic field

Information • Exam and numerical answers are on Moodle • All students have labs today and onwards; inductance experiment is “functional” • NO use of mobile phones or music in labs • Arrive on time for labs: Students arriving late for labs will get reduced marks. Students arriving 15 or more minutes late will not be allowed top do a lab, and will get a zero mark.

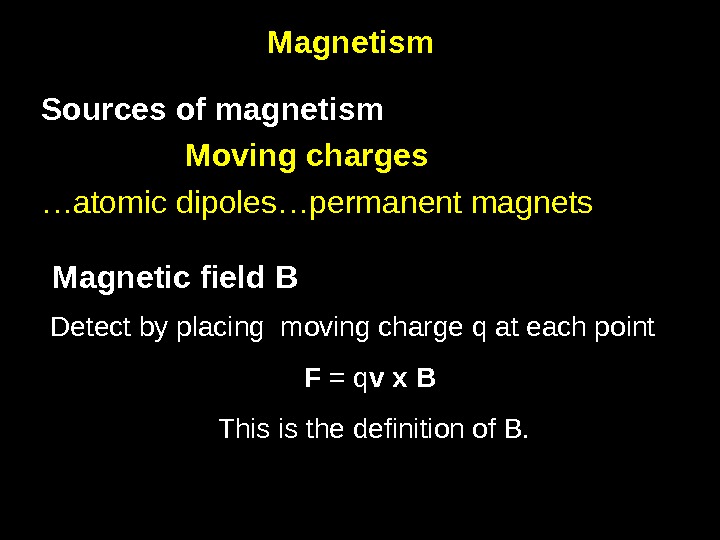
3 Magnetism Sources of magnetism Moving charges … atomic dipoles…permanent magnets Magnetic field B Detect by placing moving charge q at each point F = q v x B This is the definition of B.

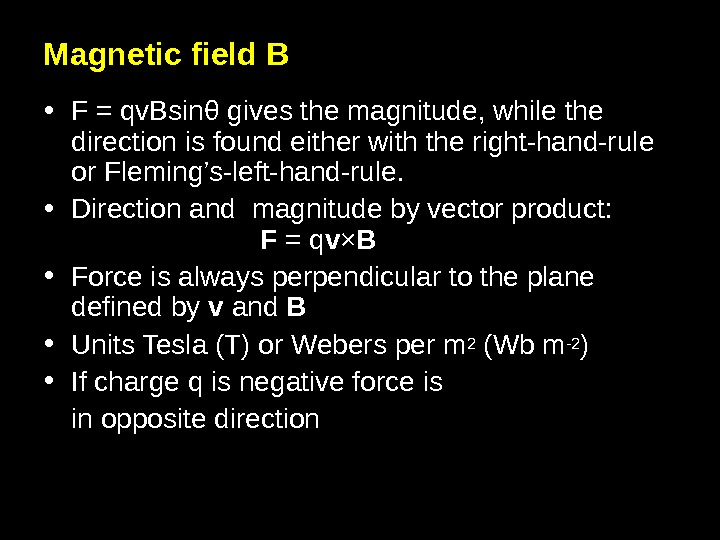
4 Magnetic field B • F = qv. Bsin θ gives the magnitude, while the direction is found either with the right-hand-rule or Fleming’s-left-hand-rule. • Direction and magnitude by vector product: F = q v × B • Force is always perpendicular to the plane defined by v and B • Units Tesla (T) or Webers per m 2 (Wb m-2 ) • If charge q is negative force is in opposite direction

F = q v × B (Force a + ve charge experiences )( q v, or moving charge ) ( B, or Mag. Field )

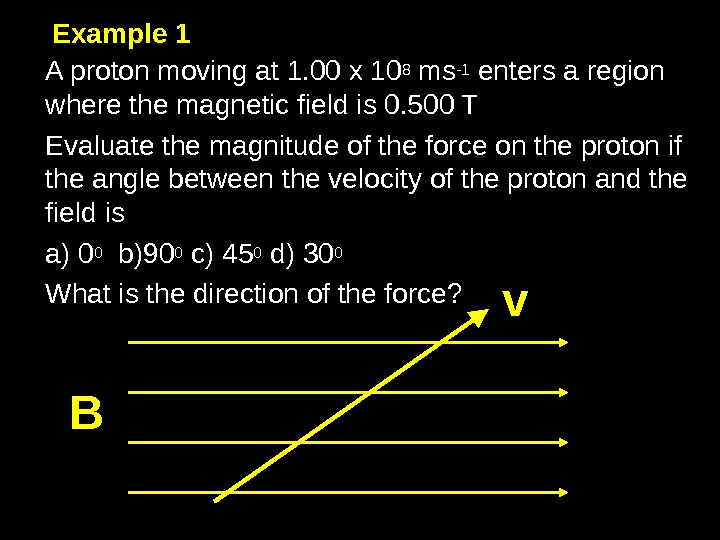
6 Example 1 A proton moving at 1. 00 x 108 ms-1 enters a region where the magnetic field is 0. 500 T Evaluate the magnitude of the force on the proton if the angle between the velocity of the proton and the field is a) 0 0 b)900 c) 450 d) 300 What is the direction of the force? B v

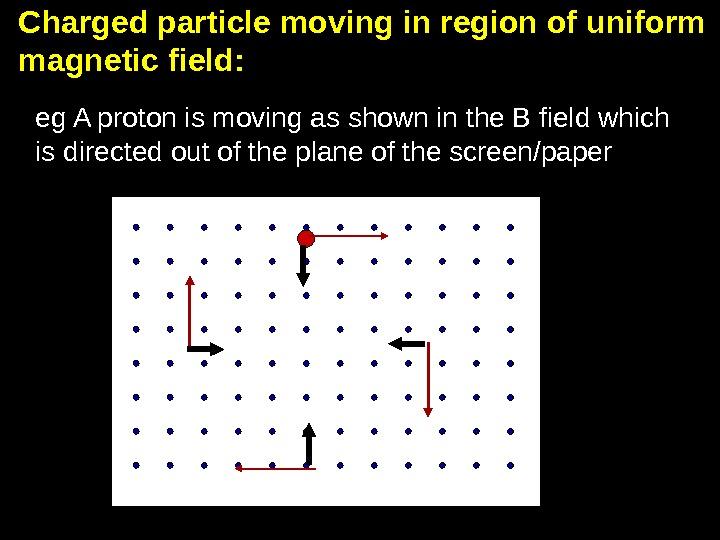
7 Charged particle moving in region of uniform magnetic field: eg A proton is moving as shown in the B field which is directed out of the plane of the screen/paper

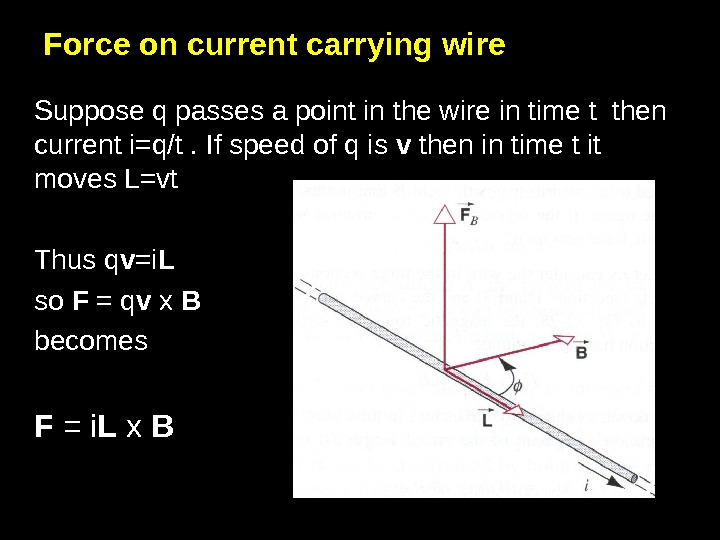
8 Force on current carrying wire Suppose q passes a point in the wire in time t then current i=q/t. If speed of q is v then in time t it moves L=vt Thus q v =i L so F = q v x B becomes F = i L x

9 Example 2 A straight 0. 400 m length of wire carries a current of 2. 00 A. It is placed in a B field of 0. 250 T as shown. The angle between the wire and the field is 300 What is the magnitude and direction of the force on the wire? B I

10 Visualising a magnetic field Magnetic “lines of force” • Flux : total number of lines passing through an area. • The flux density gives the magnitude of B field. This is the number of lines passing through unit area placed normally to the lines. Units: Wb m-2 equivalent to Tesla • Direction of the magnetic field is the direction of a tangent to a field line • Magnetic dipole aligns with field line

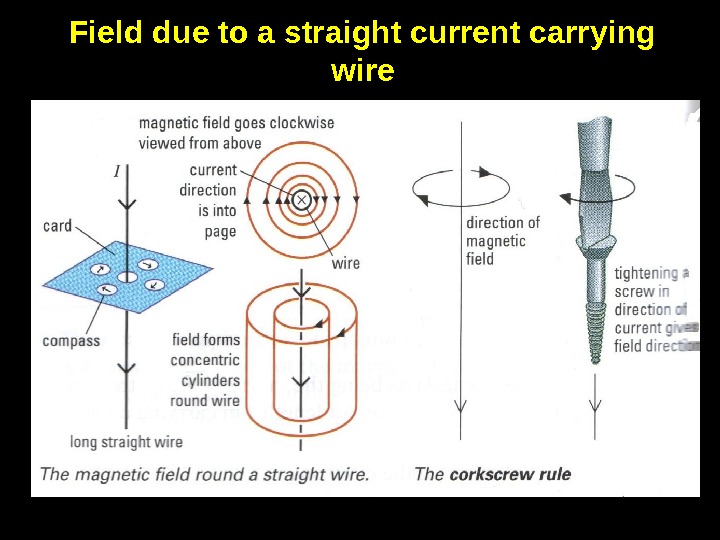
11 Field due to a straight current carrying wire

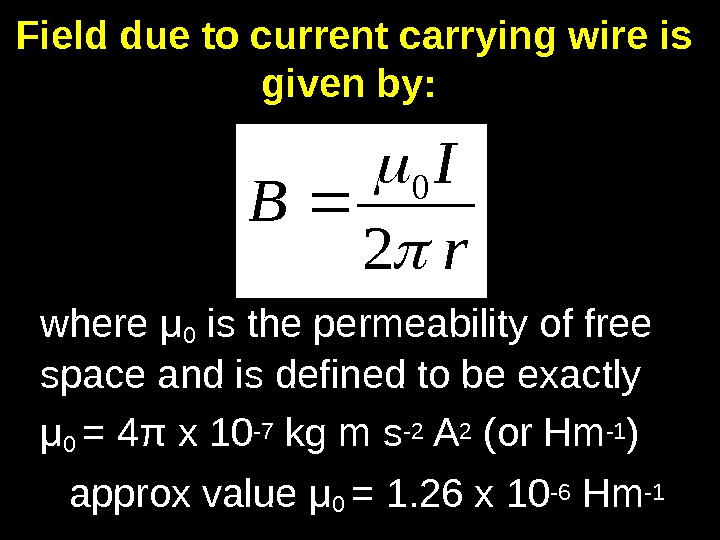
12 where μ 0 is the permeability of free space and is defined to be exactly μ 0 = 4 π x 10 -7 kg m s -2 A 2 (or Hm -1 ) approx value μ 0 = 1. 26 x 10 -6 Hm -1 Field due to current carrying wire is given by: r I

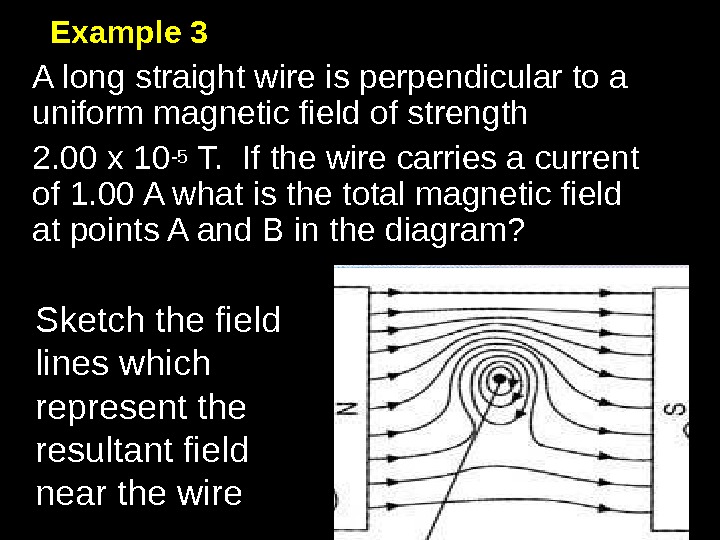
13 Example 3 A long straight wire is perpendicular to a uniform magnetic field of strength 2. 00 x 10 -5 T. If the wire carries a current of 1. 00 A what is the total magnetic field at points A and B in the diagram? Sketch the field lines which represent the resultant field near the wire

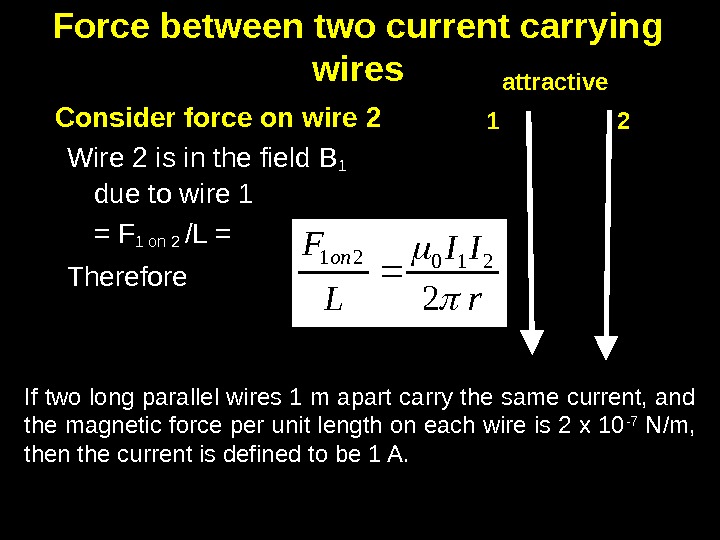
14 Force between two current carrying wires Wire 2 is in the field B 1 due to wire 1 = F 1 on 2 /L = Therefore 1 2 Consider force on wire 2 r II LF on 2 21021 attractive If two long parallel wires 1 m apart carry the same current, and the magnetic force per unit length on each wire is 2 x 10 -7 N/m, then the current is defined to be 1 A.

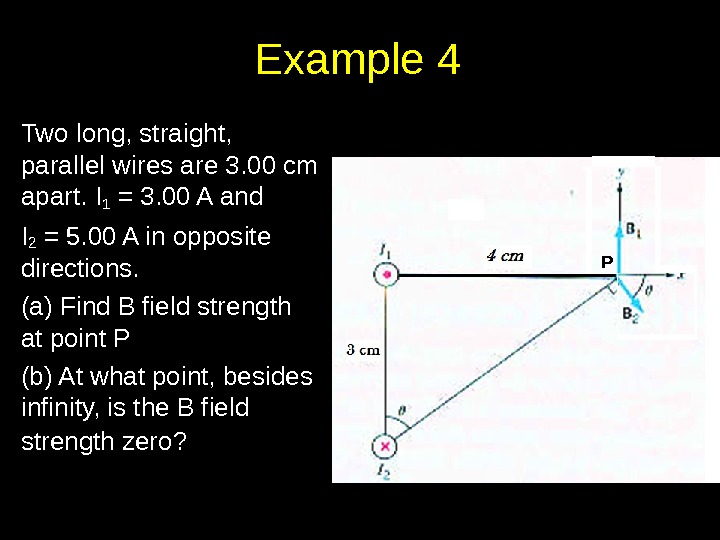
Example 4 Two long, straight, parallel wires are 3. 00 cm apart. I 1 = 3. 00 A and I 2 = 5. 00 A in opposite directions. (a) Find B field strength at point P (b) At what point, besides infinity, is the B field strength zero? P

16 LECTURE CHECK LIST LECTURE 31 Magnetic Fields READING Adams and Allday: 5. 14, 5. 15, 5. 16 Serway ch. 19. 1 -19. 3 At the end of this lecture you should: • Understand how a magnetic field is defined implicitly by the Lorentz force • Be able to calculate the magnitude and direction of the force on a conductor of length, l, carrying current, I, in a magnetic field of field strength, B • Know the formula for the field strength due to a long straight wire carrying current, I, at a distance, r, from the wire • Understand how the definition of the ampere arises and be able to give the definition • Understand the concept of field lines for a magnetic field

17 Fields due to coil and solenoid

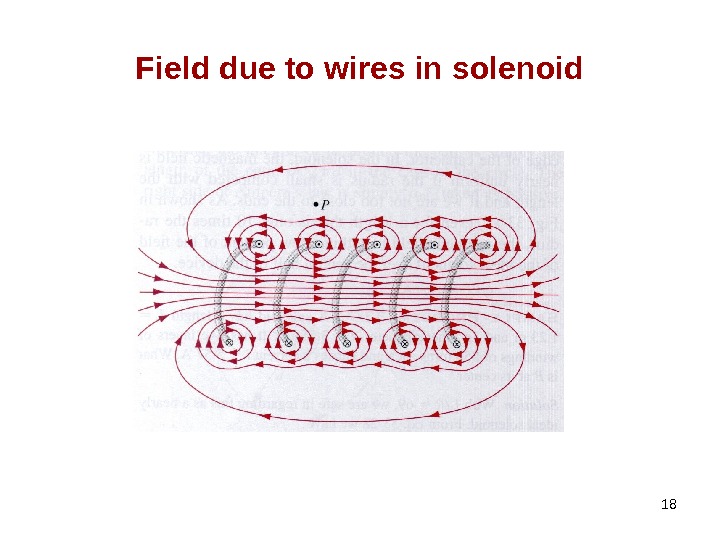
18 Field due to wires in solenoid

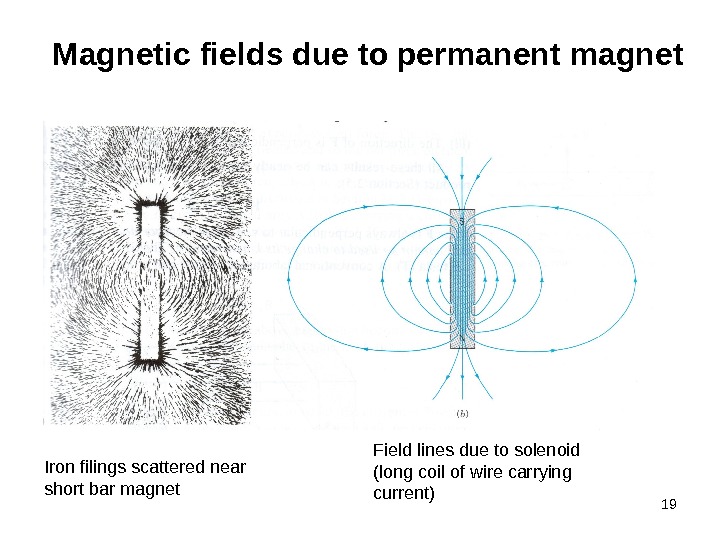
19 Magnetic fields due to permanent magnet Iron filings scattered near short bar magnet Field lines due to solenoid (long coil of wire carrying current)

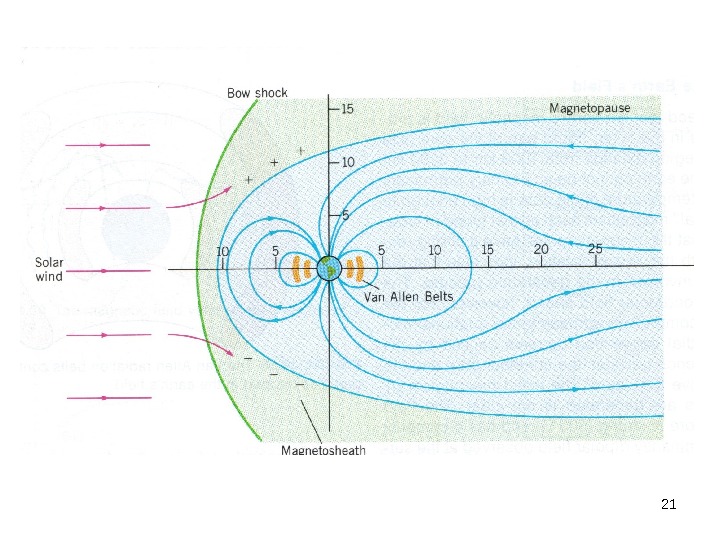
20 Earth’s magnetic field

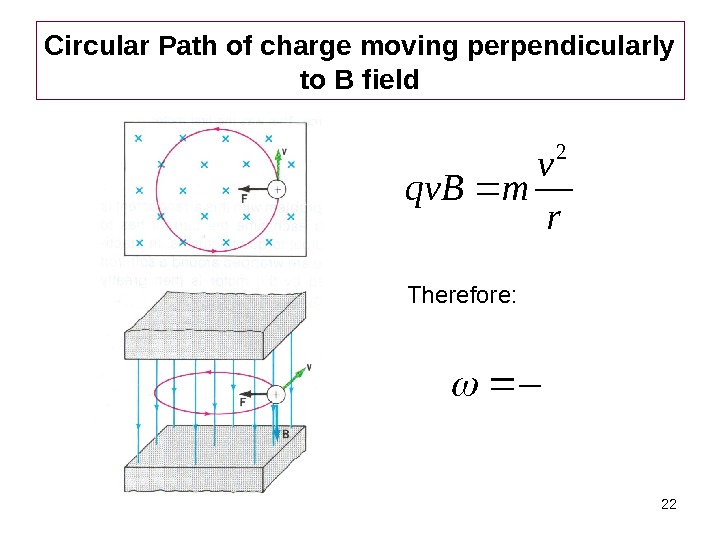
22 Circular Path of charge moving perpendicularly to B field rv mqv. B 2 Therefore: rv mqv.

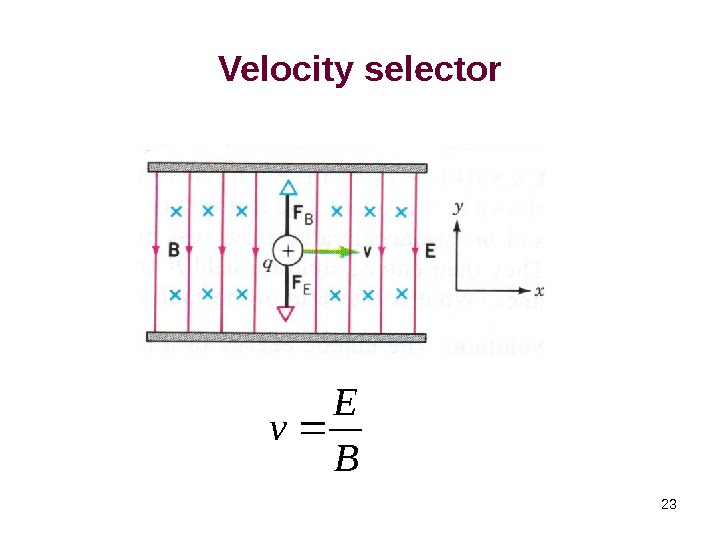
23 Velocity selector. B E v

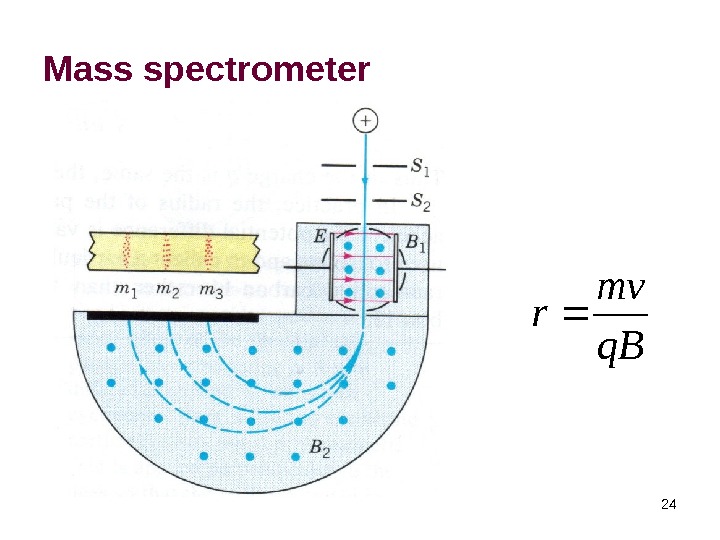
24 Mass spectrometerq. B mv r

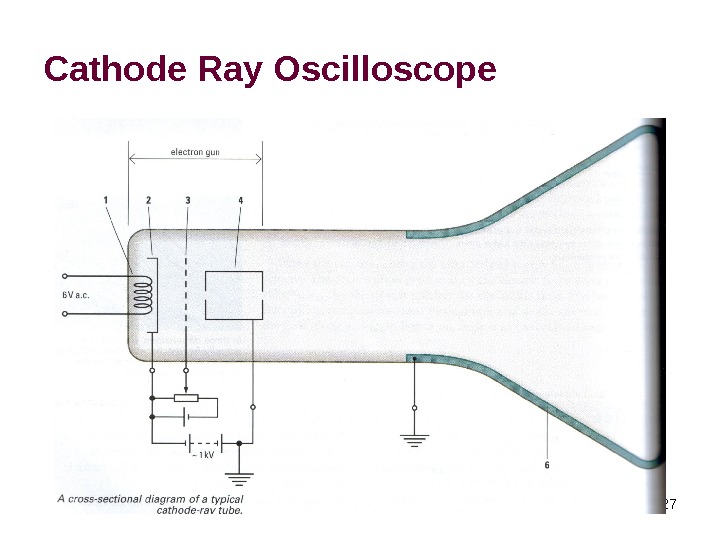
25 Cathode Ray Oscilloscope

27 Cathode Ray Oscilloscope

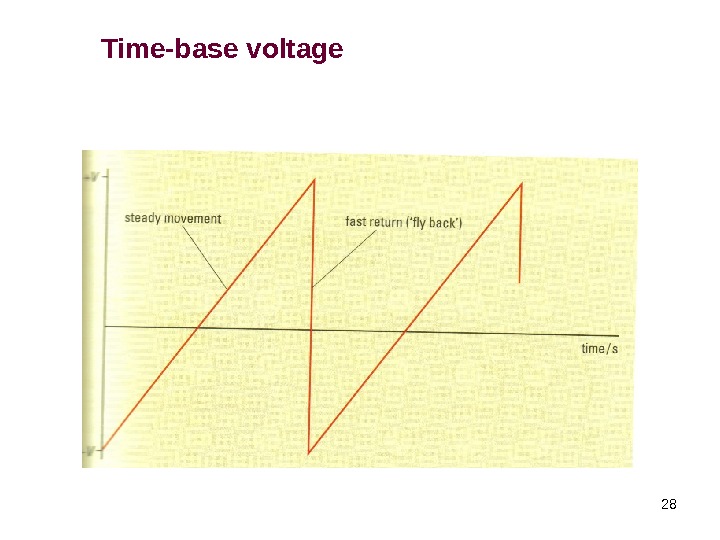
28 Time-base voltage

29 LECTURE CHECK LIST LECTURE 27 Magnetic Fields READING Adams and Allday: 5. 14, 5. 15, 5. 16 At the end of this lecture you should: • Understand how a magnetic field is defined implicitly by the Lorentz force • Be able to calculate the magnitude and direction of the force on a conductor of length, l, carrying current, I, in a magnetic field of field strength, B • Know the formula for the field strength due to a long straight wire carrying current, I, at a distance, a, from the wire • Understand how the definition of the ampere arises and be able to give the definition • Understand the concept of field lines for a magnetic field • Know the formula for the magnetic field strength due to a solenoid and be able to perform calculations using that formula • Have an understanding of the form of the Earth’s magnetic field and understand that this field resolves into two components

30 LECTURE CHECK LIST LECTURE 28 Applications of Electric and Magnetic Fields READING Adams and Allday: 5. 19, 5. 20 8. 3, 10. 16 At the end of this lecture you should: • Understand the principles behind the mass spectrometer and be able to perform calculations which demonstrate that understanding • Understand the workings of the Cathode Ray Oscilloscope (CRO) • Appreciate that a television works on the same principles as a CRO