1 -5 Roots and Irrational Numbers Preview Warm

1 -5 Roots and Irrational Numbers Preview Warm Up California Standards Lesson Presentation

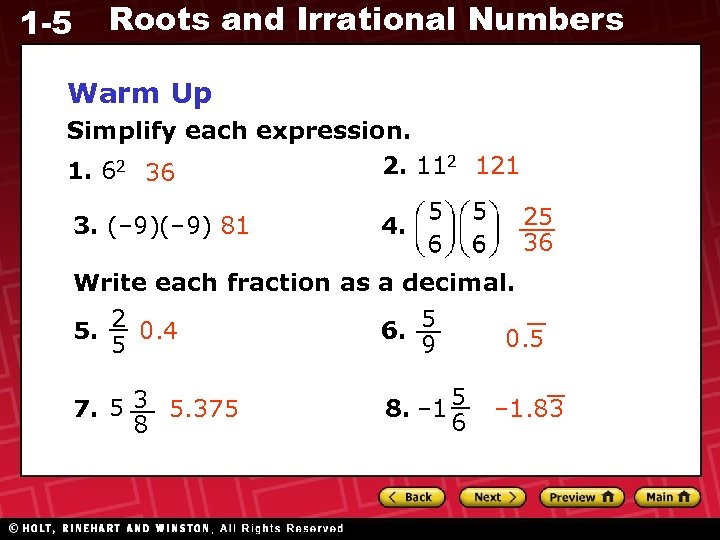
1 -5 Roots and Irrational Numbers Warm Up Simplify each expression. 2. 112 121 1. 62 36 3. (– 9) 81 25 36 4. Write each fraction as a decimal. 5. 2 0. 4 6. 5 0. 5 5 9 7. 5 3 5. 375 8 8. – 1 5 6 – 1. 83

1 -5 Roots and Irrational Numbers California Standards 2. 0 Students understand use such operations as taking the opposite, finding the reciprocal, taking a root, and raising to a fractional power. They understand use the rules of exponents.

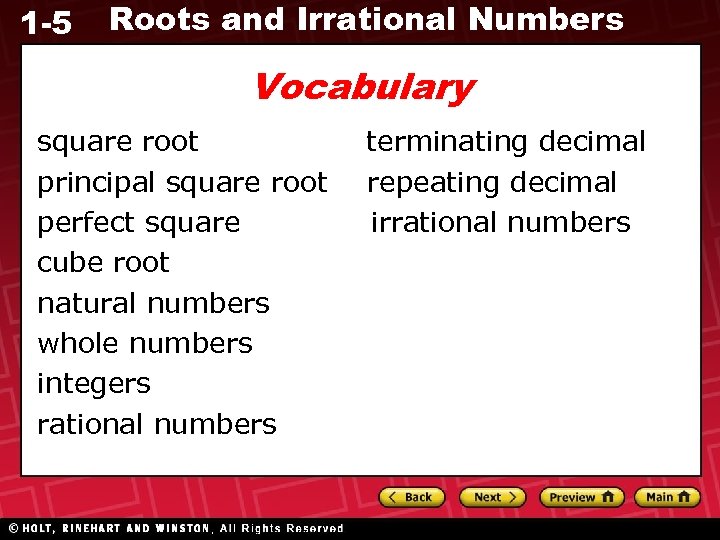
1 -5 Roots and Irrational Numbers Vocabulary square root principal square root perfect square cube root natural numbers whole numbers integers rational numbers terminating decimal repeating decimal irrational numbers

1 -5 Roots and Irrational Numbers A number that is multiplied by itself to form a product is a square root of that product. The radical symbol is used to represent square roots. For nonnegative numbers, the operations of squaring and finding a square root are inverse operations. In other words, for x ≥ 0, Positive real numbers have two square roots. =4 4 4 = 42 = 16 (– 4) = (– 4)2 = 16 – Positive square root of 16 = – 4 Negative square root of 16

Roots and Irrational Numbers 1 -5 The principal square root of a number is the positive square root and is represented by. A negative square root is represented by –. The symbol is used to represent both square roots. A perfect square is a number whose positive square root is a whole number. Some examples of perfect squares are shown in the table. 0 1 4 9 02 12 22 32 16 25 36 49 64 81 100 42 52 62 72 82 92 102

1 -5 Roots and Irrational Numbers Writing Math The small number to the left of the root is the index. In a square root, the index is understood to be 2. In other words, is the same as.

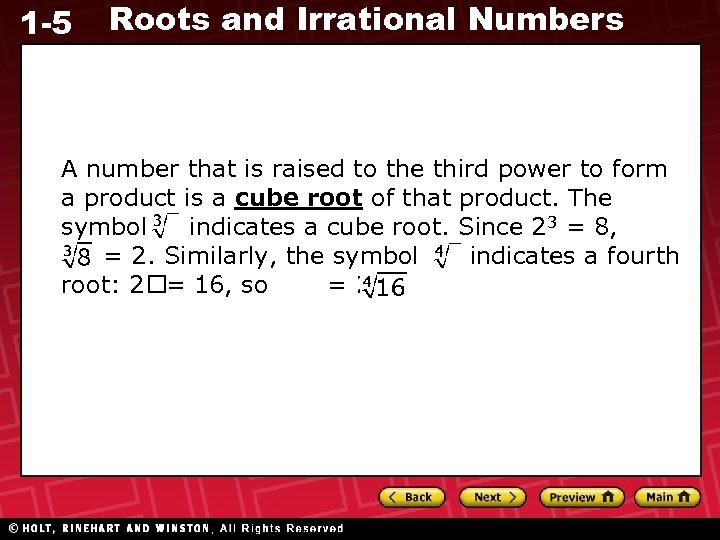
1 -5 Roots and Irrational Numbers A number that is raised to the third power to form a product is a cube root of that product. The symbol indicates a cube root. Since 23 = 8, = 2. Similarly, the symbol indicates a fourth root: 2 = 16, so = 2.

1 -5 Roots and Irrational Numbers Additional Example 1: Finding Roots Find each root. Think: What number squared equals 81? Think: What number squared equals 25?

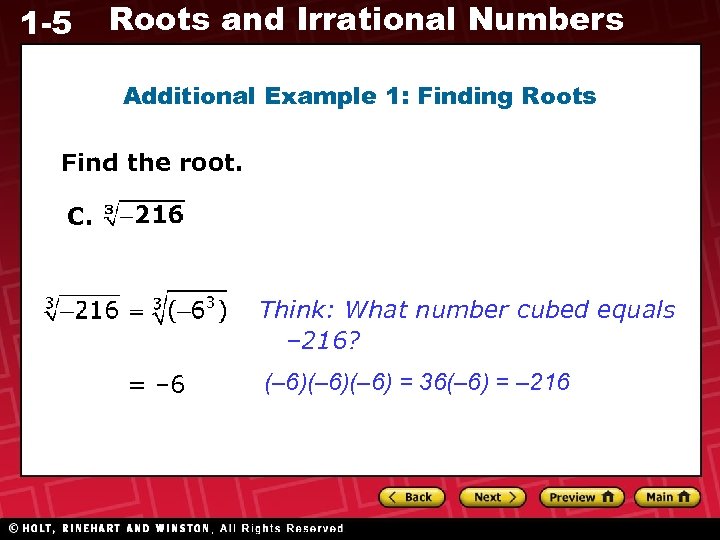
1 -5 Roots and Irrational Numbers Additional Example 1: Finding Roots Find the root. C. Think: What number cubed equals – 216? = – 6 (– 6)(– 6) = 36(– 6) = – 216

1 -5 Roots and Irrational Numbers Check It Out! Example 1 Find each root. a. Think: What number squared equals 4? b. Think: What number squared equals 25?

1 -5 Roots and Irrational Numbers Check It Out! Example 1 Find the root. c. Think: What number to the fourth power equals 81?

Roots and Irrational Numbers 1 -5 Additional Example 2: Finding Roots of Fractions Find the root. A. Think: What number squared equals

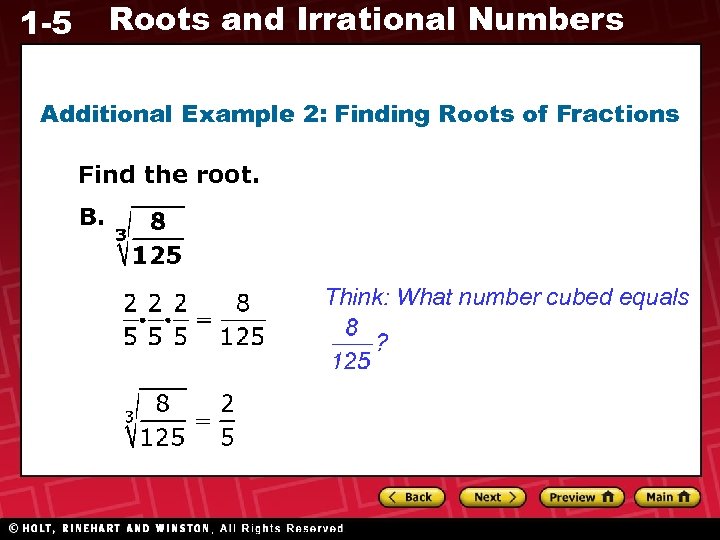
Roots and Irrational Numbers 1 -5 Additional Example 2: Finding Roots of Fractions Find the root. B. Think: What number cubed equals

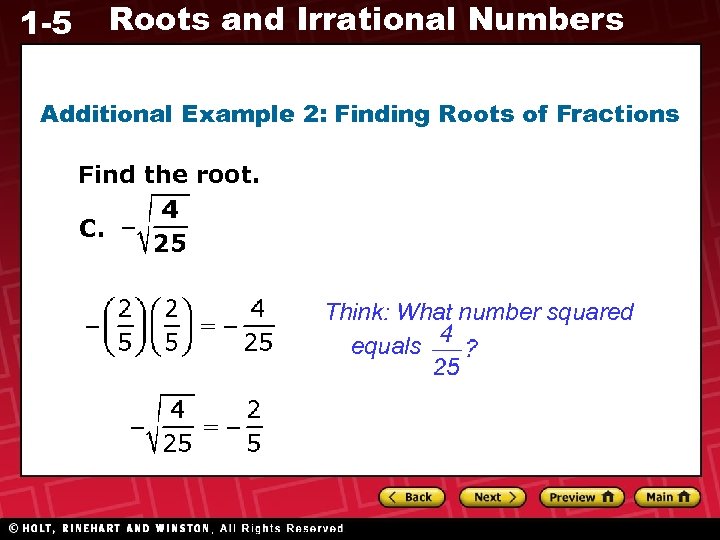
Roots and Irrational Numbers 1 -5 Additional Example 2: Finding Roots of Fractions Find the root. C. Think: What number squared equals

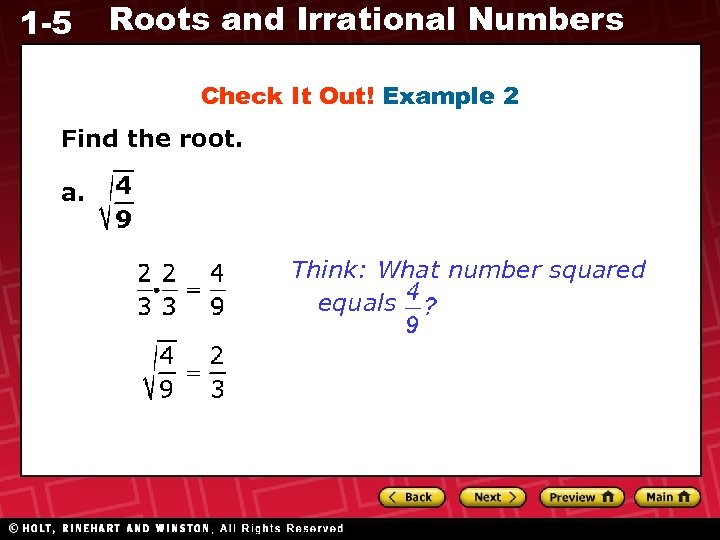
1 -5 Roots and Irrational Numbers Check It Out! Example 2 Find the root. a. Think: What number squared equals

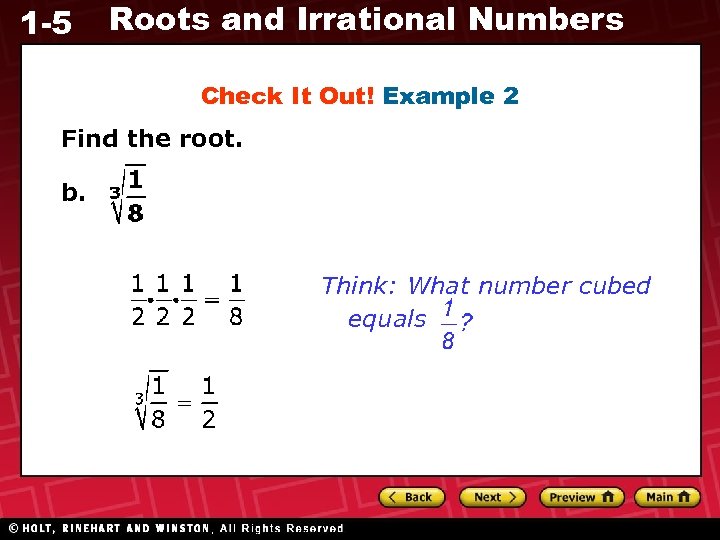
1 -5 Roots and Irrational Numbers Check It Out! Example 2 Find the root. b. Think: What number cubed equals

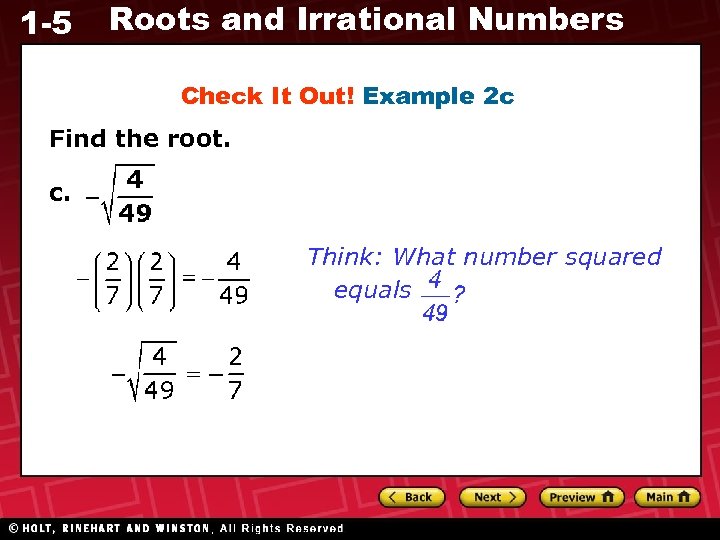
1 -5 Roots and Irrational Numbers Check It Out! Example 2 c Find the root. c. Think: What number squared equals

1 -5 Roots and Irrational Numbers Square roots of numbers that are not perfect squares, such as 15, are not whole numbers. A calculator can approximate the value of as 3. 872983346. . . Without a calculator, you can use square roots of perfect squares to help estimate the square roots of other numbers.

1 -5 Roots and Irrational Numbers Additional Example 3: Art Application As part of her art project, Shonda will need to make a paper square covered in glitter. Her tube of glitter covers 13 in². Estimate to the nearest tenth the side length of a square with an area of 13 in². Since the area of the square is 13 in², then each side of the square is in. 13 is not a perfect square, so find two consecutive perfect squares that is between: 9 and 16. is between and , or 3 and 4. Refine the estimate.

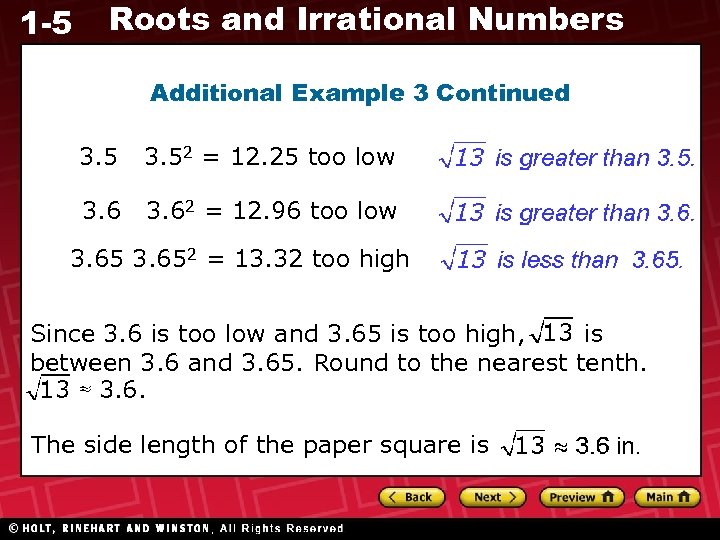
1 -5 Roots and Irrational Numbers Additional Example 3 Continued 3. 52 = 12. 25 too low 3. 62 = 12. 96 too low 3. 652 = 13. 32 too high Since 3. 6 is too low and 3. 65 is too high, is between 3. 6 and 3. 65. Round to the nearest tenth. The side length of the paper square is

1 -5 Roots and Irrational Numbers Writing Math The symbol ≈ means “is approximately equal to. ”

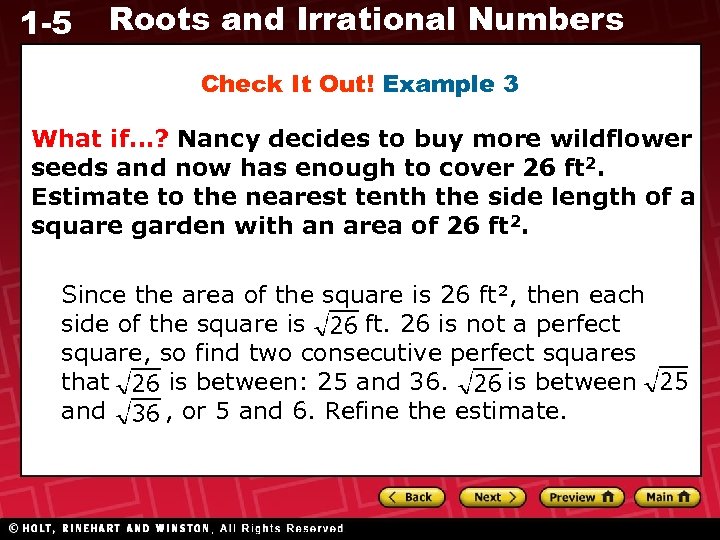
1 -5 Roots and Irrational Numbers Check It Out! Example 3 What if…? Nancy decides to buy more wildflower seeds and now has enough to cover 26 ft 2. Estimate to the nearest tenth the side length of a square garden with an area of 26 ft 2. Since the area of the square is 26 ft², then each side of the square is ft. 26 is not a perfect square, so find two consecutive perfect squares that is between: 25 and 36. is between and , or 5 and 6. Refine the estimate.

1 -5 Roots and Irrational Numbers Check It Out! Example 3 Continued 5. 02 = 25 too low 5. 12 = 26. 01 too high Since 5. 0 is too low and 5. 1 is too high, is between 5. 0 and 5. 1. Rounded to the nearest tenth, 5. 1. The side length of the square garden is 5. 1 ft.

1 -5 Roots and Irrational Numbers Real numbers can be classified according to their characteristics. Natural numbers are the counting numbers: 1, 2, 3, … Whole numbers are the natural numbers and zero: 0, 1, 2, 3, … Integers are the whole numbers and their opposites: – 3, – 2, – 1, 0, 1, 2, 3, …

1 -5 Roots and Irrational Numbers Rational numbers are numbers that can be expressed in the form , where a and b are both integers and b ≠ 0. When expressed as a decimal, a rational number is either a terminating decimal or a repeating decimal. • A terminating decimal has a finite number of digits after the decimal point (for example, 1. 25, 2. 75, and 4. 0). • A repeating decimal has a block of one or more digits after the decimal point that repeat continuously (where all digits are not zeros).

1 -5 Roots and Irrational Numbers Irrational numbers are all numbers that are not rational. They cannot be expressed in the form where a and b are both integers and b ≠ 0. They are neither terminating decimals nor repeating decimals. For example: 0. 1010010000100000… After the decimal point, this number contains 1 followed by one 0, and then 1 followed by two 0’s, and then 1 followed by three 0’s, and so on. This decimal neither terminates nor repeats, so it is an irrational number.

1 -5 Roots and Irrational Numbers If a whole number is not a perfect square, then its square root is irrational. For example, 2 is not a perfect square and is irrational.

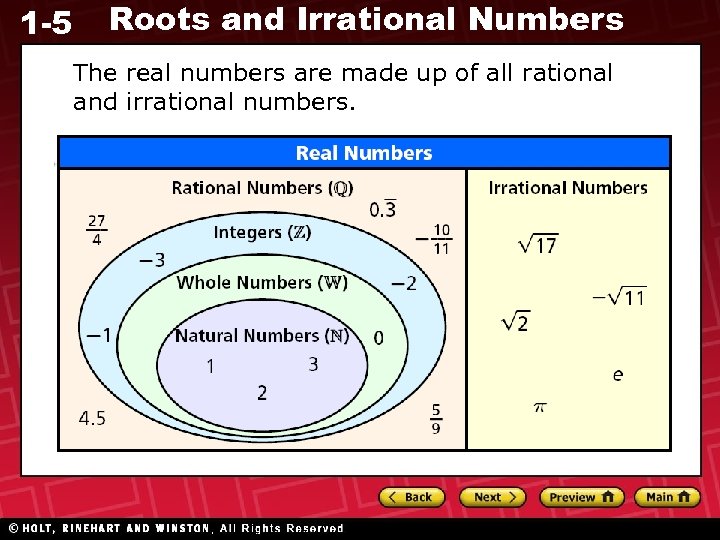
1 -5 Roots and Irrational Numbers The real numbers are made up of all rational and irrational numbers.

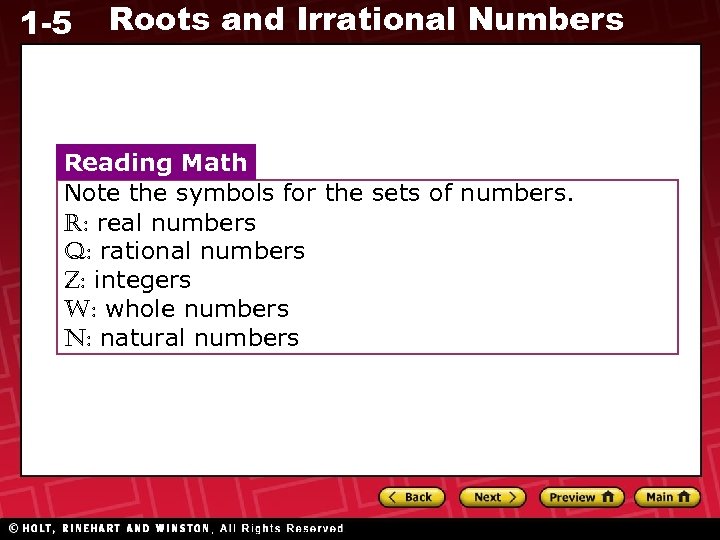
1 -5 Roots and Irrational Numbers Reading Math Note the symbols for the sets of numbers. R: real numbers Q: rational numbers Z: integers W: whole numbers N: natural numbers

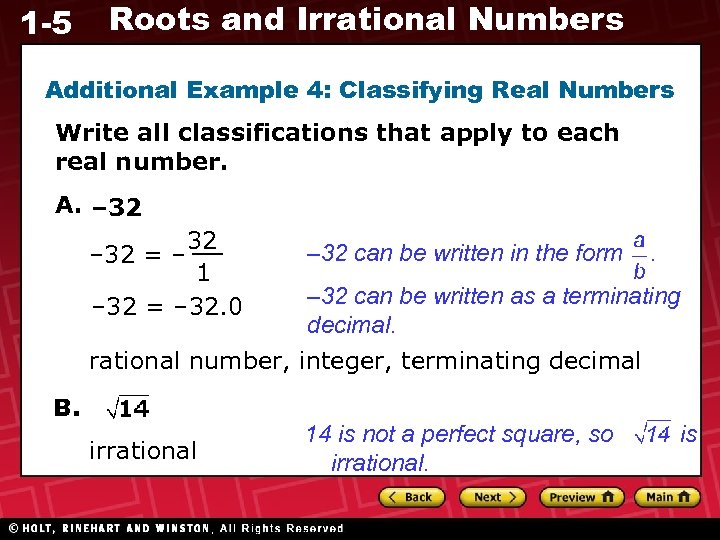
1 -5 Roots and Irrational Numbers Additional Example 4: Classifying Real Numbers Write all classifications that apply to each real number. A. – 32 32 1 – 32 = – 32. 0 – 32 = – – 32 can be written in the form . – 32 can be written as a terminating decimal. rational number, integer, terminating decimal B. irrational 14 is not a perfect square, so irrational. is

1 -5 Roots and Irrational Numbers Check It Out! Example 4 Write all classifications that apply to each real number. a. 7 7 49 can be written in the form . can be written as a repeating decimal. rational number, repeating decimal b. – 12 can be written in the form. – 12 can be written as a terminating decimal. rational number, terminating decimal, integer 67 9 = 7. 444… = 7. 4

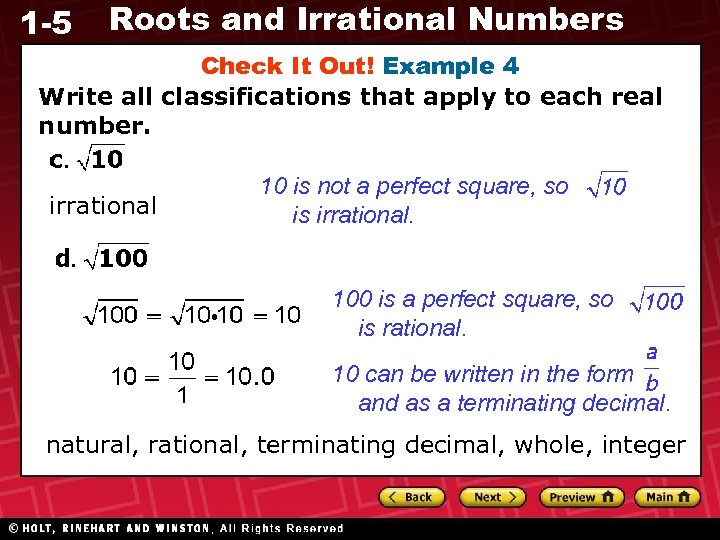
1 -5 Roots and Irrational Numbers Check It Out! Example 4 Write all classifications that apply to each real number. irrational 10 is not a perfect square, so is irrational. 100 is a perfect square, so is rational. 10 can be written in the form and as a terminating decimal. natural, rational, terminating decimal, whole, integer

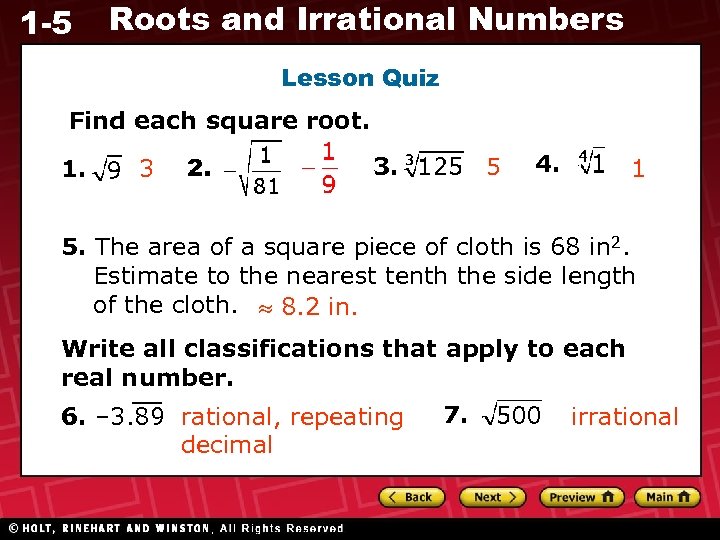
1 -5 Roots and Irrational Numbers Lesson Quiz Find each square root. 1. 3 2. 3. 5 4. 1 5. The area of a square piece of cloth is 68 in 2. Estimate to the nearest tenth the side length of the cloth. 8. 2 in. Write all classifications that apply to each real number. 6. – 3. 89 rational, repeating decimal 7. irrational