Reduction Method of Integration Used to find where

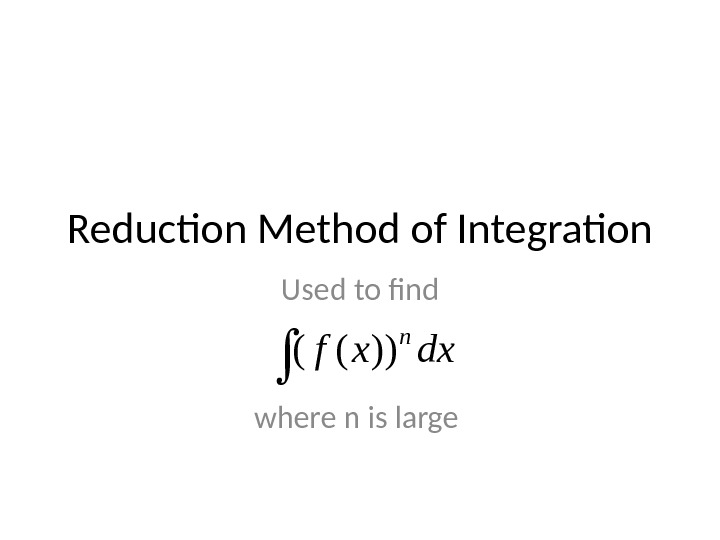Reduction Method of Integration Used to find where n is large dxxf n ))((

Establish a reduction formula for • Let • Use by parts: • So, xdx ncos xdxxxdx. I nn n 11 coscoscos xux dxdu xxn dxdv xv nn sincos)1(cos 21 xdxxxnxx. I nn nsinsincos)1(cossin

Establish a reduction formula for xdx ncos nnn nn nn n In. Inxx xdxnxx dxxxnxx xdxxnxx. I )1()1(cossin cos)1(cossin )cos 1(cos)1(cossin sincos)1(cossin

Establish a reduction formula for xdx ncos 21 21 21 )1( cossin 1 , )1(cossin)1(, nn nn I nn xx n. IHence Inxxn. I Inxx. In. ISo

Use a reduction formula to find xdx 5 cos 34 5 2515 5 21 5 4 cossin 5 1 5 )15( cossin 5 1 , )1( cossin 1 , Ixx. I So I n n xx n I Now nn n

Use a reduction formula to find xdx 5 cos Cxxxxx. I xdxxxxx. I Ixx. I sin 3 2 cossin 3 1 5 4 cossin 5 1 cos 3 2 cossin 3 1 5 4 cossin

Establish a reduction formula for • The same method can be applied to: • Hence, xdx n sin xdxxxdx. Inn n 11 sinsinsin xx n I n nn 1 2 sincos 1)1(

Establish a reduction formula for • Let • Using xdx ntan xdxxxdx. Inn n 22 tantantan 1 sectan 22 xx 21 222 22 tan 1 1 tantansec tan)1(sec nn nn n n Ix n xdxxdxx. I x n xdxx Since nn 12 tan 1 1 tansec

Establish a reduction formula for • Let • Use by parts: • So, dxex xn dxex. I xn n xx nn eue dxdu nx dxdv xv 1 dxexnex. I dxenxex. I xnxn n

Establish a reduction formula for • Hence, dxex xn 1 n xn n n. Iex. I

Using a reduction formula find • Using: dxex x 4 1 n xn n n. Iex. I dxexxeexexex. I Iexexex. I Iex. I xxxxx xxx xx x

Using a reduction formula find • Hence, dxex x 4 Cexeexexex. I xxxxx